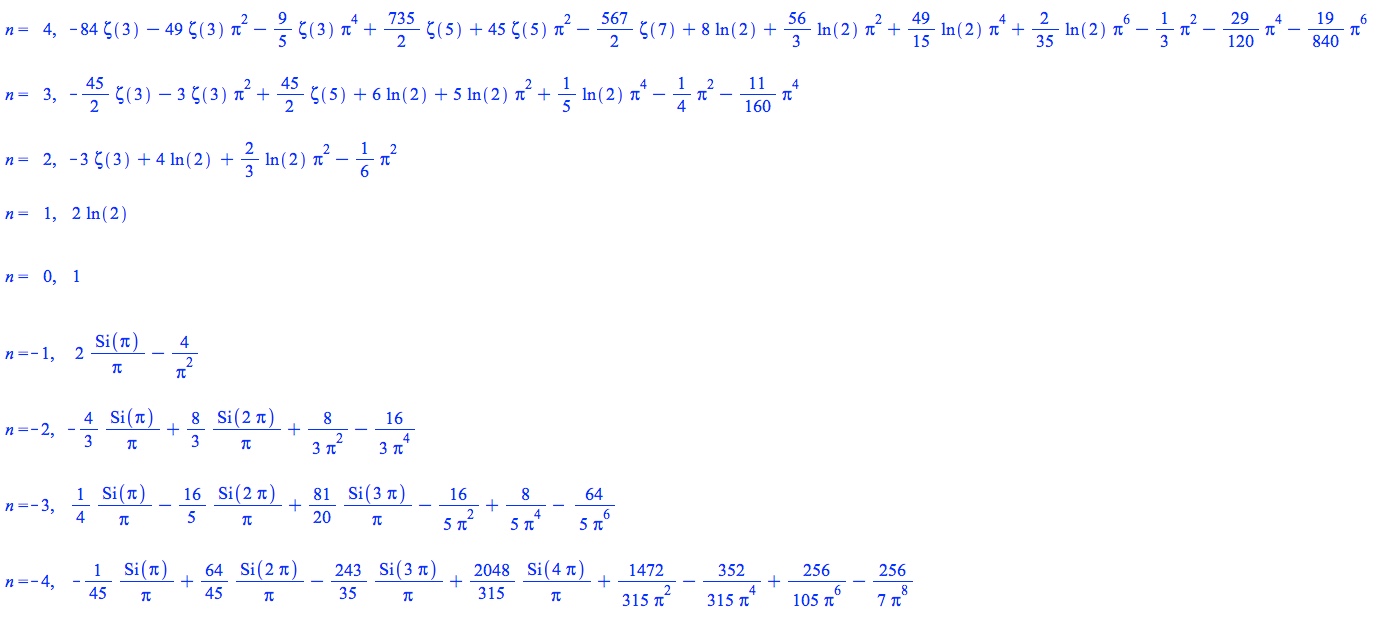Another integral that has a closed form involving finite series of $\zeta(2k+1)$'s. Could it be reflexive?

Question

In the context of a series of questions here, here and here, about closed form expressions involving finite series of $\zeta(2k+1)$'s for certain integrals, I would like to raise another one:

$$f(n):=\int\limits_0^1\bigg(\frac{\pi x}{2}\,\csc\left(\frac{\pi x}{2}\right)\bigg)^{2n}dx$$

Various CAS-tools induce closed forms at integer values. Below are the ones for $n=4 \dots-4$:

where $Si(x)$= the Sine integral.

I managed to find (numerically, not a direct derivation) generic formulae for all rational coefficients in these closed forms for both $n\ge1$ and $n\le 1$. They are listed in the greyed area below.

Question:

Could this integral be reflexive, i.e. could or does there exist a functional relation between for instance $f(n)$ and $f(-n)$ (and thereby potentially relating the $\zeta(2k+1)$'s to the $Si(k)$'s)?

$\\$ $\\$ $\\$

$\rightarrow$ for $n=1,2,3,...$:

\begin{align} \small f(n) &=\small 2n\cdot\sum_{q=1}^{n-1}\sum_{r=1}^{q}(-1)^r\frac{CFN(n,q)}{\Gamma(2\,(q-r+1))}\frac{2^{2r}-1}{2^{2r}}\,{\color{blue}{\zeta(2r+1)\,\pi^{2(q-r)}}}\\ &+\small2n\cdot \sum_{q=0}^{n-1}\frac{CFN(n,q)}{\Gamma(2\,(q+1))}\,{\color{blue}{\ln(2)\,\pi^{2q}}}\\ &+2n\cdot\frac{ETA1(n-1)}{\Gamma(2n)}\,{\color{blue}{\pi^{2(n-1)}}}\\ &+2n\cdot\small\sum_{q=1}^{n-2}(-1)^{n-q}\,\frac{ETA(n,n-q)}{\Gamma(2\,(q+1))}\,{\color{blue}{\pi^{2q}}}\\ \end{align}

$\displaystyle \scriptsize CFN(n,k) = \sum_{m=-k}^{k}(-1)^m\,s(n,n+m-k)\,s(n,n-m-k)$

This is the Central Factorial Number triangle (A008955) with $s(p,q)=$ first kind Stirling number.

$\displaystyle \scriptsize ETA1(n) \,\, = -\sum_{k=1}^{n}\left(\frac{n!}{k!}\right)^2\,\frac{\Gamma(2k)}{2^{2k}}$

$\displaystyle \scriptsize ETA(n,k) =(-1)^{k}\,\sum_{m=1}^{n-k}coeff\left(\prod_{p=m+2}^{n-1}(1+p^2x),x,n-k-m\right)\,ETA1(m) \quad k>1,n>k$

with coeff$(gf,x,k)=$ the k-th coefficient of generating function $gf(x)$. $ETA(n,k)$ is directly related to the Eta-triangle A160464, e.g.$2^{2+\log_{2}(n)}\,ETA1[n]=$A160465.

$\\$ $\\$

$\rightarrow$ for $n=-1,-2,-3,...$: \begin{align} \small f(-n) &=\small 4n \sum_{q=1}^{n}(-1)^{q+n}\,\frac{q^{2n-1}}{(n-q)!\,(n+q)!}\,{\color{blue}{\frac{Si(q\,\pi)}{\pi}}}\\ &-\frac{2^{2n}}{(2n-1)}\,{\color{blue}{\frac{1}{\pi^{2n}}}}\\ &+\small \frac{n}{\Gamma(2n)}\sum_{q=1}^{n-1}\,(-1)^{q+1}\,2^{2n-q}\,\Gamma(2n-2q-1)\left(\sum_{r=0}^{q}(-1)^{r+q+1}ETT(q,r+1)\,n^r\right){\color{blue}{\frac{1}{\pi^{2(n-q)}}}}\\ \end{align}

$\displaystyle \scriptsize ETT(n,m) = \frac{1}{2^{n-m}}\,\sum_{k=1}^{n}\frac{s(k,m)\,\displaystyle \sum_{i=0}^{k-1}\,(-1)^{n+m+i}\,(i-k)^{2n}\,\binom{2k}{i}}{2^{k-1}\,k!}$ which is A083061.

Answer 1

As already said in my comment, there is no link between $f(n)$ for $n<0$ and the odd zeta values occurring for $n>0$.

For $n<0$, putting $k:=-n>0$, we have $f(-k)=\dfrac2\pi\int\limits_0^{\frac\pi2}\dfrac{\sin^{2k} z}{z^{2k} }dz$. After one integration by parts, we remain with some negative power of $\pi$ and an integral $\int \limits_0^{\frac\pi2}\dfrac{\sin^{2k-1} z\cos z}{z^{2k-1} }dz.$
Performing $2k-2$ more integrations by parts, all the remaining integrals are a combination $$\sum_{j=1}^k a_j\int\limits_0^{\frac\pi2} \dfrac{\sin^{2j-1} z\cos z}{z }dz.$$ By the multiple angle formulae, we can write the numerators as linear combinations of $\sin 2rz$ with $r=1,...,j$, thus evaluating the integrals as multiples of $Si(\pi),...,Si(k\pi)$.

As an example, $f(-1)=\frac2\pi\int_0^{\frac\pi2}\frac{\sin^2 z}{z^2}dz=\frac2\pi\left[\frac{2 z Si(2 z) + \cos(2 z) - 1}{2z}\right]_{z=0}^{z=\frac\pi2}=\frac2\pi(Si(\pi)-\frac2\pi)$.