as the title suggests I'm looking for some clarifications in the computations of the ext charts of some $A(1)$-modules arising as extensions of other modules. In particular, I've the following example I'd like to consider: $$0 \to \Sigma^3D \to \Bbb R P^{\infty} \to M \to 0$$
where $D$ is the $A(1)$-module described in Freed and Hopkins' paper at page 75 (table on the right), $M$ is the Moore space (whose $A(1)$-resolution can be found here, and $\Bbb R P^{\infty}$ is the infinite projective space. Here I draw the s.e.s with the usual convention for the action of $Sq^1$ and $Sq^2$: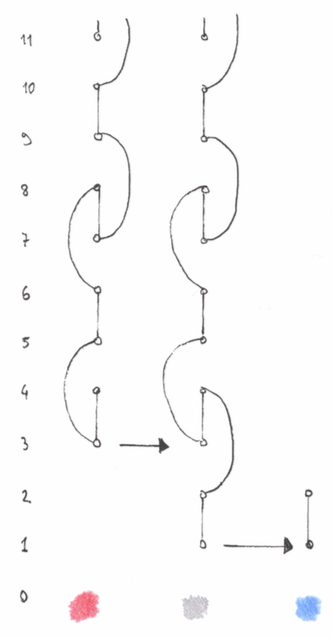
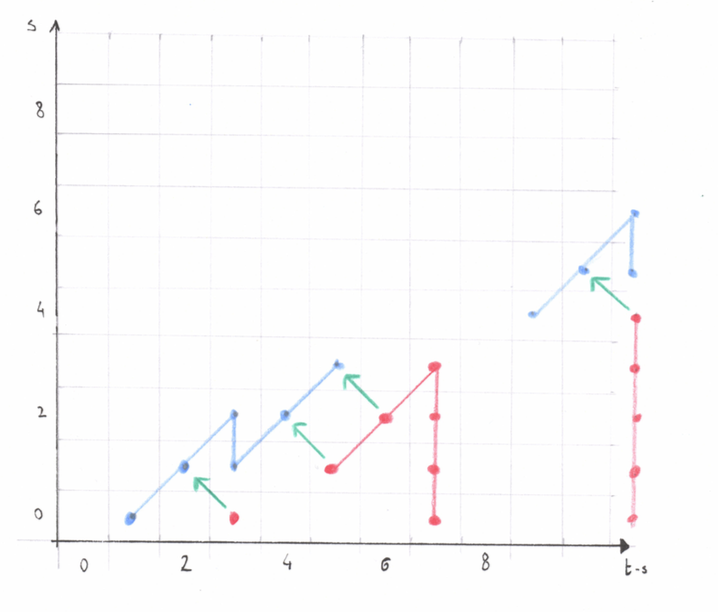
And here you can find what (according to me) is the situation at the level of ASS:
The green arrows should be the map representing the connecting homomorphisms in the l.e.s. of ext-groups induced by the above s.e.s.
Since we know what should be the stable page of the ASS for $\Bbb R P^{\infty}$, and there are no differentials for dimensions reason, we see that the only non-trivial green arrows are the one starting in position $(5,1)$ and $(6,2)$.
This is what I don't understand: If the arrows represent the maps induced by the l.e.s. of the ext's then at least the first one $$\delta \colon \hom_{A(1)}(\Sigma^3D, \Bbb F_2) \to Ext^1_{A(1)}(M, \Bbb F_2)$$ shouldn't be trivial, since the s.e.s. above is non-split. In particular this would be the green arrow starting from position $(1,0)$, which we observed that has to be zero.
So what am I missing?
I apologise in advance if the question is stupid.