7
$\begingroup$

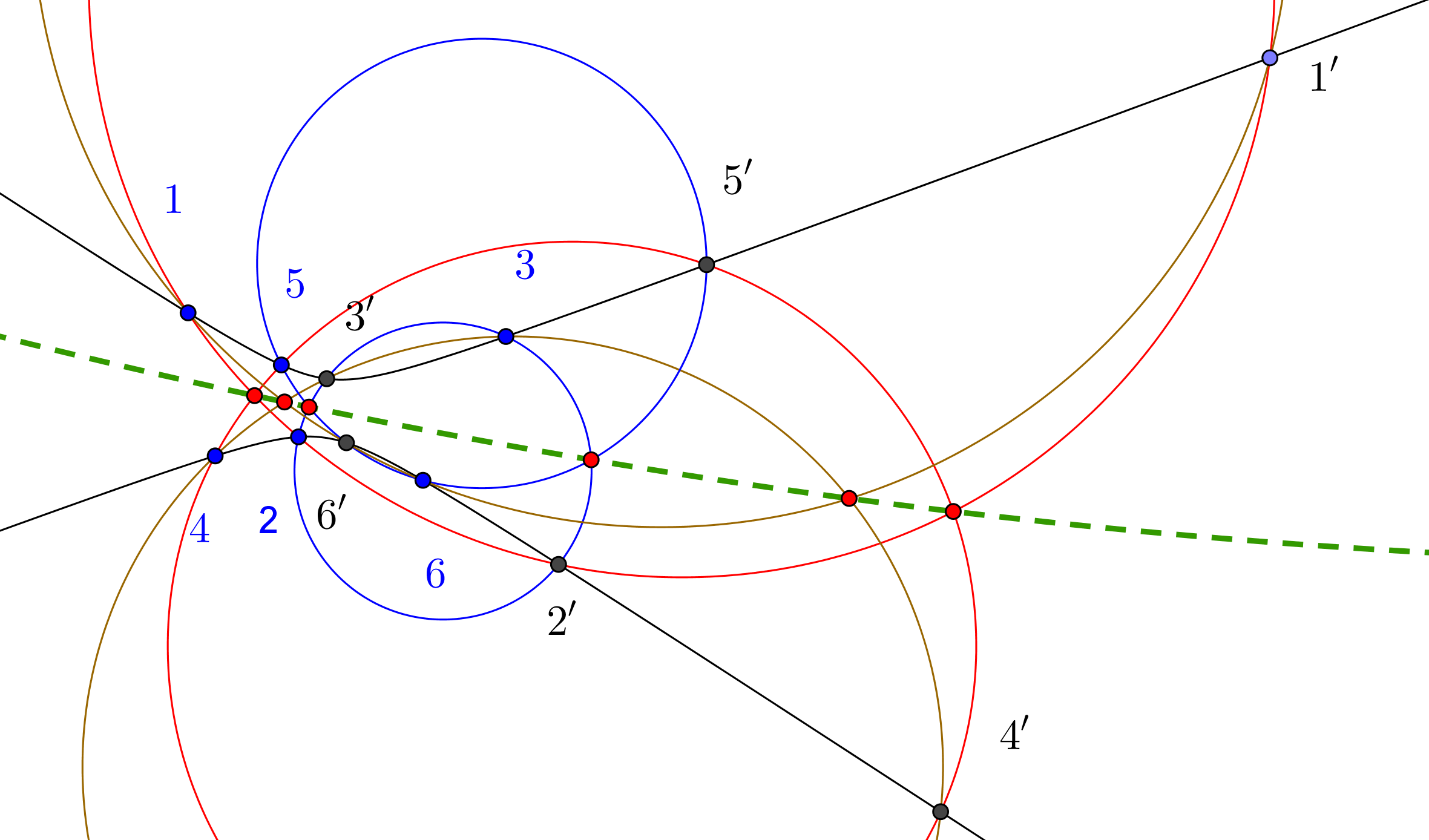
Please see a chain of six circles associated with a conic. This is a generalization of Pascal theorem, Pappus theorem. I reformulate as following:

Let $1, 2, 3, 4, 5, 6$ be six arbitrary points in a hyperbola. Let $1'$ be arbitrary point in the hyperbola. The circle $(121')$ meets the hyperbola at point $2'$. The circle $(232)$ meets the hyperbola again at $3'$, define points $4', 5', 6'$ similarly. Let circle $(121')$ meets the circle $(454')$ at $A, B$, Let circle $(232')$ meets the circle $(565')$ at $C, D$. Let circle $(343')$ meets the circle $(616') $ at $E, F$. Then six points $A, B, C, D, E, F$ lie on a circle.

enter image description here

Special case:

1. If $1'$ at $\infty$, six circles are six lines, so the theorem is Pascal theorem.

2. If the hyperbola is two lines, and $1'$ at $\infty$ then six circles are six lines, the theorem is Pappus theorem

enter image description here

My question: Can generalization the result above to Higher Dimensions?

Improve this question
$\endgroup$
7
  • $\begingroup$ I don't know why some ones put on hold ? $\endgroup$
    – Cố Gắng Lên
    Commented Jun 21, 2017 at 15:26
  • 1
    $\begingroup$ MathOverflow is simply not the right place to ask questions about elementary Euclidean geometry, unless they are connected with research topics. Please post them on Math.Stackexchange instead. $\endgroup$
    – Gro-Tsen
    Commented Jun 21, 2017 at 18:48
  • $\begingroup$ This is a generalization of one great theorem in Euclidean Geometry. I ask can generalization this? $\endgroup$
    – Cố Gắng Lên
    Commented Jun 21, 2017 at 22:38
  • $\begingroup$ I understand, but this is not a research problem, and elementary Euclidean geometry is not a research topic, so this is not the right place. $\endgroup$
    – Gro-Tsen
    Commented Jun 22, 2017 at 7:40
  • 7
    $\begingroup$ @Gro-Tsen Euclidean and axiomatic geometry are research topics. $\endgroup$
    – user40276
    Commented Jun 23, 2017 at 4:58

0

Reset to default