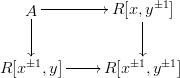What is the relationship between connective and nonconnective derived algebraic geometry?

Question

"Derived algebraic geometry" usually means the study of geometry locally modeled on "$Spec R$" where $R$ is a connective $E_\infty$ ring spectrum (perhaps with further restrictions). Why "connective", though?

In my (limited) understanding, approaches to the subject like that of Toen and Vezzosi are motivated as an approach to studying things like intersection theory in ordinary algebraic geometry. The picture is that a connective $E_\infty$ ring $R$ (incarnated as a simplicial commutative ring, usually) is an "infinitesimal thickening" of the ordinary ring $\pi_0 R$. This picture breaks down if $R$ is not connective, motivating one to restrict attention to the connective case (moreover, I don't know of a way to model nonconnective ring spectra analogous to simplicial rings).

But another motivation comes from homotopy theory, $TMF$, and the moduli stack of elliptic curves, which is a nonconnective derived Deligne-Mumford stack. When the basic motivating objects are nonconnective, it leaves me puzzled that Lurie continues to focus primarily on the connective case in Spectral Algebraic Geometry.

I see basically two mutually exclusive possible reasons for this:

1. The theory of nonconnective derived algebraic geometry is wild / ill-behaved / hard to understand, so one restricts attention to the connective cases which is more tractable.

2. The theory of nonconnective derived algebraic geometry is a straightforward extension of the theory of connective derived algebraic geometry; it is easy to study nonconnective objects in terms of connective covers, but the results are most naturally phrased in terms of the connective objects, so that's the way the theory is expressed.

Question A. Which of (1) / (2) is closer to the truth?

Maybe as an illustrative test case, here are two statements pulled at random from SAG. Let $R$ be a connective $E_\infty$ ring, let $Mod_R$ denote its $\infty$-category of modules, and $Mod_R^{cn}$ its $\infty$-category of connective modules (both of which are symmetric monoidal), and let $M \in Mod_R$.

• $M$ is perfect(=compact in $Mod_R$) iff $M$ is dualizable in $Mod_R$.

• $M$ is locally free (= retract of some $R^n$) iff $M$ is connective and moreover dualizable in $Mod_R^{cn}$.

Question B. Do these statements have analogs when $R$ is nonconnective? If so, are they straightforward extensions of these statements from the connective case?

For Question B, feel free to substitute a better example of a statement if you like.

Answer 1

Here is an example of a nonconnective $E_\infty$ ring spectrum which, I think, illustrates a key problem. (A more extensive discussion of this phenomenon occurs in Lurie's DAG VIII and in a paper by Bhatt and Halpern-Leinster.)

Let $R$ be an ordinary commutative ring, viewed as an $E_\infty$ ring concentrated in degree zero, and $A$ be the homotopy pullback / derived pullback in the following diagram of $E_\infty$ rings:

Then $\pi_0 A = R[x,y]$ and $\pi_{-1} A$ is the local cohomology group $R[x,y] / (x^\infty, y^\infty)$; all the other homotopy groups of $A$ are zero. As a result, there's a map $R[x,y] \to A$ of $E_\infty$ rings, and any $A$-module becomes an $R[x,y]$-module by restriction.

Here's a theorem. The forgetful map from the derived category $D(A)$ to the derived category $D(R[x,y])$ is fully faithful, and its essential image consists of modules supported away from the origin. This extends to an equivalence of $\infty$-categories.

We could think of this in the following way. The ring $A$ is the $E_\infty$ ring of sections $\Gamma(\Bbb A^2 \setminus \{0\}, \mathcal{O}_{\Bbb A^2})$ on the complement of the origin in affine 2-space over $R$, and the above tells us we actually have an equivalence between $A$-modules and (complexes of) quasicoherent sheaves on $\Bbb A^2 \setminus \{0\}$.

Here are some takeaways from this.

• Nonconnective ring spectra are actually quite natural. Global section objects $\Gamma(X, \mathcal{O}_X)$ are usually nonconnective, and we're certainly interested in those.

• The above says that even though the punctured plane is not affine but merely quasi-affine, it becomes affine in nonconnective DAG. This is a general phenomenon.

• Solely on the level of coefficient rings, the map $R[x,y] \to A$ looks terrible. It is indistinguishable from a square-zero extension $R[x,y] \oplus R[x,y]/(x^\infty,y^\infty)[-1]$. (There is more structure that does distinguish them.)

Many of the definitions as given in DAG for a map are given in terms of the effect (locally) of a map $B \to A$ of ring spectra (e.g. flatness, étaleness, etc etc). For connective objects, this works very well. However, we have just shown that for nonconnective objects, a map of ring spectra may have nice properties—the map $Spec(A) \to Spec(R[x,y])$ should be an open immersion!—which are completely invisible on the level of coefficient rings. This goes for the rings themselves and doubly so for their module categories.

If I have one point here, it is that trying to give definitions in nonconnective DAG in terms of coefficient rings is like trying to define properties of a map of schemes $X \to Y$ in terms of the global section rings $\Gamma(Y,\mathcal{O}_Y) \to \Gamma(X,\mathcal{O}_X)$. This makes nonconnective DAG fundamentally harder.

So far as your question A, this places me somewhere in between your two options (1) and (2). I don't think (1) is right because I think that nonconnective objects are much too important; I have a mild objection to the language in (2) because I don't think that nonconnective objects are straightforward.

Answer 2

As Tyler pointed out, it is "too easy" to be representable in the non-connective world. This might sound good, but it comes at the cost of geometric intuition. It is related to the fact that negative homotopy groups of the cotangent complex arise from "stacky" phenomena, while in the non-connective setting it will be impossible to distinguish what comes from stackiness and what comes from non-connectiveness of the rings themselves. I will try to give an example of this below.

1) First, a slight reformulation of Tyler's example (just to show that this is a very general phenomenon). Let $X = Spec(A)$ be an affine scheme and $U \subset X$ a quasi-compact open subscheme.

Lemma: When considered as a nonconnective spectral scheme, $U$ is affine.

Proof: $U$ can be written as the vanishing locus of some perfect complex $F \in Perf(X)$. In other words, as a non-connective spectral stack, the functor of points of $U$ is as follows: a $T$-point $T \to U$ is a $T$-point $x : T \to X$ such that $x^*(F) = 0$. According to Prop. 1.2.10.1 in Toën–Vezzosi's HAG II, there exists a canonical epimorphism $A \to B$ of non-connective $E_\infty$-ring spectra such that $Spec(B)$ has the functor of points described. (This $B$ is discrete if and only if $U$ is actually affine as a classical scheme.)

2) Let $X$ be a (connective) spectral scheme and let $\mathcal{A}$ be a quasi-coherent $\mathcal{O}_X$-algebra. Consider the relative Zariski spectrum, the (connective) spectral stack $Spec_X(\mathcal{A})$ whose space of $T$-points is $Maps_{\mathcal{O}_T\text{-alg}}(x^*(\mathcal{A}), \mathcal{O}_T)$, for any $X$-scheme $x : T \to X$. In particular you can take $\mathcal{A} = Sym_{\mathcal{O}_X}(F)$ for any perfect complex $F$; let $V_X(F) := Spec_X(Sym_{\mathcal{O}_X}(F))$ denote the "generalized vector bundle" associated to $F$.

One can compute (see Theorem 5.2 in Antieau-Gepner) the relative cotangent complex of $V_X(F)$ at any point $s : T \to V_X(F)$, for an $X$-scheme $x : T \to X$, as $x^*(F)$. You can read off a lot of information about $V_X(F)$ from the cotangent complex. Namely, say $F$ is of tor-amplitude $[a,b]$ (I'm going to use homological grading). If $a \ge 0$, i.e. $F$ is of non-negative tor-amplitude (hence connective), then $V_X(F)$ is representable by a (connective) spectral scheme (which is affine over $X$): by Zariski descent, you can assume $X$ is affine, and then $V_X(F) = Spec(\Gamma(X, Sym_{\mathcal{O}_X}(F)))$. If $a \le 0$, then $V_X(F)$ is a spectral $(-a)$-Artin stack (this is what I meant about the cotangent complex controlling "stackiness"). If further $b \le 0$ then $V_X(F)$ is smooth.

That was the connective story. In the non-connective world, $V_X(F)$ will "automatically" become representable by a non-connective spectral scheme even when $F$ is non-connective. In other words, by passing to the non-connective world, we allowed ourselves to replace stacks by "schemes", but on the other hand we lost something significant: it is not clear anymore what information we can read from the cotangent complex about the geometry of the "scheme".