Addition, multiplication and exponentiation have important combinatorial analogues. By looking at if there a combinatorial analogue of the "sum over all possible paths", I hope to shed light on if there is a combinatorial analogue for tetration, or iterated exponentiation. So I'm looking for references regarding combinatorial analogues of the "sum over all possible paths"?
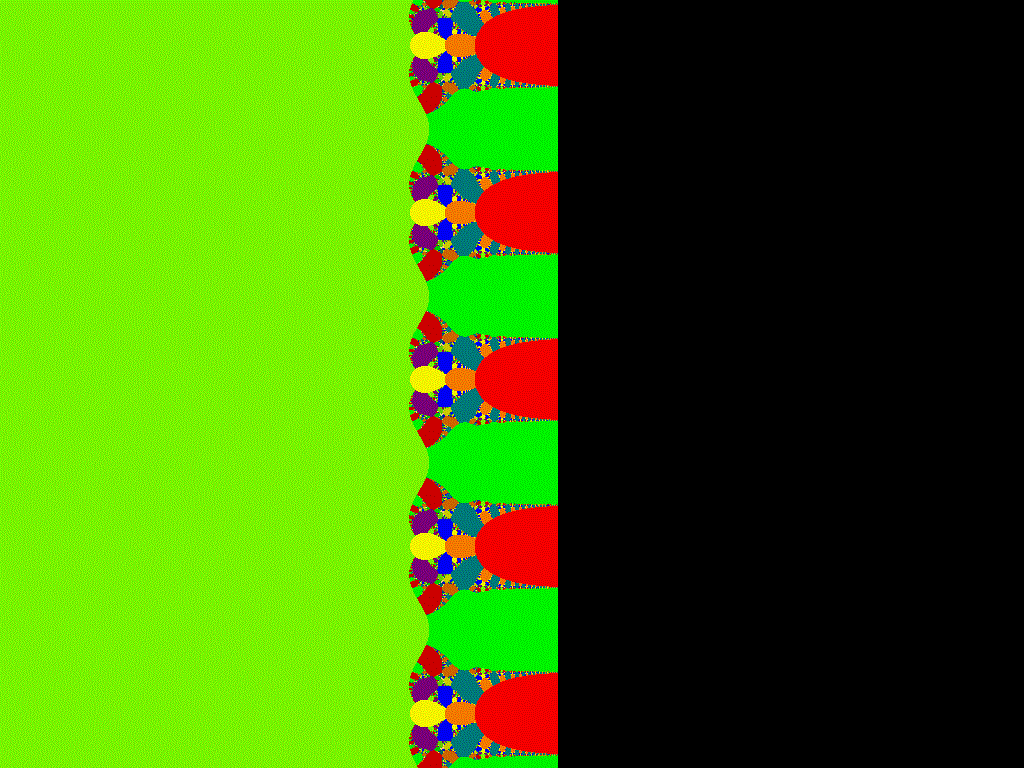
The justification of the question follows. The following picture is the Julia set of the exponential map, which displays the dynamics in the complex plane of the iteration of the exponential function $e^z$ or more simply tetration. The black part of the picture represents numbers too large to be modeled by the program used to make the fractal.
The fractal contains an infinite number of copies of the same structure and like other fractal is self-similar, you can zoom into the fractal structure as far as you want and you will only see repetitions of the same fractal structure. Now we are going to map the two dimensional complex plane into the one dimensional real line.

Note the periodic structure of the Julia set of the exponential map dividing the complex plane into bands. The exponential map has a countably infinite number of fixed points, $e^{z_n}=z_n$. Each band $n$ is associated with a fixed point $z_n$. Consider the exponential Julia set divided into identical bands where each individual band contains a fractal structure representing a point on a real line in physical space. So the Point 1 band contains fixed point $z_1$ and represents a single point in physical space while the Point 2 band represents a different single point. Now we consider the problem of how the points in the Point 1 band transition to the Point 2 band; where the forward orbits of the exponential function map points from Point 1 to Point 2. The largest set of points that transition from Point 1 to Point 2 directly transition there in a single step. Because of the fractal nature of the Julia set you also need to consider that given any other Point $k$, there will also be a transition from Point 1 to Point $k$ and then Point 2, a transition taking two steps, but the number of points making the two step transition are fewer by far than the number of points making a direct transition. It turns out that you can find points transitioning from Point 1 to Point 2 taking all possible paths. It appears that the number of points or area transitioning are governed by the power law of the number of steps in the transition.
Now consider a toy quantum field theory of a one dimensional physical space. Once again consider a Point 1 on the real number line and a Point 2 on the number line. The Feynman Path Integral, gives the transition amplitude from Point 1 to Point 2. The transitions follow what are referred to as "the sum of all possible paths", that there is a direct transition from Point 1 to Point 2 in a single step. Given any other Point $k$, there will also be a transition from Point 1 to Point $k$ and then Point 2, a transition taking two steps. Just like the transitions from the band Point 1 in the Julia set to the band Point 2, the Feynman Path Integral also obeys the same pattern of transitions. Once again, the number of points or area making a specific transition are determined by a power law and the number of step taken.