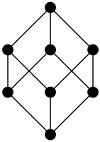
Let $\mathcal{P}$ be an irreducible subfactor planar algebra and $\mu$ the Möbius function of its biprojection lattice $[e_1,id]$. Then the Euler characteristic of $\mathcal{P}$ is defined as follows:
$$\chi(\mathcal{P}) := -\sum_{b \in [e_1,id]} \mu(b,id)|id:b|$$
Question: Is $\chi(\mathcal{P})$ nonzero for any irreducible subfactor planar algebra $\mathcal{P}$?
Note that $\mu(id,id)=1$ and $\mu(b,id) = -\sum_{b' \in (b,id]}\mu(b',id)$, so it is computed recursively.
Remark: we use the name "Euler characteristic" because for any interval of finite group $[H,G]$, $$\chi(H,G) = \chi(\mathcal{P}(R \rtimes H \subset R \rtimes G))$$ is the (reduced) Euler characteristic of the order complex of the coset poset $$\{ Kg \ | \ K \in [H,G), \ g \in G \}. $$
Gaschütz showed that $\chi(G)=\chi(1,G)$ is nonzero if $G$ is solvable and the question whether $\chi(G)$ is nonzero for any finite group $G$ is an open problem motivated by Brown (see arXiv:1406.6067).
We have checked by GAP that $\chi(G) \neq 0$ for $|G| \le 100$, and $\chi(H,G) \neq 0$ for $|G:H| \le 31$. Moreover for $\hat{\chi}(H,G) = \chi(\mathcal{P}(R^G \subset R^H))$ and $\hat{\chi}(G)=\hat{\chi}(1,G)$, same checking as above.
This open problem is reputed hard in group theory, so I don't expect a general planar algebraic proof as answer. What I can expect is a subfactor counterexample, or some indications showing whether the planar algebra framework is relevant.
Model for a counter-example: Assume $\mathcal{P}$ to be an irreducible subfactor planar algebra of index $\phi^8$, with a biprojection lattice $[e_1,id]$ which is boolean of rank $3$
and such that any atom $b $ of $ [e_1,id]$ satisfies $|b:e_1| = \phi^2$ and $|b^{\complement}:e_1| = \phi^6$.
Then $-\chi(\mathcal{P}) = \phi^8 -3\phi^6+3\phi^2-1 = 0.$
The existence of such a planar algebra is unknown (to me). Note that $\phi^8 \simeq 46.98$.
The Euler characteristic should not be confused with the Euler totient defined (in arXiv:1703.04486) by:
$$\varphi(\mathcal{P}) := \sum_{b \in [e_1,id]} \mu(b,id)|b:e_1|$$
Note that $\varphi(\mathcal{P}(R \rtimes H \subset R \rtimes G))$ is the cardinal of $\{ Hg \ | \ \langle Hg \rangle = G , \ g \in G \}$.
Recall that the reciprocal of the Riemann zeta function can be written as the Dirichlet series: $$\frac{1}{\zeta(s)} = \sum_{n \ge 1} \frac{\mu(n)}{n^s} = \sum_{H \in [\{1\},\mathbb{Z}]}\frac{\mu(H,\mathbb{Z})}{|\mathbb{Z}:H|^s} $$
By analogy, the zeta function of $\mathcal{P}$ can be defined as the reciprocal of the finite Dirichlet-like series:
$$\frac{1}{\zeta(\mathcal{P},s)} := \sum_{b \in [e_1,id]} \frac{\mu(b,id)}{|id:b|^s}$$
Then $$ \varphi(\mathcal{P}) = \frac{\delta^{2}} {\zeta(\mathcal{P},1)} \ \text{ and } \ \chi(\mathcal{P}) = \frac{-1}{\zeta(\mathcal{P},-1)}$$