Is there an example of a pair $M,N$ of connected, oriented equidimensional Riemannian manifolds with the following properties:
$M$ is everywhere non-flat, $N$ is flat.
There exist a map $f:M \to N$ which is differentiable almost everywhere (a.e), and $df$ is an orientation-preserving isometry a.e.
(An easier goal: Find a pair of manifolds which are not locally isometric, but which admit a map as in 2. We should probably restrict here to manifolds without boundary, since otherwise $M=[0,1],N=\mathbb{R},f(x)=x$ is an example. )
Context:
The point is to see whether curvature differences obstruct existence of a.e orientation-preserving isometries.
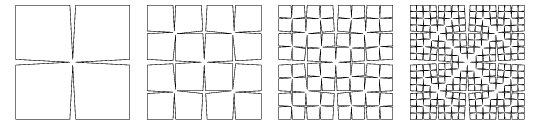
If we omit the requirement on the orientation, then there is a lot of flexibility; Gromov showed that for any metric $g$ on the unit $d$-dimensional disk $\mathbb{D}^d$ there is an a.e isometry $f:(\mathbb{D}^d,g) \to (\mathbb{R}^d,e)$. ($e$ is the Euclidean metric).
Further comments:
- Gromov's a.e isometry cannot be orientation-preserving:
It's $1$-Lipschitz, and hence in $W^{1,\infty}(M,N)$, and every map $f \in W^{1,\infty}(M,N)$ satisfying $df \in \text{SO}$ a.e is smooth. (Thus Gromov's map cannot be orientation-preserving or orientation-reversing on any open subset of the domain. It must "switch" orientations in an"infinite rate").
- An a.e orientation preserving isometry does not need to be smooth:
For an example take $M=[0,1],N=[0,2],f(x)=c(x)+x$ where $c$ is the Cantor function. Then $f'=1$ a.e.
This example can be used to show that there is an a.e orientation-preserving isometry from a circle of radius $1$ into a circle of radius $2$. (Of course, there is no smooth local isometry from the former into the latter).