Analytic continuation of definite integral?

Question

This question comes from physics...

We have the following functional (or function dose not matter): $$S\left[x\right]:=\intop_{-\frac{t_{0}}{2}}^{+\frac{t_{0}}{2}}dt\,\mathcal{L}\left[x\right]\::\:{\displaystyle \mathcal{L}}:={\displaystyle \frac{m}{2}\left(\frac{dx}{dt}\right)^{2}-V\left[x\right]}$$

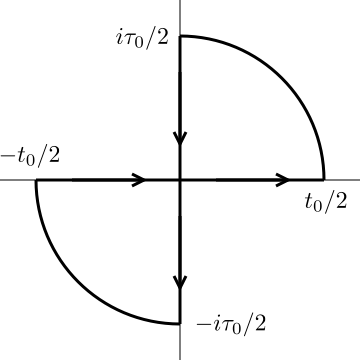
Religiously speaking, how to do an analytic continuation for $S$?

Usually, we follow this by the so called Wick rotation $t\rightarrow-i\tau$, this leads to get $iS=-S_{E}$ where: $$S_{E}:={\displaystyle \intop_{-\tau_{0}/2}^{+\tau_{0}/2}\mathcal{L}_{E}\,d\tau}\::\:\mathcal{L}_{E}\left[x\right]:={\displaystyle \frac{m}{2}\left(\frac{dx}{d\tau}\right)^{2}+V\left[x\right]}$$

While it is clear how to do the Wick rotation, It seems to me that I am missing something in relation to integral boundaries, where somehow $i$ disappears there after Wick rotation, I think the reason burred in how to analytically continue $S$ at first place.

Answer 1

This image should explain the Wick rotation, I hope:

The segment of the contour along the real $t$ axis may need to be deformed so that it avoids any poles, but when it encloses no poles the contour integral is zero. The contributions from the two quarter circles cancel when the integrand is an even function, so then the integral $\int_{-t_0/2}^{t_0/2}dt$ along the real axis equals the integral $\int_{-\tau_0/2}^{\tau_0/2}d\tau$ along the imaginary axis, upon analytical continuation of the integrand from $t\mapsto i\tau$.

In words, there is no $i$ in the integral boundaries because what you are carrying out is not a change of variables, but a $\pi/2$ rotation of the integration path.