I was reading about horseshoes and heterclinic bifurcation but my knowledge of dynamical systems is really old fashioned.
as I understand the local stable manifold and the local unstable manifold intersect. I am looking for a differential equation or geometric construction that demonstrates this phenomenon
(actually the paper says "surface diffeomorphism" which I just took to mean "differential equation")
this seems highly improbable. for example the flow defined by
$$ \frac{df }{ dt } = (x^3 + ax +b) f $$
could never exhibit such self-tangent orbits. perhaps I am looking at the wrong place for example?
I also don't understand how the stable and unstable manifolds can intersect.
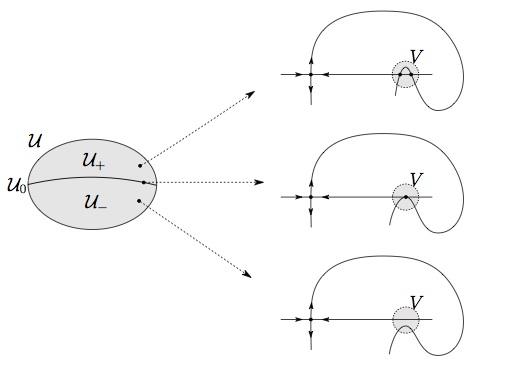
Grover is correct. The blog talks about a $C^2$ diffeomorphism $f:M\to M$ it has a horseshoe and a periodic point. And the local (un)stable manifolds have to do with the periodic point $p \in K$. These two manifolds have quadratic tangency at some other point $q \in M - K$ (which I guess means these curves agree to second $o(x^2)$ rather than just linearly.
I had not even begun to read the rest of the blog about horseshoe dynamics. The blog does not name any map in particular. Just looking for a specific $f$ that might work (if that's even possible). There's no reason to believe an algebraic example should work here but there must have been some classical instance of this.
