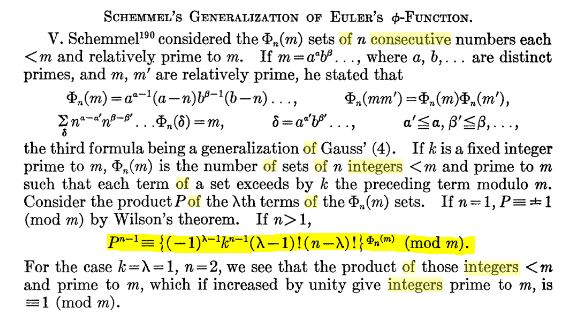
The case $n=4$ and $m=35$ given in both the question and the original German paper (link now removed from question) is a little misleading. In this case the sets are disjoint. The case $n=2$ and $m=35$ shows a bit better what's going on. We're now counting pairs $(d,d+1)$ with both $d$ and $d+1$ coprime to 35, and these pairs can overlap (for example we count $(1,2)$ and $(2,3)$ etc). Clearly if $n$ is at least the smallest prime factor $p$ of $m$ then $\Phi_n(m)=0$ because in a run of $n$ consecutive integers, one will be a multiple of $p$, and in this case the yellow highlighted formula just claims that $1=1$, so this is fine.
To do the interesting case where $n$ is less than the smallest prime factor of $m$, we first get a feel for the question by proving the first formula. To give a run of $n$ consecutive integers is to give the smallest one, and if they're all prime to $p$ ($p$ some prime factor of $m$) then the smallest one had better be congruent to one of $1,2,3,\ldots,p-n$ modulo $p$. There are hence $p-n$ choices mod $p$ for the first term in the run, so if $p^a$ is the exact power of $p$ dividing $m$ then there are $p^{a-1}(p-n)$ choices mod $p^a$ for the first term, and the first formula follows by CRT. We also have a "formula" now for the $\lambda$'th term in any such run.
Now to the proof of the highlighted formula. WLOG $k=1$, because $k$ is coprime to $m$ by assumption, so every run with common difference $k$ is just $k$ times a run with common difference 1 (note that runs with common difference 1 will never "overflow" mod $m$ but runs with common difference $k$ might, as the 35 example shows).
By CRT it suffices to check the congruence modulo $p^a$ where $p$ is an arbitrary prime factor of $m$ and $p^a$ divides $m$ exactly. The $\lambda$'th term in a run of $n$ consecutive integers all coprime to $p$ will be $i+\lambda$ mod $p$ with $i=0,1,2,\ldots,p-n-1$, and we don't care what's going on modulo other primes, by CRT. So we set $D=\Phi_n(m/p^a)$ and observe that $\Phi_n(m)=(p-n)p^{a-1}D$.
Now I have to break into two cases because my brain hurts otherwise. If $a=1$ then modulo $p$ we have
$$P=\left((\lambda)(\lambda+1)\cdots(\lambda+p-n-1)\right)^D$$
and if we set $Q=\left((\lambda)(\lambda+1)\cdots(\lambda+p-n-1)\right)$ then by Wilson's theorem we have $Q(\lambda-1)!(-1)^{n-\lambda}(n-\lambda)!=-1$ modulo $p$. The highlighted assertion modulo $p$ now follows from Wilson's theorem and Fermat's Little Theorem.
If $a>1$ then it's a bit messier, unless I missed a trick. The same strategy works but we need to do all computations modulo $p^a$. Here's a sketch of something which will work. To proceed in this way, the sort of question we need to know the answer to is this: what is the product, modulo $p^a$, of all the integers between 1 and $p^a$ which are congruent to $\lambda,\lambda+1,...,p-n-1+\lambda$ modulo $p$? There's a trick for this though; to deal with the terms congruent to $j$ modulo $p$ we can replace $j$ by $j^{p^{a-1}}$; this doesn't change $j$ modulo $p$, but mod $p^a$ it replaces it by its Teichmueller lift which is a $p-1$st root of unity. So if $X$ is the product mod $p^a$ of all the numbers congruent to 1 mod $p$, then the product is $X^{p-n}$ multiplied by some $p-1$st root of unity congruent to our messy factorial thing $Q$ above, modulo $p^a$. This $p-1$st root of unity is also a Teichmueller lift, and you can see this on the right hand side because the factor $\Phi_n(m)$ has a factor of $\Phi_n(p^a)$ and hence of $p^{a-1}$. For $p=2$ the argument is a little different because you need to work mod 4. This reduces the question (modulo some unenlightening algebra) to computing $X$, the product mod $p^a$ of all the elements of $(\mathbf{Z}/p^a)^\times$ which are congruent to 1 mod $p$; for $p>2$ this is 1 mod $p^a$ because each element is cancelled by its inverse; for $p=2$ it's a bit messier but not really much harder (you just need to keep track of the elements of order 2). I don't really want to write down the details because I need to do the kitchen :-/ but if you really want to prove the result you should hopefully be able to piece it together from this.