typo in Selberg's "An Elementary Proof of the Prime-Number Theorem"

Question

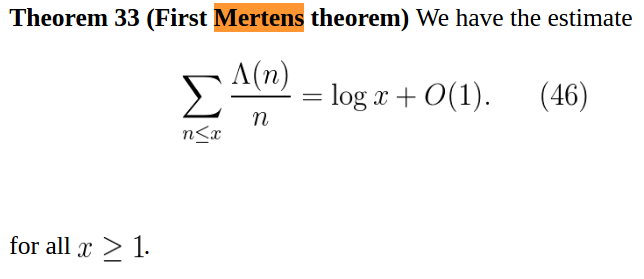
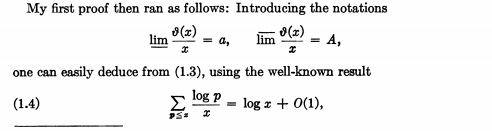I am asking about Selberg's elementary proof. There seem to be a few key points. One is his symmetry formula:

$$ \sum_{pq \leq x} \log p \, \log q + \sum_{p \leq x} \log^2 p = 2x \log x + O(x) $$

Instead let's start from a more basic starting point. Selberg uses:

$$ \sum_{p \leq x} \frac{\log p}{x} = \log x + O(1) $$

This would have $\theta(x) = x \log x + O(x)$. Merten's theorem is a bit different:

$$ \sum_{p \leq x} \frac{\log p}{p} = \log x + O(1) $$

Both of them are true I believe. Which one can be used to show this error term is small?

$$ \sum_{p \leq x} \log p \;\log \frac{x}{p} \stackrel{?}{=} O(x) $$

Hopefully I have copied the statements correctly.

---

Here is the passage I am referring to:

If I multiply both sides by $x$ I get that $\sum_{p \leq x} \log p = x \log x + O(x)$ which GH from MO says is false. And I don't see why he is using Prime Number Theorem to prove the Prime Number Theorem.

Yet he also says that Merten's theorem is false. Here is one statement

and I do not understand the downvoting since the equations I have written are accurate reflection of Mertens' theorem and Selberg's typo.

Answer 1

The last display in your original post is used in (2.5) of Selberg: An elementary proof of the prime-number theorem, Annals of Math. 50 (1949), 305-313. It is a simple consequence of Chebyshev's estimate $\theta(t)=O(t)$, and it goes as follows: $$ \sum_{p \leq x} \log p \;\log \frac{x}{p} = \int_{2-}^x\left(\log\frac{x}{t}\right)d\theta(t) = \left[\left(\log\frac{x}{t}\right)\theta(t)\right]_{2-}^x-\int_2^x \left(\log\frac{x}{t}\right)'\theta(t)\,dt.$$ The first term on the right hand side is zero, hence we get $$ \sum_{p \leq x} \log p \;\log \frac{x}{p} = \int_2^x\frac{\theta(t)}{t}\,dt= \int_2^x O(1)\,dt = O(x).$$ Done.