Topological properties of complex valued Riemann sum limit curve and a particular integral inequality

Question

I am studying under what conditions the following integral inequality would hold ($a$ real, $a>0$): $$ \int_{-\infty} ^{\infty} \frac{f(ix)}{a\pm ix}dx\ = 0 \ \ \ \ \Rightarrow \ \ \ \int_{-\infty} ^{\infty} \frac{f(ix)}{a \mp ix}dx\ \neq 0 $$ whereby the notation $f(ix)$ is meant to clarify that we are referring to a continuous complex valued function of the real variable $x$. Furthermore said function features the property that the integral of its ratio with one or the other of the two possible denominators $(a \pm ix)$ vanishes.

Let us take the very simple example $f(ix) = e^{ix}$, then $$ \int_{-\infty} ^{\infty} \frac{e^{ix}}{a-ix}dx\ = 0 $$ whereas $$ \int_{-\infty} ^{\infty} \frac{e^{ix}}{a+ix}dx\ = \frac{2 \pi }{e^{a}} $$ Thus, when the integral for the case "minus sign" at the denominator vanishes, the integral for the "plus sign" does not. What I am trying to find out is whether this particular example can possibly be extended to a more general case, whereby it would be sufficient to know that one of said two integrals (e.g. the "minus sign" case for the particular example above) is = 0 to hence prove that the other cannot be. Recalling that (when both limits exist):

$$ \int_{-\infty} ^{\infty} \frac{e^{ix}}{a-ix}dx\ = \lim_{t \to \infty}\int_{0} ^{t} \frac{e^{ix}}{a-ix}dx \ - \ \lim_{t \to \infty}\int_{0} ^{-t} \frac{e^{ix}}{a-ix}dx $$

let us now try to visualize the result of the first integration as the difference vector (meaning the vector representation of the complex valued difference) joining the two end points (by end point I mean the corresponding values pair when $t \rightarrow \infty $) of the two parametric curves (blue): $$ \int_{0} ^{t} \Re \left( \frac{e^{ix}}{a - ix} \right) dx \ \ \ , \ \ \int_{0} ^{t} \Im \left( \frac{e^{ix}}{a - ix} \right) dx $$ $$ \int_{0} ^{-t} \Re \left( \frac{e^{ix}}{a - ix} \right) dx \ \ \ , \ \ \int_{0} ^{-t} \Im \left( \frac{e^{ix}}{a - ix} \right) dx $$
In the limit $t \rightarrow \infty $ both said points coincide (as the joining vector, i.e. the value of the integral from $-\infty $ to $+\infty $, vanishes). Similarly, for the "plus sign" at the denominator case (green curves): $$ \int_{0} ^{t} \Re \left( \frac{e^{ix}}{a + ix} \right) dx \ \ \ , \ \ \int_{0} ^{t} \Im \left( \frac{e^{ix}}{a + ix} \right) dx $$ $$ \int_{0} ^{-t} \Re \left( \frac{e^{ix}}{a + ix} \right) dx \ \ \ , \ \ \int_{0} ^{-t} \Im \left( \frac{e^{ix}}{a + ix} \right) dx $$

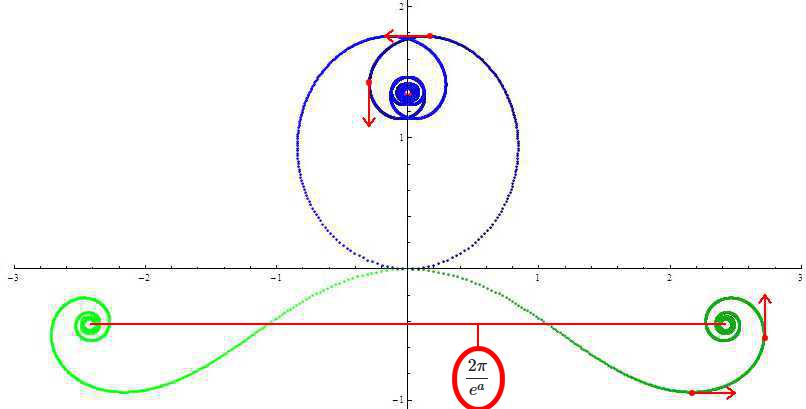
The curves in the plot at the end illustrate the example $a=0.25$, and are obtained by approximating the above integral by means of the corresponding Riemann sums (whose limit for vanishing $\Delta_i$ is then the integral of interest) adding up the "infinitesimal" vectors $e^{ix_n}/(a \pm ix_n) \ \Delta x$ (for an introduction to this approach to the visualization of complex valued integrals I have much enjoyed this wonderful book http://usf.usfca.edu/vca/): $$ \sum_{n=1}^{T} \frac{e^{ix_n}}{a \pm ix_n} \ \Delta x \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \sum_{n=-1}^{-T} \frac{e^{ix_n}}{a \pm ix_n} \ \Delta x $$

(for said plot the approximations are $\Delta x = 0.01$ and sums ending at $t= \pm 25$, i.e. $x_n = n \Delta x$ $T=25/\Delta x = 2500$). BLUE and LIGHT BLUE refer to the $e^{ix}/(a - ix)$ integrand, branch to $+t$ and to $-t$ respectively. GREEN and LIGHT GREEN have corresponding meanings, though for the opposite sign case $e^{ix}/(a + ix)$.

In this particular example the integral (i.e. the difference vector) vanishes for the "minus sign" case. Thus, the two branches $\pm \infty $ endpoint limits must coincide. In other words, the corresponding Riemann sum limit curve is a closed curved (BLUE + LIGHT BLUE when taken to $t \rightarrow \infty $). Whereas for the other sign case (the green branches) the limit curve is open. It is easy to verify how the difference vector (red line) joining the two endpoints of the GREEN + LIGHT GREEN branches indeed approximates $\frac{2 \pi }{e^{a}} = 4.89... $

From a topological perspective one might observe that the GREEN + LIGHT GREEN curve may be obtained from the BLUE + LIGHT BLUE by rotation of each "infinitesimal" vector $e^{ix_n}/(a -ix_n) \ \Delta x$ by the angle $-2\arctan \frac{x_n}{a}$ (because $a+ix = (a-ix)e^{i2\arctan \frac{x}{a}}$). This would also suggest that, for any given $t$, the lengths of the green and blue curves are identical.

Try now to follow said "infinitesimal" vectors rotation:

• the green and blue curves start out from the origin with the same tangent,
• at any given value of $x$ the tangent to the green curve appears then rotated, wrt to the tangent to the blue curve, by a progressively increasing angle (as $x$ increases),
• this up to the asymptotic value $-2\arctan \frac{x}{a} \rightarrow \pi$ (one can easily recognize this in the example plot, whereby the red arrows are pointing in the direction of the tangent vector to the curves at same values of $x \gg a$).

If we take any closed curve (not necessarily a simple one), we cut it at one point, and we redraw it in such a way that at any point the original value of the tangent vector to the curve is now rotate by 180°, we will obtain a curve which is also closed (and symmetrical to the one we started from). Intuitively, one might expect that if, instead of considering a fixed 180° rotation angle, we progressively increase said angle along the length of the curve (we have first to agree on the starting point, which is the origin in the example of the plot below), starting from 0° at $x=0$ and up to the asymptotic 180°, we would instead obtain an open curve. However, intuition alone is often misleading, and unfortunately a rigorous proof has so far eluded me.

To reframe the above observation in the language of differential geometry, by expressing the starting curve in terms of its tangent vector angle $\varphi$ considered as a function of the arc length $s$, i.e. $\varphi = g(s)$ (Whewell equation), the "twisted around" curve can be described by the following transformation: $$ \varphi = g(s) \ \ \ \ \Rightarrow \ \ \ \ g(s) - 2\arctan \frac{x(s)}{a} $$ which can then be reformulated in terms of the Cesàro equation ($k(s)=g'(s)$): $$ k(s) \ \ \ \ \Rightarrow \ \ \ \ k(s) - 2\frac{a}{a^2+x(s)^2} \ x'(s) $$

Finally, I am looking for suggestions and advice on whether, to your knowledge, there exist general topology theorems or results which could possibly be of any help in proving the integral inequalities we started from, based only on topological arguments aimed at proving that a closed curve (e.g the one described by the vanishing integral as $t \rightarrow \infty $), cut at the point $t =\infty $, then "twisted around", starting from the origin, in such a way that at each point the tangent vector to the original curve is rotated by $2 \arctan (x/a)$, would result in a new curve which is instead necessarily open.

Answer 1

More a comment than an answer, but too long anyway for a comment. There is nothing weird or mysterious about your first equalities: with $R>a>0$, we have from the residue formula, $$ \int_{[-R,R]}\frac{e^{ix}}{a+i x} dx=-\int_0^π\frac{e^{iRe^{it}}}{a+i Re^{it}} iRe^{it} dt+ 2iπ\frac1i e^{i(ia)}. $$ The limit when $R$ goes to $+\infty$ of the second integral is easily seen to be 0: just check $$0=\lim_{R\rightarrow +\infty}\int_0^π e^{-R \sin t} dt,$$ and we get your $2πe^{-a}$. Replacing $ix$ by $-ix$ does not change much the second integral, but the root of the denominator is then $-ia$ in the lower half-plane, so that the residue is 0, because our half-circle is located in the upper half-plane.

To go back to your initial question: you put your hand on a meromorphic function $F$ without pole on the real line and you know that $$ \lim_{R\rightarrow +\infty} \int_{0}^{π} F(Re^{it}) iRe^{it} dt=0. $$ Then, with $A_+$ standing for the (countable) set of poles of $F$ in the upper half-plane, we find, $$ \lim_{R\rightarrow +\infty} \int_{-R}^{R} F(x) dx=2iπ \sum_{a_j\in A_+} \text{Res}(F,a_j) $$ so that the existence of the limit in the lhs is equivalent to the convergence of the series in the rhs (true in the case $A_+$ is finite). A typical occurrence of the phenomenon you are looking for is when all the poles of $F$ are located in the upper half-plane (or all the poles of $F$ are located in the lower half-plane).