Xu He's article Rigidity of Infinite Disk Patterns and I have a problem with a statement he makes on page 7.
He considers weighted embedded planar graphs $G=(V, E)$ with weight function $\Theta: E \to [0, \frac{1}{2}\pi]$ and defines a vertex $v_0$ of $G$ to be interior if there is a closed chain of neighbouring vertices $v_1, v_2, \dots, v_l$, where either $l\geq 4$, or $l=3$ and each edge $[v_0, v_k]$ is irreducible, $1\leq k\leq 3$ (given $4$ vertices $v_1, v_2, v_3, v_4=v_0$ of the graph $G$ an edge $[v_0, v_2]$ is called reducible if $[v_{i-1}, v_i] \in E$ and $\Theta[v_{i-1}, v_i]=\frac{1}{2}\pi$ for $1 \leq i \leq 4$, and $\Theta[v_0, v_2]=0$). Otherwise, it is called a boundary vertex.
Claim: Suppose $T$ is the $1$-skeleton of a triangulation of a planar surface possibly with boundary so that each boundary component has at least four vertices, and the following two conditions hold
- If a simple loop in $T$ formed by three edges $e_1, e_2, e_3$ separates the vertices of $T$ (meaning there is a pair of vertices in the complement $T\backslash (e_1 \cup e_2 \cup e_3)$ so that any path joining the vertices passes through the set $e_1 \cup e_2 \cup e_3$), then $\sum_{i=1}^3 \Theta(e_i)<\pi$.
- If $v_1, v_2, v_3, v_4=v_0$ are distinct vertices in $T$ and if $[v_{i-1}, v_i] \in E$ and $\Theta([v_{i-1}, v_i])=\frac{1}{2}\pi$ for $i=1, 2, 3, 4$, then either $[v_0, v_2]$ or $[v_1, v_3]$ is an edge in $T$.
Then a vertex in $T$ is interior (as defined above) precisely when it lies in the interior of the surface.
I can't seem to be able to prove the direct implication: if $v$ is an interior vertex, then it belongs to the interior of the surface. In fact it seems to me that the triangulation of the closed disk below is a counterexample to the claim.
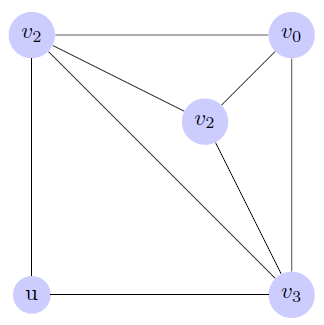
The vertex $v_0$ has a closed chain of neighbouring vertices $v_1, v_2, v_3$. Since the edges $[v_0, v_2]$, $[v_2, v_3]$ and $[v_3, v_0]$ form a simple loop in $T$ that separates the vertices of $T$ (namely, the vertices $v_2$ and $u$) we must have $\Theta([v_0, v_2])+\Theta([v_2, v_3])+\Theta([v_3, v_0])<\pi$ by condition $(1)$, which implies none of the edges $[v_0, v_i]$ is reducible for $i=1, 2, 3$. So $v_0$ is an interior vertex, but clearly does not belong to the interior of the disk.
Am I missing something? I will be very thankful if anybody can explain why the claim is true and what goes wrong with the above ''counterexample''.
