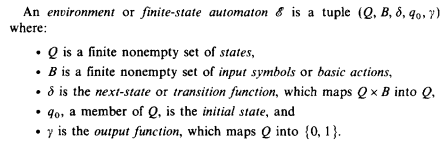
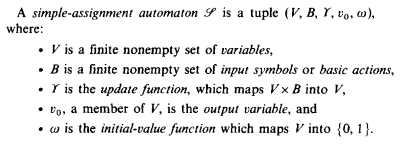
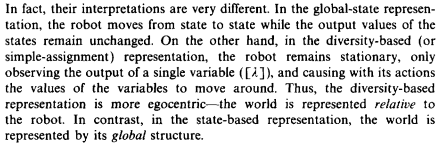
First, I agree with the commenters in not understanding your objection to giving different names to the same mathematical objects if they are used to model different phenomena. The terms "position" and "velocity" can both refer to vectors in $\mathbb{R}^3$, but this doesn't imply that using both terms is bad habit or that there are modeling gaps that need filling in.
When modeling some phenomenon, it's important to distinguish between the mathematical structure of a thing and the meaning given to this structure. (For example, in CS we often refer informally to the need for both syntax and semantics.)
One good example is the pair $G = (V,E)$ where $V$ is a finite set and $E \subseteq V \times V$. We often interpret this structure as a "connectivity graph" where $(u,v) \in E$ has the semantic meaning "there is an edge from $u$ to $v$" and it makes sense to ask the question "Is there any path from $s$ to $t$?" where a path is a sequence of pairs $((u_1,v_1), \cdots, (u_n,v_n))$ where $v_i = u_{i+1} ~ (\forall 1
\leq i M n)$ and each $(u_i,v_i) \in E$.
On the other hand we can also use $G$ as a model of eligible partners where $(u,v) \in E$ has the semantic meaning "$u$ can be assigned as a partner to $v$" and a reasonable question is "what is the largest matching in $G$?" where a matching is an $M \subseteq E$ where $(u_1,u_2),(u_3,u_4) \in M \implies u_i \neq u_j (\forall i < j)$.
Of course, you could technically ask the path question in the second example or the matching question in the first example, but it wouldn't make much sense, nor does restricting ourselves to only one interpretation of the formalism $G = (V,E)$.
More concretely, it sounds like you might want to check their prior work for more explanation, as they say (p302):

See the bottom of p304 and top of p305 for a reiteration of this point.
So it looks like to me that what's happening is that the same phenomenon -- i.e. a robot with a set of things it can sense and actions it can take -- is being modeled two different ways. These two ways have the same formalism but different meanings. The first, state-based way, explicitly tracks all possible states the world might be in, the set $Q$. The second, "diversity-based" way tracks the number of equivalence classes of "tests" that produce different results for the robot; this is modeled by $V$.
Importantly, a particular state-based representation (your first definition) models the same phenomenon as a particular diversity-based representation (your second definition), but the mapping between these is NOT the identity!