The Leech lattice and the 23 Niemeier lattices make a single genus. How does it break up into spinor genera?
$\begingroup$
$\endgroup$
4
-
$\begingroup$ see mathoverflow.net/questions/54027/… I think it likely that the genus and spinor genus coincide, as the masses of spinor genera in a genus are equal. However, if you can find a partition of the list of automorphism counts with two equal reciprocal sums, maybe... $\endgroup$– Will JagyCommented Oct 13, 2016 at 17:21
-
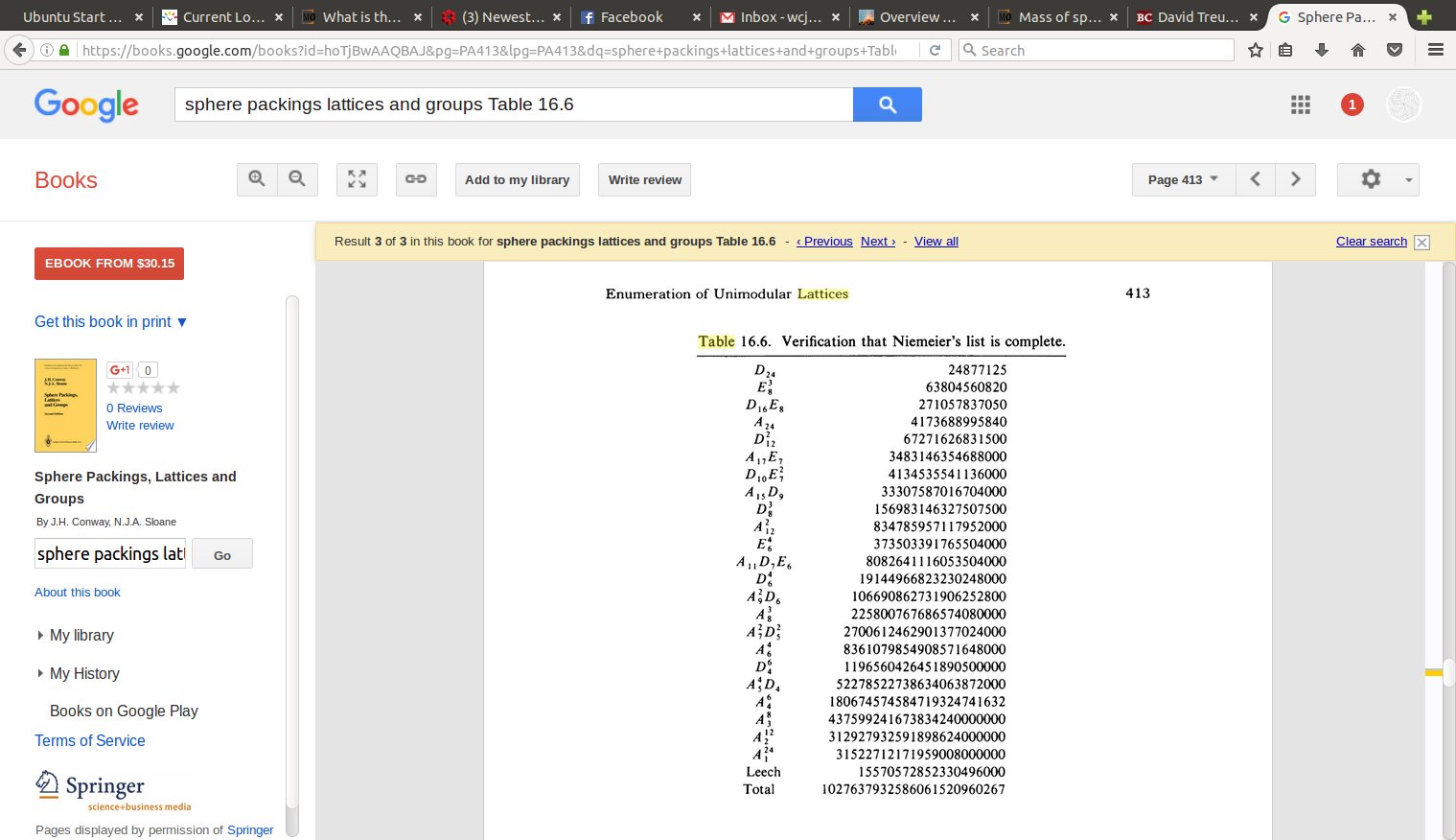
$\begingroup$ table 16.6 on page 413 of SPLAG gives the reciprocal of the automorphism count, all multiplied by a huge common denominator. So, one may try to partition 16.6 into two sets with equal sums. $\endgroup$– Will JagyCommented Oct 13, 2016 at 17:25
-
$\begingroup$ hmmmm. All those numbers are even except for $D_{24},$ so such a partitioning is impossible. Similar, all the numbers are divisible by $5$ except $A^6_4.$ That seems to be it. $\endgroup$– Will JagyCommented Oct 13, 2016 at 17:34
-
$\begingroup$ Thanks Will. How is the mass of a spinor genus defined? Should I weight each lattice by the order of a finite subgroup of Spin(24), and if so which subgroup? $\endgroup$– David TreumannCommented Oct 13, 2016 at 17:55
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
see Mass of spinor genus, positive integral quadratic forms for the statement that spinor genera in the same genus have the same mass, answer by Schulze-Pillot.
The spinor genus count being a power of two, if there were more than one, we would be able to collect the reciprocal automorphism counts into two subsets with equal sum. However, from SPLAG Table 16.6 on page 413, we see that this impossible. All but one number are even, the number for $D_{24}$ is odd. All but one number are divisible by $5,$ the number for $A^6_4$ is equivalent to $2 \pmod 5.$
The mass is just the sum of the reciprocals of the automorphism counts. An automorphism meaning, given the Gram matrix $G$ of a positive form, an integer matrix $A$ such that $A^T G A = G.$ The set of such $A$ is finite when $G$ is positive. For the automorphism group of a positive form, or lattice $\Lambda,$ SPLAG writes $|\mbox{Aut}(\Lambda)|.$ For a spinor genus, we just sum up the reciprocals of these integers for all equivalence classes of forms/lattices in the spinor genus. Oh, SPLAG does not list these counts as such for this genus, the counts are enormous.
answered Oct 13, 2016 at 18:00
$\begingroup$
$\endgroup$
The genus of every even unimodular $\mathbb Z$-lattice has only one spinor genus. See O'Meara, particularly Example 102:10.
