What is the most symmetric configuration of four 2-surfaces linked in $S^4$?

Question

What are some of the most symmetric configurations of four 2-surfaces linked in the 4-dimensional sphere $S^4$?

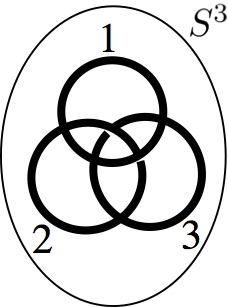
To make a lower-dimensional analogy, recall that in 3-dimensional sphere $S^3$, we can have a very symmetric configuration of three 1-circles $S^1$ linked in the 3-dimensional sphere $S^3$, as the Borromean rings.

We can define the Milnor’s triple linking number $\mu$ of three $S^1$ circles in $S^3$ (e.g. the page 9 of arxiv/1101.3374) for Borromean rings, and we can find that the relabeling of 1,2,3 circles (for examples the labels 1,2,3 can be labels of gauge fluxes / conjugacy classes for a QFT gauge theory with oriented Wilson loops) will not change the absolute value of triple linking number: $$|\mu(1,2,3)|=|\mu(2,3,1)|=|\mu(3,1,2)|=|\mu(1,3,2)|=|\mu(3,2,1)|=|\mu(2,1,3)|,$$ they only differ by the orientations of the curves, thus differ by an orientational multiplied factor $\pm 1$.

We can imagine some linkings of the 2-surfaces defining topological invariance numbers which are very symmetric respect to interchanging the indices of surfaces. One candidate can be the triple linking number $Tlk(\Sigma_1,\Sigma_2,\Sigma_3)$ of three 2-surfaces $\Sigma_1,\Sigma_2,\Sigma_3$ in $S^4$, the one defined in the book "S. Carter, J. Carter, S. Kamada, and M. Saito, Surfaces in 4-Space, Encyclopaedia of Mathematical Sciences (Springer, 2004)." See also an online Ref: arxiv/math/0007141. However, this number is only symmetric (up to orientation ) respect to exchange the first and the last surface indices, $$Tlk(\Sigma_1,\Sigma_2,\Sigma_3)=-Tlk(\Sigma_3,\Sigma_2,\Sigma_1)$$ $$|Tlk(\Sigma_1,\Sigma_2,\Sigma_3)|=|Tlk(\Sigma_3,\Sigma_2,\Sigma_1)|$$ This number is not symmetric respect to exchange the middle surface with the first or the last surface, so $|Tlk(\Sigma_1,\Sigma_2,\Sigma_3)|\neq |Tlk(\Sigma_1,\Sigma_3,\Sigma_2)|$ and $|Tlk(\Sigma_1,\Sigma_2,\Sigma_3)|\neq |Tlk(\Sigma_2,\Sigma_1,\Sigma_3)|$ in general.

Naturally, since the three 2-surfaces linked in the 4-dimensional sphere $S^4$ do not provide such a symmetric topological invariance and do not provide the configuration analogous to the dimensionally-extended "Borromean tori" in $S^4$, we will instead look for four 2-surfaces linked in the 4-dimensional sphere $S^4$, and define the linking invariances:

question 1: What are some of the most symmetric configurations of four 2-surfaces linked in the 4-dimensional sphere $S^4$?

I imagine that certain topological invariance $Q(1,2,3,4) \equiv Q(\Sigma_1,\Sigma_2,\Sigma_3,\Sigma_4)$ of four surfaces $\Sigma_1,\Sigma_2,\Sigma_3,\Sigma_4$ are symmetric under exchanging the labels of $\Sigma_1,\Sigma_2,\Sigma_3,\Sigma_4$ with a fixed configurations. $$|Q(1,2,3,4)|=|Q(2,3,4,1)|=|Q(3,4,1,2)|=\dots=|Q(1,3,2,4)|=|Q(3,2,4,1)|=|Q(2,4,1,3)|=\dots$$ for all $4!=24$ interchanging of labelings.

question 2: What are the topological invariance detects this symmetric configuration of four 2-surfaces linked in the 4-dimensional sphere $S^4$?

Intuitively, the 2-surfaces can be $S^2$ or $T^2$ for simplicity.

This can be naively a dimensional extended story from the analogous Borromean rings in $S^3$ to four 2-surfaces in $S^4$.

Answer 1

There are some very symmetric links of spheres in higher dimensions. A nice construction is in Rolfsen, Knots and Links, Chapter 7.J. He finds Brunnian (delete one component and the rest is a trivial link) links with $k$ components in $S^n$ for $n \geq 3$ and for any $k$. From the construction, there is a least a $k$-fold cyclic symmetry, and perhaps a dihedral symmetry as well. The non-triviality of these links is detected by the Alexander polynomial for the $n$-dimensional homology of the infinite cyclic cover, which works out to be $(1-t_1)(1-t_2)\cdots(1-t_k)$.