2
$\begingroup$

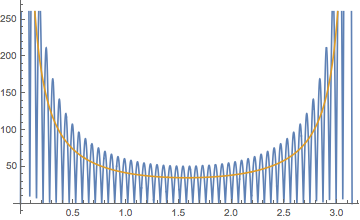
Consider the space $\mathrm{SU}(2)^\natural$ of conjugacy classes in $\mathrm{SU}(2)$. It has a natural identification with the interval $[0,\pi]$ with Haar measure $\frac{2}{\pi} \sin^2\theta\, \mathrm{d}\theta$, via the mapping $$ \theta \mapsto x_\theta = \begin{pmatrix} e^{i\theta} \\ & e^{-i\theta}\end{pmatrix} . $$ Under this identification, the map $U_k\colon \mathrm{SU}(2)^\natural \to \mathbf{R}$ given by $U_k(x) = \mathrm{tr}(\mathrm{sym}^k x)$ is $$ U_k(\theta) = \frac{\sin((k+1)\theta)}{\sin(\theta)} . $$

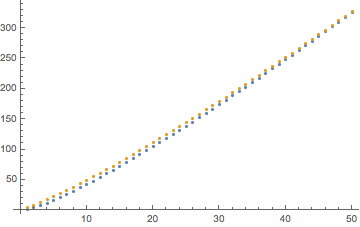
I am wondering: is the exact value of the total variation of the functions $U_k$ known? In this context, this means: is there an exact formula (or asymptotic for) $$ \int_0^\pi |U_k'(\theta)|\, \mathrm{d}\theta ? $$

More generally, for $G$ a compact Lie group, we can identify $G^\natural$ with a quotient of Euclidean space via $\exp\colon \mathfrak{t} \to G^\natural$ for $\mathfrak{t}$ the Lie algebra of a maximal torus. Is there any reasonable formula for the total variation of the trace of an irreducible representation of $G$, in terms of the corresponding highest weight?

Improve this question
$\endgroup$
2
  • $\begingroup$ What do you mean by variation? For the usual compact Lie groups, the irreducible characters are similarly expressible (and the formula above is simply the character of the irreducible representations, restricted to a maximal torus). $\endgroup$
    – David Handelman
    Commented Sep 29, 2016 at 14:14
  • $\begingroup$ I'm interested in the asymptomatics of the above integral as $k$ approaches infinity. $\endgroup$
    – Daniel Miller
    Commented Sep 29, 2016 at 17:40

1 Answer 1

Reset to default
4
$\begingroup$

For the large-$k$ asymptotics I would first approximate $|U'_k(\theta)|$ by its envelope $$F_k(\theta)=\frac{k}{\sqrt{2}\sin\theta},$$ plotted together for $k=50$:

The divergence of $F_k(\theta)$ at $\theta=0$ is cut-off at $\theta_1=1/k$, and similarly the divergence at $\theta=\pi$ is cut-off at $\theta_2=\pi-1/k$. Integration of $F_k(\theta)$ from $\theta_1$ to $\theta_2$ produces the asymptotics

$$\int_{0}^\pi |U'_k(\theta)|\,d\theta\rightarrow\sqrt{2}\,k\log(2k),\;\;k\gg 1.$$

A numerical evaluation of the integral (orange points) seems quite close to this asymptote (blue points):

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .