1
$\begingroup$

There seems to exist an asymptotic line
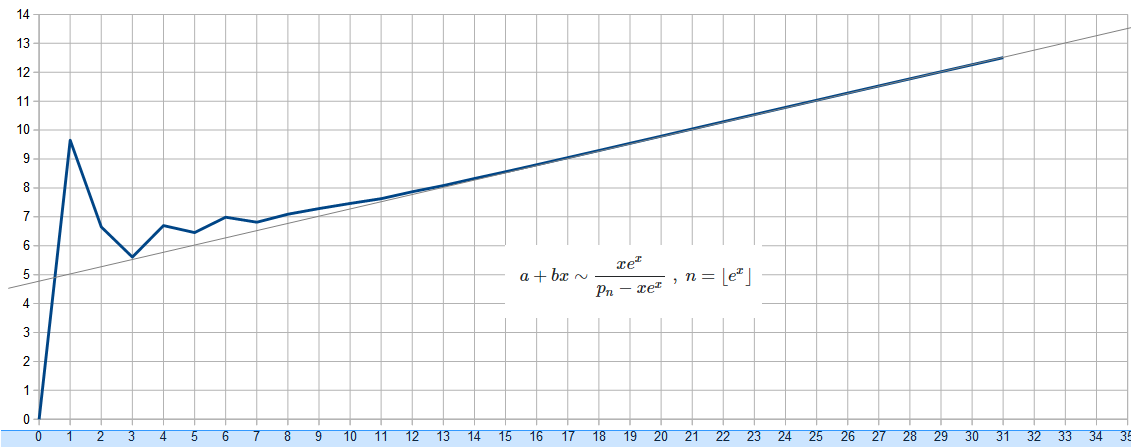
$$\displaystyle a+bx\sim \frac{x e^x}{p_{n}-x e^x}\; ,\;n=\lfloor e^x\rfloor\tag1$$ enter image description here enter image description here Which suggests an estimation

$$\displaystyle g(n)=\Big(1+\frac{1}{a+b\ln n}\Big)\cdot n \ln n\tag2$$ of $p_n$.

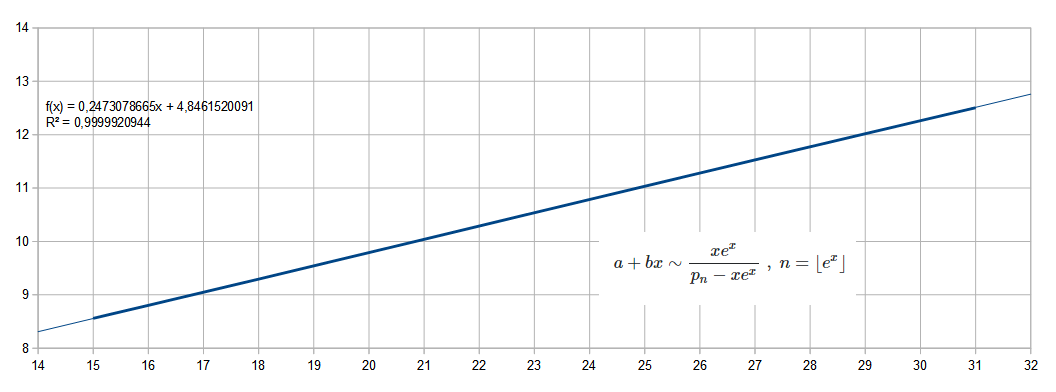
With $a=4,8461520091$ and $b=0,2473078665$, from the linear regression in the diagram above, $|p_n-g(n)|<|p_n-n\cdot(\ln n+\ln\ln n -1)|$ for $30456<n\leq5761455$, that is up to all primes $<100,000,000$ (the present limit of my prime number table). For $1941$ numbers $n\leq 30456\;\; g_n$ is less accurate.

Is this estimation known?

Are there methods to calculate values of $a$ and $b$?

Can the asymptotic equivalence in $(1)$ be proved?

No, I guess that Vincenzo Oliva on MSE is right. The accuracy comes from a local approximation and b must be a function of n that very slowly approach to zero when $n\to \infty$.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
5
$\begingroup$

Your right hand side is asymptotically $x/\log x$, hence it cannot be approximated well by a linear function. More precisely, with your notation, we have $$ p_n=n\bigl(\log n+\log\log n+O(1)\bigr) = \bigl(e^x+O(1)\bigr)\bigl(x+\log x+O(1)\bigr),$$ whence $$\frac{xe^x}{p_n-xe^x}=\frac{xe^x}{e^x\bigl(\log x+O(1)\bigr)}=\frac{x}{\log x+O(1)}.$$

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .