Sofa in a snaky 3D corridor

Question

What is the largest volume object that can pass though a $1 \times 1 \times L$ "snaky" corridor, where $L$ is large enough to be irrelvant, say $L > 6$.

---

         

---

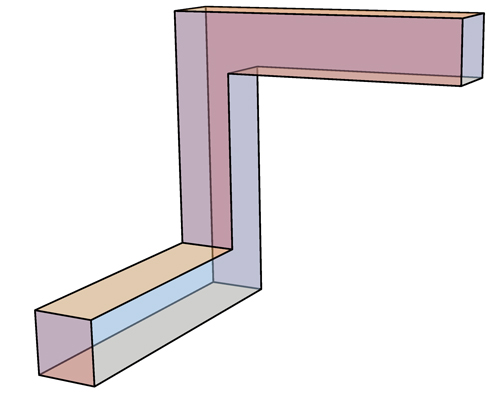
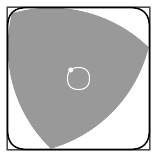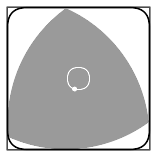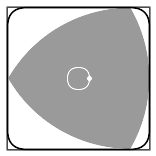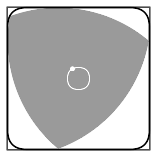
This is a 3D version of the 2D sofa-moving problem, which has been heavily studied. See especially Dan Romik's web pages. The optimal-area 2D sofa is conjectured to be Gerver's (slight) modification of Hammersley's shape, the latter of which I show below, extruded in 3D to fill the corridor.

---

         

---

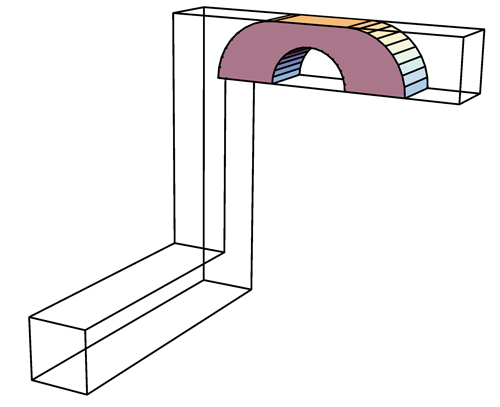
There are two natural candidates: (1) Slice the extruded 2D optimal shape, in the orthogonal direction, so it can negotiate both turns in the same manner. See image added below. (2) Rotate the illustrated shape $90^\circ$ but shear-off every portion that falls outside the $1 \times 1$ corridor.

A basic question is: Is either of these the optimal solution, or can one identify some shape that beats both? An even more basic (and easier) question is: Which of (1) or (2) has larger volume?

Added:

---

         
          ^{The intersection of the two shapes can pass through the corridor.}

---

         
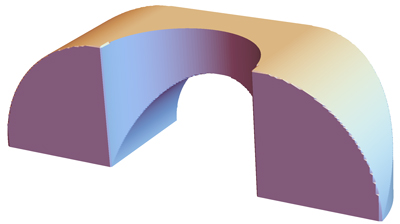
          ^{The intersection. (Thanks to J.M. & JackLaVigne @MathematicaSE.)}

---

Volume: $\frac{4 \left(8+\pi^3\right)}{3 \pi ^3} \approx 1.67735$.

Answer 1

[Not a solution, just answering the "even more basic" question of the original post.]

The Hammersley sofa is given by cutting a semicircle of radius $2/\pi$ out of a $1\times 2/\pi$ rectangle and adding unit-radius quarter discs to the sides:

This sofa has area $\frac{\pi}2+\frac2\pi-\frac2{\pi^2}=2.2017$, so of course its 3D variant extruded by a distance of $1$ in the $z$-axis has the same volume.

If we intersect this sofa with a cylinder of radius $0.5$ positioned within the corridor (i.e. $(x-0.5)^2+(z-0.5)^2\le0.25$), the resulting shape will be able to rotate after making the first turn, so that it will be in position for the second turn. The volume of this rounded shape is given by

$$\int_0^12\sqrt{0.25-(y-0.5)^2} \left(\frac2\pi+2\sqrt{1-y^2}\right)\,dy $$ $$- \int_0^{2/\pi}2\sqrt{0.25-(y-0.5)^2}\cdot2\sqrt{\frac4{\pi^2}-y^2}\,dy$$ $$\approx1.76755 > 1.67735$$

so it is an improvement over the intersection with a $90$-degree rotated copy. (One could probably improve slightly on this by performing the same operation to Gerver's improved sofa, but the exact integral becomes much more ugly.)

One could try intersecting with other square rotors, like a Reuleaux triangle at various orientations:

                                          

Empirically this didn't seem to improve things, as might be expected from the fact that the Releaux triangle is the minimal-area square rotor. Area isn't the only consideration here though, since the weighted sum of the widths of our rotor at different heights is what matters.