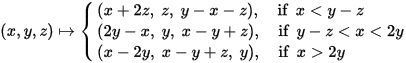0
$\begingroup$

Zagier's has found a famous one sentence proof for Fermat's theorem on sums of two squares. It centers on the following involution of the set $S= \lbrace (x,y,z) \in N^3: x^2+4yz=p \rbrace $ having exactly one fixed point.

enter image description here

The author of this question as well as several other sources say, that this can easily been seen. Wikipedia states that the fixed point is $(1, 1, k)$. Now I perfectly understand that $(1, 1, k)$ always falls into the second case of the involution and returns $(1, 1, k)$ thus being a fixed point, but I completely fail to recognize that there is absolutely no possibility for another fixed point to exist.

I started out trying to find another (which might be very difficult), and came up with $(x, x, 1)$ but i did not find an example for which $(x, x, 1)$ is part of the given set of prime numbers. Still I don't see why it can't be possible that there is another fixed point for some $x$ I haven't found yet.

Can anyone show that to me?

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ Maybe it is worth remarking that the crucial argument in this "one-sentence" proof is actually due to Heath-Brown $\endgroup$
    – Francesco Polizzi
    Commented Jul 27, 2016 at 12:24

1 Answer 1

Reset to default
4
$\begingroup$

Setting the coordinates to be equal, it's clear that the only fixed point in $\mathbb R^3$ for the top map is (0,0,0), and ditto for the bottom map. Finally, as you (almost) noted, the fixed points in $\mathbb R^3$ of the middle map are the points of the form $(x,x,z)$. But for a point of this form, the quantity $x^2+4yz$ is $x^2+4xz=x(x+4z)$. Now, if $x$ and $z$ are in $\mathbb N$, the only way for $x(x+4z)$ to be prime is for $x=1$, and then also one needs $1+4z$ to be prime.

Improve this answer
$\endgroup$
2
  • $\begingroup$ The fact that $(x,x,z)$ actually corresponds to a divisor of $n=x^2+4yz$ can be used to factor $n$ if it is not prime, using the Zagier maps. Take for example $n=p_1 p_2$, with two distinct primes $3 \mod 4$. For details see section 2.2. of my paper "A Combinatorial Approach to Sums of Two Squares and Related Problems " link.springer.com/chapter/10.1007%2F978-0-387-68361-4_8, (or paper 30 of: math.tugraz.at/~elsholtz/WWW/papers/papers.html ) Starting with $(1,1,k)$ and iterating the two Zagier maps one must come to a fixed point of the form $(x,x,z)$. Very slowly algorithm. $\endgroup$
    – Christian Elsholtz
    Commented Jul 27, 2016 at 12:57
  • $\begingroup$ @Joe Thank you. That was prompt and very clear. Good explanation. I just noticed that i should've posted this on Math.StackExchange. My bad. Thank you anyway. $\endgroup$
    – Toastgeraet
    Commented Jul 29, 2016 at 21:36

Not the answer you're looking for? Browse other questions tagged .