1
$\begingroup$

Let $T_{n}(b)$ be the $n\times n$ Toeplitz matrix determined by the symbol $$ b(z)=\frac{1}{z}+\sum_{j=0}^{k}a_{j}z^{j} $$ where $k\in\mathbb{N}$ and $a_{0},\dots,a_{k}\in\mathbb{R}$, $a_{k}\neq0$. That means $T_{n}(b)$ is the $n\times n$ left-top truncation of the banded Toeplitz Hessenberg matrix $$ T(b)=\begin{pmatrix} a_{0} & 1 & 0 & 0 & \\ a_{1} & a_{0} & 1 & 0 & \ddots \\ a_{2} & a_{1} & a_{0} & 1 & \ddots \\ a_{3} & a_{2} & a_{1} & a_{0} & 1 & \ddots \\ & \ddots & \ddots & \ddots & \ddots\\ \vdots & & \\ a_{k} \\ & \ddots \\ \end{pmatrix}\!.$$

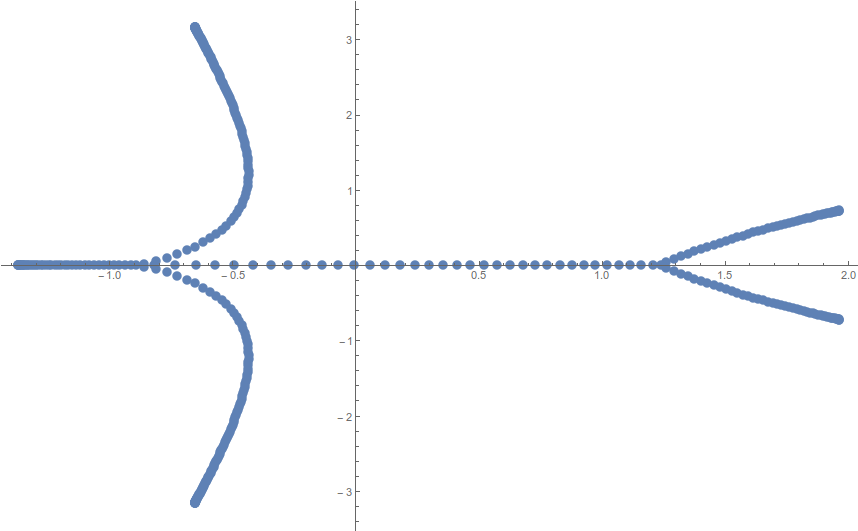
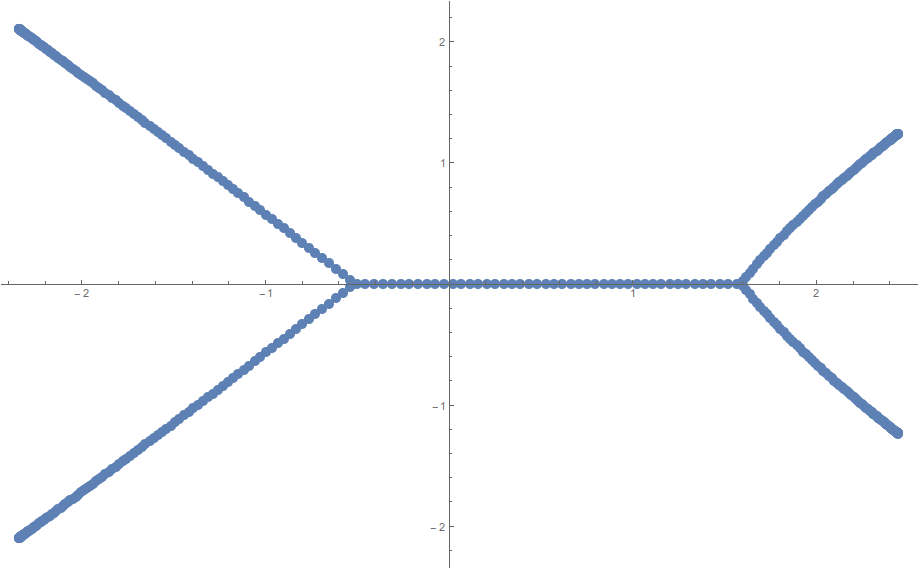
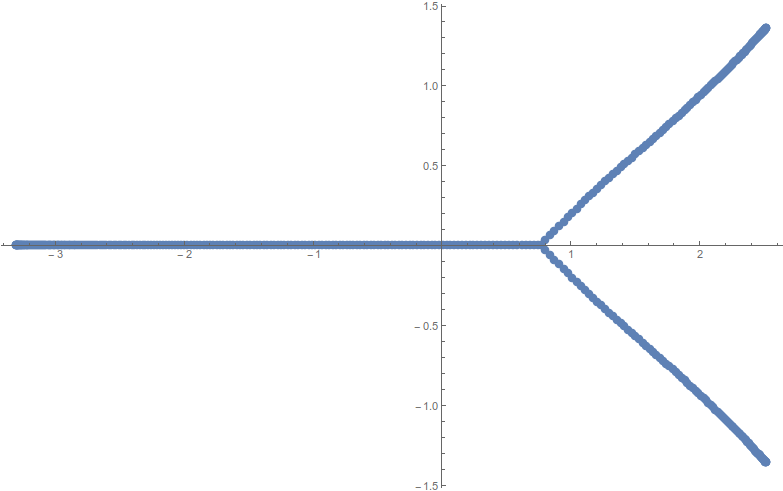
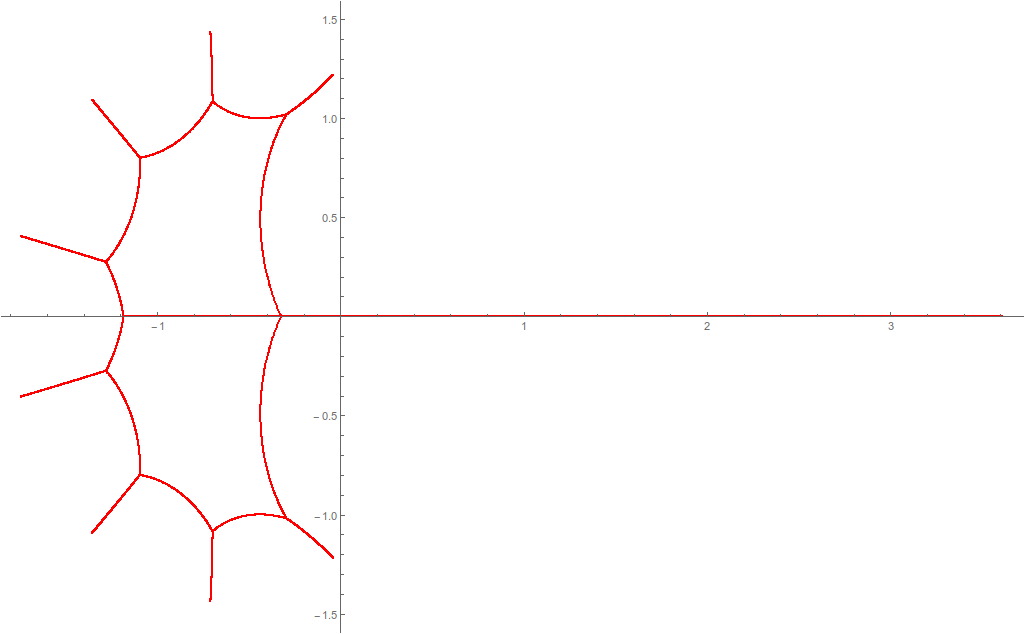
Further, for $\lambda\in\mathbb{C}$, let $z_{0}(\lambda),\dots,z_{k}(\lambda)$ denote the roots of the equation $b(z)=\lambda$ (repeated according to their multiplicity) arranged such that $$|z_{0}(\lambda)|\leq|z_{1}(\lambda)|\leq\dots|z_{k}(\lambda)|.$$ Schmidt and Spitzer (1960) showed that the set $$\Lambda(b):=\{\lambda\in\mathbb{C} \mid |z_{0}(\lambda)|=|z_{1}(\lambda)|\}$$ coincides with the set of limit points of eigenvalues of matrices $T_{n}(b)$, as $n\to\infty$.

There is a strong numerical evidence (I add 3 pictures approx. $\Lambda(b)$ below) that the set $\mathbb{C}\setminus\Lambda(b)$ is connected though all my attempts to prove this assertion failed. So my question is: $$\textbf{Is the set } \mathbb{C}\setminus\Lambda(b) \textbf{ connected?}$$

Relevant remarks:

  1. If $b$ is a general Laurent polynomial in $z$ (the Schmidt and Spitzer Theorem holds as well), the set $\mathbb{C}\setminus\Lambda(b)$ need not be connected as shown, e.g., in Proposition 5.2. in Böttcher, Grudsky, LAA, 2002. So the particular form of $b$ (or the Hessenberg form of $T(b)$), which we assume, is essential.

  2. If $a_{1}=\dots=a_{k-1}=0$, the set $\mathbb{C}\setminus\Lambda(b)$ is know to be connected.

  3. The set $\Lambda(b)$ is always connected, Ullman, Bull. AMS, 1967.

$k=8$, $a=(0,-1,2,3,-5,-4,-3,-3)$

$k=4$, $a=(0,1,2,-7)$

$k=12$, $a=(0,2,-2,-2,-2,-2,-1,-1,-1,-1,-6,-6,-6)$

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

This is more of a longer comment.

Note that your question does not really have anything to do with Toepliz matrices, even thought it is a nice application. In fact, it can be rephrased as a question about boundedness of linear recurrences.

What you are asking is the following:

Let $\lambda \in \mathbb{C}$ be the set of numbers such that the the two smallest roots (magnitude) of $P(z)=\lambda z$ agree in magnitude, where $P$ is a polynomial. Is the complement of this set connected?

Can this question be generalized, perhaps?

Is the corresponding question for $P(z) = \lambda z^k$, $P(z) = \lambda Q(z)$ or perhaps even a polynomial in two variables, $P(z,\lambda)=0$. The latter seems too general to be true though.

Some more computer experiments are welcome!

Also, there is a higher-dimensional analogue of Schmitd and Spitzers statement, and perhaps the corresponding statement holds there?

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ I realize that I need not to mention the relation with Toeplitz matrices. The reason why I did that is: 1. I think it might be useful for a possible proof of the statement. 2. I made the pictures by plotting the eigenvalues of $T_{n}(b)$, with $n=300$. Maybe I could use another title. Of course, one can think about all the generalizations as you mentioned. Nevertheless, I would like to stay at this "simple case of $b$" (or your formulation, $P(z)=\lambda z$) for some time since I have no idea how to solve even this problem. $\endgroup$
    – Twi
    Commented Jun 19, 2016 at 21:54
  • 1
    $\begingroup$ I searched a bit, and I think you might get some more intuition from this article, using pseudo-spectra: people.maths.ox.ac.uk/trefethen/publication/PDF/1992_53.pdf $\endgroup$
    – Per Alexandersson
    Commented Jun 19, 2016 at 23:03
  • 1
    $\begingroup$ Also, I suspect that this is connected to root measures obtained from linear differential operators, see e.g. diva-portal.org/smash/get/diva2:190169/FULLTEXT01.pdf (in particular, page 80 states these are trees), perhaps it is possible to show that one can construct a differential operator with same limit measure as the Toeplitz case? One reason to study Toeplitz matrices is in fact to better understand such differential operators, so it would not be surprising if there is a stronger connection... $\endgroup$
    – Per Alexandersson
    Commented Jun 19, 2016 at 23:34
  • 1
    $\begingroup$ 2Per Alexandersson: Thanks for the interesting references. I read them. I tried to find a differential operator $L$ of fixed order and polynomial coefficients such that the characteristic polynomial $p_{n}$ of a $n\times n$ banded Toeplitz matrix satisfies the eigenvalue equation $Lp_{n}=\lambda_{n}p_{n}$ for all $n$ sufficiently large. However, except the tridiagonal matrix, where the polynomials $p_{n}$ are related to Chebyshev polynomials of the 2nd kind, I found nothing. Now, I doubt that such operator $L$ exists in general (but I have no proof for this claim). $\endgroup$
    – Twi
    Commented Aug 27, 2016 at 20:32
1
$\begingroup$

This is not a complete answer to the question. However, the following example indicates that the curve $\Lambda(b)$ actually can separate the plane $\mathbb{C}$. However, this is just a numerically computed plot and I have no rigorous proof showing this to be a counterexample, indeed.

For the symbol $$b(z)=1/z+z+2 z^2+3 z^3+4 z^4+5 z^5+6 z^6+7 z^7+8 z^8+9 z^9+10 z^{10}$$ the set $\Lambda(b)$ is as follows: enter image description here

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .