I ask here the question since it hasn't been answered in Math Stack Exchange.
I am working through Greenberg and Harper, Lecture notes on Algebraic Topology, and I am having trouble with one exercise. I have spoken with a professor and he encouraged me to ask here or look for it in books since the exercise (which i will insert below) is related to 3-manifold theory, which isn't covered in the book and I have never studied it before. Here it goes what is explained on the book:
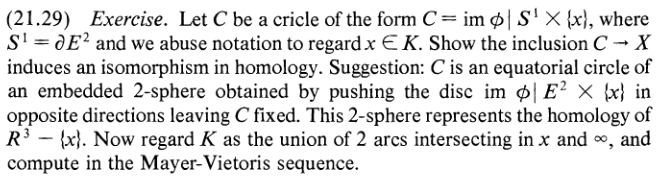
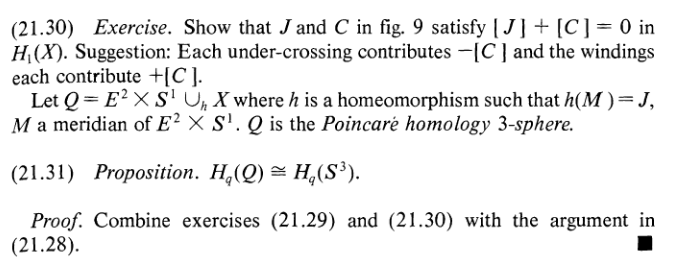
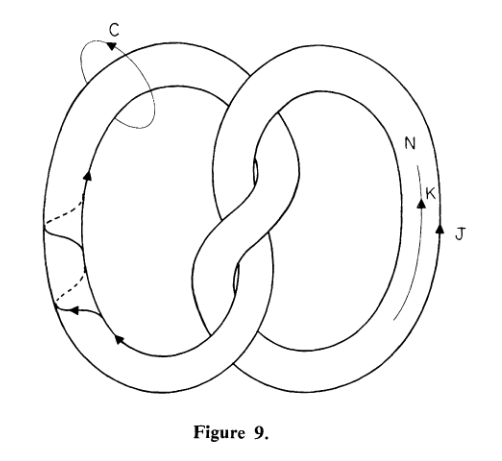
I have managed to solve Exercise 21.29 and to prove Proposition 21.31 in an alternative way using Mayer-Vietoris (with some help: here). My problem is in Exercise 21.30 since I have no idea of how to start or how to deal with it.
In the beginning I thought I could use Exercise 21.29 and see both [C] and [J] as the images of [C] by the isomorphism I showed in Exercise 21.29. And then all I had to do is to show that there are the same number of "windings" that "under-crossings", 2 of each. However, I think this procedure is not rigorous.
I am afraid I can't show no more progress on the problem besides the idea above. I have been thinking for a few days but no more useful ideas have come to my mind.
Any help would be appreciated, even if it consists on pointing me to a book or reference where I can find something related. And please I would really appreciate detailed explanations if you have any idea since this is the first time I deal with something like this.