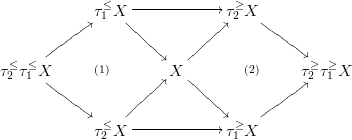
$\def\tee{\mathfrak{t}}$ Let $\tee_1,\tee_2$ be two $t$-structures on a triangulated category $\cal T$; call them fracturing if the two fiber sequences $\tau^\le_1X\to X\to \tau^\ge_1X$ and $\tau^\le_2X\to X\to \tau^\ge_2X$ obtained from the axiom of $t$-structure for an object $X$ arrange into an hexagonal diagram
such that the following conditions hold:
- the two squares (1) and (2) are homotopy pullback-and-pushout;
- the two sequences $\tau_2^\le \tau_1^\le X\to \tau_1^\le X\to \tau_2^\ge X\to \tau_2\tau_1^\ge X$, and the analogous lower one, are fiber sequences (namely $\tau_2\tau_1^\ge X \cong \tau_2^\le \tau_1^\le X[1]$).
I'm looking for at least two different examples of such a situation in nature.