A deformation of a Hopf algebra is defined as follows.
On page 171 of the book a guide to quantum groups, Remark 2, it is said that Any deformation of a Hopf algebra $A$ as a bialgebra is automatically a Hopf algebra.
Now we consider a deformation (mod $h^{n+1}$) of a Hopf algebra $A$. It is defined on page 172 of the book: a guide to quantum groups.
My question is: is a deformation (mod $h^{n+1}$) of a Hopf algebra $A$ as a bialgebra also automatically a Hopf algebra? Thank you very much.
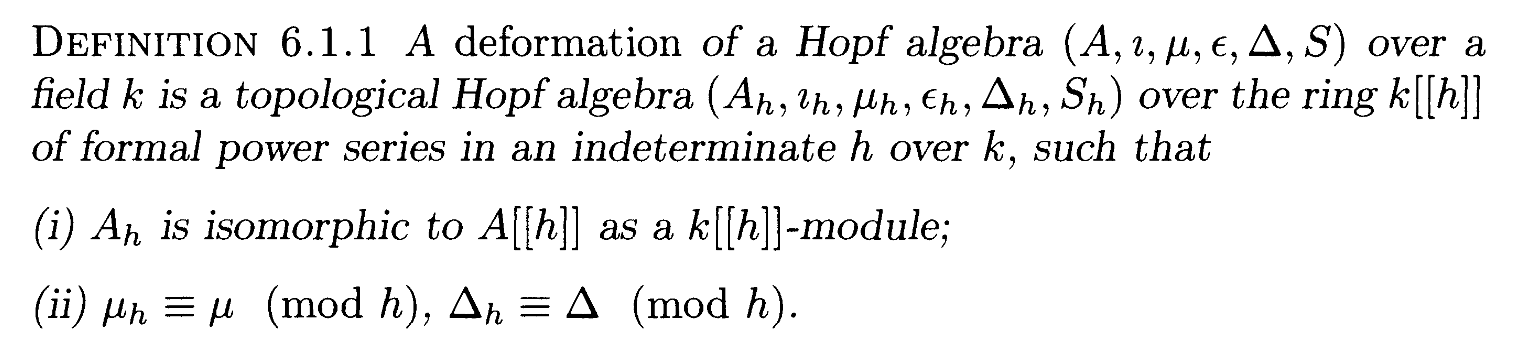
Although I am used to slightly different wording of the definitions I think the deformations mod $h^{n+1}$ will again be Hopf algebra again for the following reason.
If we have any unital algebra $(A,\mu, \eta)$ we can consider a deformation mod $h^{n+1}$ as a product on $A[h]/h^{n+1}A$. By definition this product is given by the sum $\sum_{i=0}^nh^i\mu_i$ for a set $\mu_0,\ldots, \mu_n$ of $k$-linear maps such that $\mu_0=\mu$. Similarly the unit will be given by the sum $\sum_{i=1}^nh^i\eta_i$ of $k$-linear maps such that $\eta_0=\eta$. Suppose that $f\in A$ is an invertible element. Then we have $$ \mu_h(f\otimes f^{-1})=1+\sum_{i=1}^nh^i\mu_i(f\otimes f^{-1}).$$ So we have $$\mu_h(f\otimes \left(f^{-1}+hf^{-1}\eta_1(1)-h\mu_0(f\otimes \mu_1(f\otimes f^{-1}))\right))=$$ $$1+h\eta_1(1)+\sum_{i=2}^nh^i\mu_i(f\otimes f^{-1})+\sum_{i=1}^{n-1}h^{i+1}\mu_i(f\otimes \left(f^{-1}\eta_1(1)-\mu_0(f^{-1}\otimes \mu_1(f\otimes f^{-1})\right))$$
So while $f^{-1}$ is an inverse modulo $h$ we see that $$f^{-1} +hf^{-1}\eta_1(1)-h\mu_0(f^{-1}\otimes \mu_1(f\otimes f^{-1}))$$ is an inverse modulo $h^2$. We can continue like this to obtain an inverse modulo $h^{n+1}$. In other words an inverse in $A[h]/h^{n+1}A$. In fact by a similar procedure we can see that elements of $(A[h]/h^{n+1}A,\mu_h,\eta_h)$ given by $\sum_{i=1}^nh^if_i$ with $f_0$ invertible in $(A,\mu,\eta)$ are invertible.
Now suppose $A$ is a Hopf algebra. This means (by definition) that $\mbox{id} \in Hom_k(A,A)$ is invertible for the convolution product $\star$ given by $$ f\star g=\mu\circ f\otimes g\circ \Delta$$ (the inverse is of course the antipode), the identity for the convolution product is $\eta\circ\epsilon$. The deformation modulo $h^{n+1}$ gives us a deformation of the convolution product. Namely for $f_h=\sum_{i=0}^nh^if_i$ and $g_h=\sum_{i=0}^nh^ig_n$ with $f_i,g_i\in Hom_k(A,A)$ we have $$ f_h\star g_h=\sum_{i,j=0}^nh^{i+j}\mu_h\circ f_i\otimes g_j\circ \Delta_h$$ and this is an associative product on $Hom_k(A,A)[h]/h^{n+1}Hom_k(A,A)$. Then, since $\mbox{id}$ is invertible in $(Hom_k(A,A),\star, \eta\circ\epsilon$, it is also invertible for $\star_h$ product (with unit $\eta_h\circ \epsilon_h$) and the inverse is the antipode.
