What is the fundamental difference between Poisson Point Process and Binomial Point Process?
I am evaluating a solution in a Binomial Point Process setup. If I want to evaluate that in a Poisson Point Process setup, what all issues need to be considered?
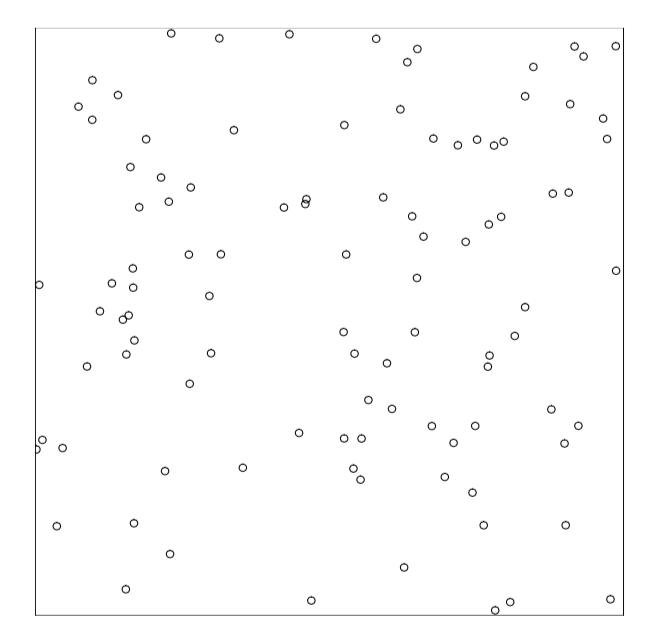
This figure (copied from these notes) serves to illustrate the difference between a binomial and a Poisson point process. Shown are $n=100$ points randomly and independently placed in the unit square. This is a binomial point process, because the distribution of the number of points in an area $W$ inside the unit square is the binomial distribution with $n$ attempts and success probability $p=W$.
The same figure can also be seen as a realization of Poisson point process, given that there are $n=100$ points in the unit square. If this is not given, different realizations of the Poisson process will have different $n$'s, and the distribution of $n$ is the Poisson distribution. This is the only difference between the two processes, the binomial point process has a fixed number of points, while in the Poisson point process this number varies between different realizations.
If $n$ is large enough, the number of points in the unit square for the Poisson process will be peaked around the average of the Poisson distribution, so you might neglect the fluctuations around the average and just fix $n$ at the average value. In that approximation the Poisson and binomial point processes amount to the same thing.
