Tightening a loop

Question

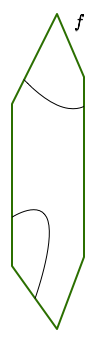
Consider two $d$-dimensional convex polytopes $c_1, c_2$ that share a $(d-1)$-dimensional face $f$. Let $M$ be a surface ($2$-manifold) that intersects each of $c_1$ and $c_2$ in a $2$-ball. Suppose that $f$ is intersected in two $1$-balls; see Figure.

On each side of $f$ the two $1$-balls must connect to a $2$-ball in $c_1$ and $c_2$. Thus either these $2$-balls form a sphere or there must be a tunnel bounded by these $2$-balls. In the case where there is a tunnel, choose a loop $\gamma$ around that tunnel. Can I tighten $\gamma$ so that the resulting loop $\tilde{\gamma}$ lies in a 2-dimensional plane?

Answer 1

The answer is "no".

The intersection $(c_1\cup c_2)\cap M$ might be a thin cylinder $Z$ that lies in a tiny neighborhood of a closed simple curve $\gamma$ in $\partial (c_1\cup c_2)$. We can choose $\gamma$ to be far from being planar. In this case there is no chance to choose a noncontractible plane curve in $Z$.