24
$\begingroup$

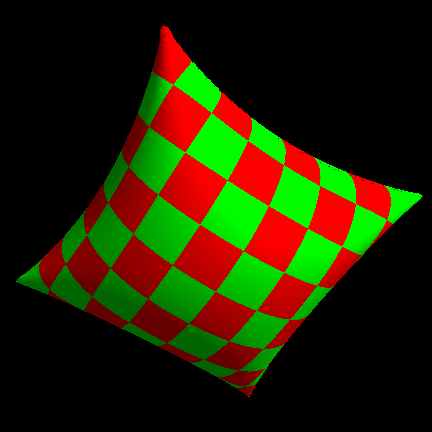
It's obvious that the volume of a envelope is 0 when flat and non-0 when you open it up. However, if you were to fill it with liquid, there must be some shape where it has a maximum volume. Is there a practical way to determine that volume?

I think a starting point would be to start with a cylinder and seal one end by putting it in something like a vice. That gives you the same shape.

EDIT: The reason this problem came to mind is because Quaker's oatmeal packets have a fill line on them so that you don't need a measuring cup. Every day, as I fill up the little packet with water, I think "what a clever idea," but also I wonder about the math behind it. I expect they approximate because, after all, it is just oatmeal.

Improve this question
$\endgroup$
6
  • $\begingroup$ Ok, thank you. It seemed a bit more advanced around here than that I was looking for. $\endgroup$
    – Victor Stone
    Commented Dec 31, 2015 at 20:46
  • 5
    $\begingroup$ Some good problems have elementary formulations. $\endgroup$
    – Douglas Zare
    Commented Dec 31, 2015 at 20:52
  • $\begingroup$ Interesting question, but how is it complex geometry? $\endgroup$
    – Fedor Petrov
    Commented Dec 31, 2015 at 22:41
  • $\begingroup$ @FedorPetrov: I changed the tag to "metric geometry." I think he meant that the geometry was complicated=complex. Meanwhile @ YCor has correctly added the Calculus of Variations tag. $\endgroup$
    – Joseph O'Rourke
    Commented Jan 2, 2016 at 0:40
  • 1
    $\begingroup$ This 2006 preprint by Igor Pak (who has previously been active on MO) is highly relevant: math.ucla.edu/~pak/papers/pillow4.pdf . See also www3.math.tu-berlin.de/geometrie/ps/ddg07/slides/Pak.pdf . $\endgroup$
    – j.c.
    Commented Jan 3, 2016 at 20:01

2 Answers 2

Reset to default
25
$\begingroup$

Your question is a variant of the teabag problem. I don't believe an exact answer is known, but for the $1 \times 1$ square teabag, the maximum volume is about $0.2$:

         
          (Image from Wikipedia article.)

  • The primary reference is Anthony Robin's 2004 article, "Paper Bag Problem". Mathematics Today—Bulletin of the Institute of Mathematics and its Applications. 40.3 (2004): 104-107.

  • MathWorld article on "paper bags", which quotes Robin's 2004 volume bound.

  • $13$-second YouTube Simulation of Inflating a Teabag, using a cloth simulation algorithm.

Improve this answer
$\endgroup$
7
  • $\begingroup$ The volume seems to be irrational as per the Wikipedia article but the surface area can be assumed as rational...the inflated teabag seems to be an interesting solid in this regard. $\endgroup$
    – ARi
    Commented Jan 1, 2016 at 13:14
  • 1
    $\begingroup$ Tangentially related: "Maximum volume convex body coverable by a unit square". $\endgroup$
    – Joseph O'Rourke
    Commented Jan 1, 2016 at 14:22
  • 1
    $\begingroup$ I am missing the differential geometric aspect of the problem, namely that the underlying coordinate transformation must be length-preserving along the "lines" of the coordinate net. As a smooth isometric deformation is not possible (originally the Gauss curvature is zero for both sheets and non-zero afterwards) a true solution would be piecewise linear. I remember having seen an article about such polyhedra in the German version of Scientific American but it will take me some time to unearth that source. $\endgroup$
    – Manfred Weis
    Commented Jan 2, 2016 at 7:16
  • $\begingroup$ Good find, that you @JosephO'Rourke, I had no idea it was quite this complex. It seems that the problem I'm asking about should be the same as if you were to cut the teabag in half. At least that what it seems like based on the image. $\endgroup$
    – Victor Stone
    Commented Jan 3, 2016 at 16:18
  • $\begingroup$ @JosephO'Rourke It has been impossible to find Anthony Robin's work on the web. Do you have a link or name of data base where it can be located ? Thank you. $\endgroup$
    – Daniel Castro
    Commented Jan 22, 2023 at 15:20
5
$\begingroup$

As the envelope is made of paper, a mathematical model of its deformation would be an isometric coordinate transformation and thus must have zero Gauss curvature almost everywhere; that restriction is clearly violated by the cited solution of the teabag problem.

I already pointed out in a comment, that there was an article in the German issue (Spektrum der Wissenschaft, June 1995) of Scientific American, where the topic has been discussed here (in German).
Maybe the same topic has also been treated a few month earlier in Scientific American.

The essential ressource is Edouard Baumann, especially this link (unfortunately in German only); there is also an image of such a polyhedral cushion.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I believe there would be wrinkling creases, as is evident in mylar balloons: Paulsen, William H. "What is the shape of a mylar balloon?" American Mathematical Monthly (1994): 953-958. (Jstor link.) $\endgroup$
    – Joseph O'Rourke
    Commented Jan 3, 2016 at 23:39
  • $\begingroup$ @JosephO'Rourke the solution clearly depends on whether the ballon is made of rubber, aluminum foil or something else; therefore my suggestion, that the proper mathematical be clarified before thinking about a solution. Paper contains inelastic whiskers in every direction; therefore isometric deformations seem appropriate. Linnen pillows covers could be modelled by transformations that take square coordinate grids to rhombic ones; Finsterwalder worked on that topic about a century ago. $\endgroup$
    – Manfred Weis
    Commented Jan 4, 2016 at 4:18

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .