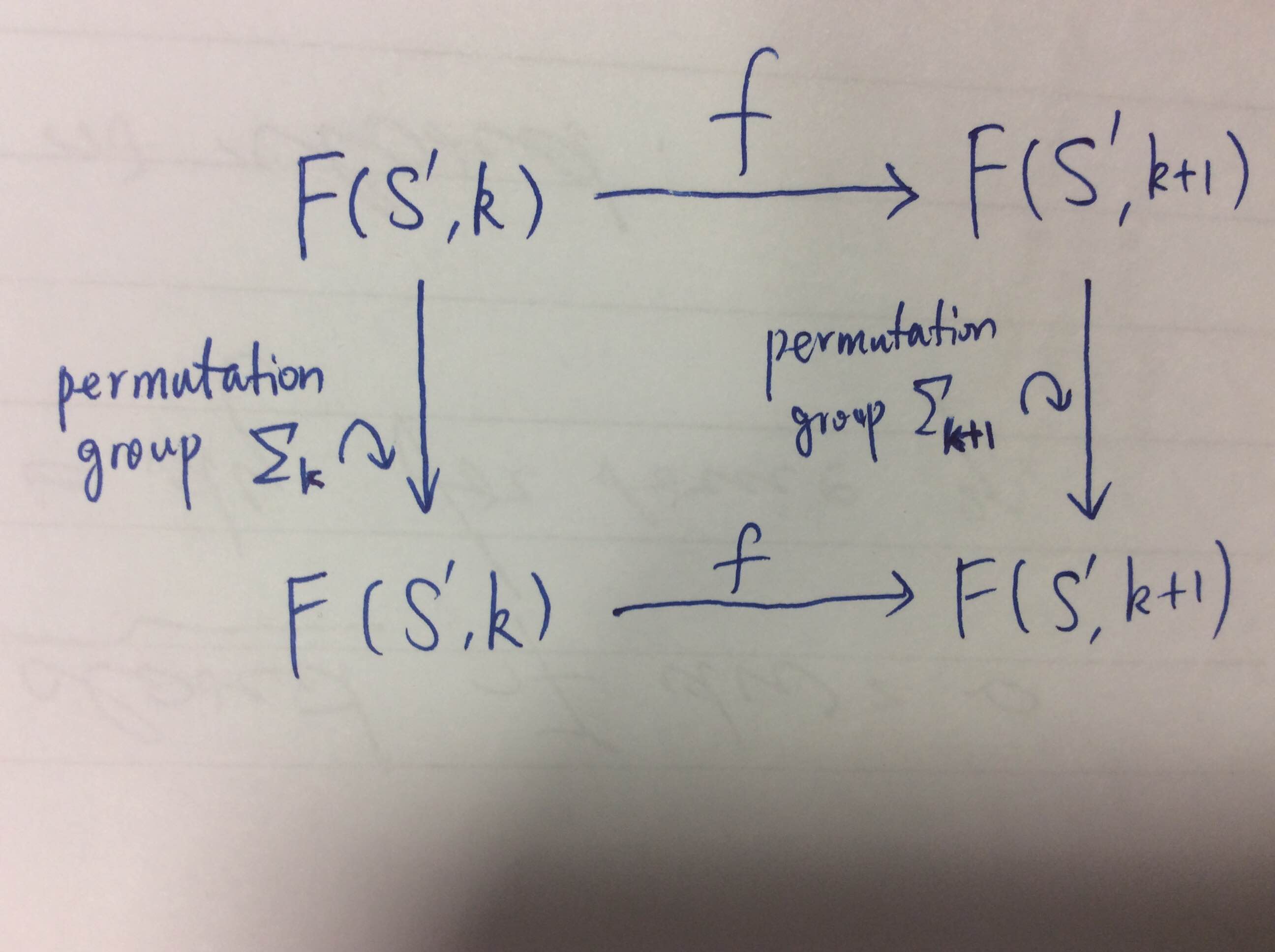
Let $S$ be a closed, orientable surface in $\mathbb{R}^3$ and $S'$ be the manifold $S\setminus\text{one point}$. Let $F(S',k)$ be the $k$-th (ordered) configuration space on $S'$. It is claimed in Cohen-Cohen-Mann-Milgram's paper that
"there is an equivariant embedding $f: F(S',k)\to F(S',k+1)$."
Why? Does this mean the following commutative diagram? What is the map $f$?