Tiling the plane with incongruent isosceles triangles

Question

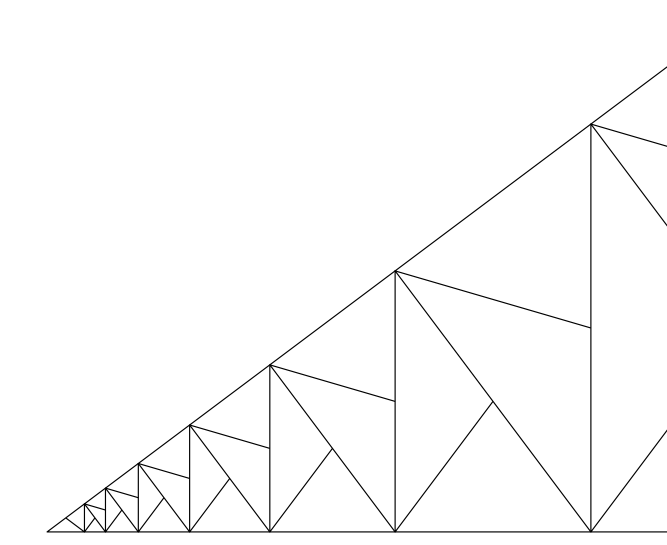
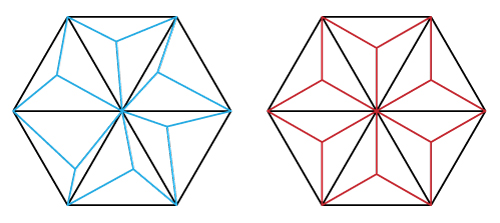
It is not difficult to tile the plane with incongruent triangles. One could tile with equilateral triangles, and then partition each equilateral into three triangles, displacing their common centerpoint so that no two triangles are congruent (left below).

---

         

---

Q1. Is it possible to tile the plane with isosceles triangles, no two of which are congruent?

It is easy to tile the plane with congruent isosceles triangles, as illustrated right above. But I don't see how to achieve a tiling with incongruent isosceles triangles.

Perhaps it is easier to answer this question:

Q2. Is it possible to tile the plane with equilateral triangles, no two of which are congruent?

---

Added 14Feb2020: Q2 has been answered (negatively) in two papers (independently). These results were presaged (by @Wojowu) below.

(1) Pach, János, and Gábor Tardos. "Tiling the plane with equilateral triangles." arXiv:1805.08840 abstract (2018).

Corollary 4. There is no tiling of the plane with pairwise noncongruent equilateral triangles whose side lengths are bounded from below by a positive constant.

(2) Richter, Christian, and Melchior Wirth. "Tilings of convex sets by mutually incongruent equilateral triangles contain arbitrarily small tiles." Discrete & Computational Geometry 63, no. 1 (2020): 169-181. Springer link.

---

Question Q1 was inspired by (but not addressed in) this paper:

Malkevitch, J. "Convex isosceles triangle polyhedra." Geombinatorics 10 (2001): 122-132.

Answer 1

Q1: Yes. Any acute non-isosceles triangle can be tiled by three pairwise incongruent isosceles triangles, by connecting each vertex to the circumcenter. Start from some isosceles $T_0$ with repeated side $s$; inscribe $T_0$ into a larger triangle $T_1$ such that $T_1 - T_0$ is the union of three acute, non-isosceles triangles with circumradii distinct from each other and from $s$; likewise inscribe $T_1$ into $T_2$, and $T_2$ into $T_3$, etc., tiling the complement of $T_0$ with ever-larger acute, non-isosceles triangles with all circumradii pairwise distinct and different from $s$. Now connect each of these triangles' vertices to its circumcenter to obtain a tiling of the plane by isosceles triangles any two of which have distinct repeated sides, and thus a fortiori are not congruent, QEF.

[Joseph O'Rourke asks how to find $T_k$ so that the three components of $T_k-T_{k-1}$ are acute and avoid circmuradius coincidences. One way is to deform the triangle, call it $T'_k$, each of whose sides contains a vertex of $T_{k-1}$ and is parallel to the opposite side of $T_{k-1}$ (so $T_{k-1}$ is the median triangle of $T'_k$). Then each component of $T'_k - T_{k-1}$ is congruent to $T_{k-1}$, and thus acute. Now form $T_k$ by slightly moving each vertex of $T'_k$, keeping all angles acute but removing any coincidences among the circumradii and $s$. While you're at it, you can make sure that none of the angles is $30^\circ$ if you don't accept an equilateral triangle as isosceles.]

Answer 2

Google soon finds that Q2 is problem C11 in Unsolved Problems in Geometry by Croft, Falconer, and Guy. But perhaps it's been solved during the intervening decades.

URL

Answer 3

Simpler construction: a non-isosceles right triangle $T_0$ can be divided into two isosceles triangles not congruent to each other, or into two right triangles similar to $T_0$. Reversing the latter construction, let $T'_n$, $T_n$ ($n = 1, 2, 3, \ldots$) be right triangles similar to $T_0$ such that $T_n = T_{n-1} + T'_n$, and divide $T_0$ and each $T'_n$ into a pair of isosceles triangles. There are two choices at each stage, most of which yield a tiling of the plane; alternatively, choose the $T'_n$ and $T_n$ to all share a vertex with $T_0$, getting a geometric progression [sic] of triangles that tiles a wedge, and then arrange several wedges around a common vertex to fill the plane.

Here's a picture of such a wedge using $(3:4:5)$ triangles:    ^(source)

Answer 4

Illustration of Noam's construction:

---

         

---

Answer 5

Q2: The answer is yes (unless we put some restrictions on tilings, see below), this has been demonstrated in this paper.

In the above construction, there are three points such that any neighbourhood of these contains infinitely many triangles, which might make it not count as a "valid tiling".

One way to exclude such situations is to enforce the triangles' areas to be uniformly bounded away from zero. I don't know of the answer in this case, but if we moreover assume that infimum of triangles' areas is achieved, i.e. there is a minimal triangle, then the answer is known to be no. This is shown in an article of Karl Scherer of very descriptive title "The impossibility of a tesselation of the plane into equilateral triangles whose sidelengths are mutually different, one of them being minimal".