This question has a recreational flavor, but may not be entirely uninteresting.
Let $P_k$ be a unit-radius regular polygon of $k$ sides, and $P_n$ a unit-radius regular polygon of $n \ge k$ sides. Both are origin-centered. Fix $P_k$, and let $P_n$ rotate about its center by angle $\theta$. Define $s(k,n)$ to be the sequence of the number of sides of $P_k \cap P_n(\theta)$ as $\theta$ varies from $0$ to $2\pi$. For example, I believe that $s(4,8)=(\overline{4,8})$, with the overbar indicating repetition, while $s(5,8)=(\overline{10,9})$. Both are illustrated below. (Reload the page to repeat the [limited] animations.)
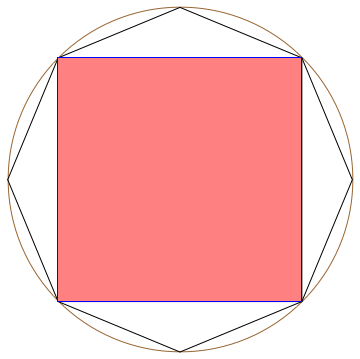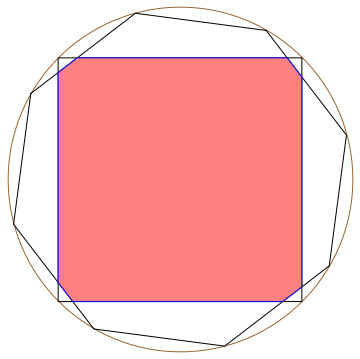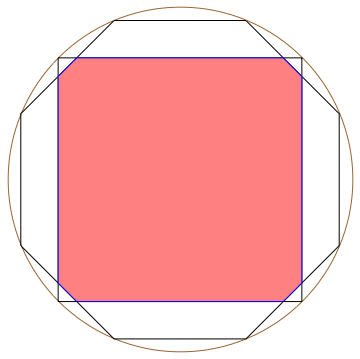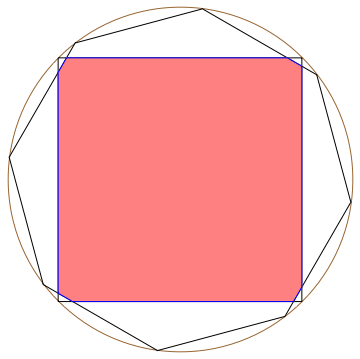
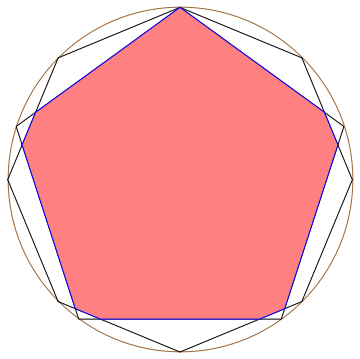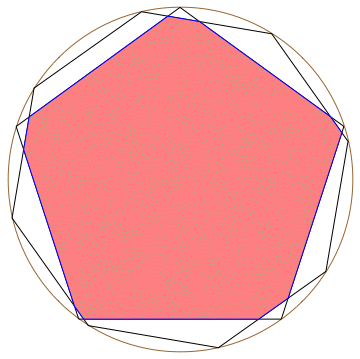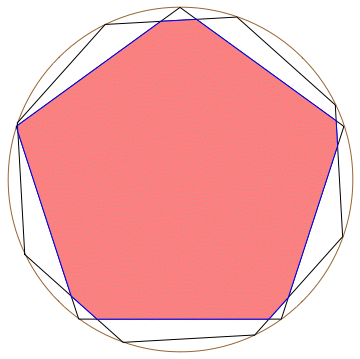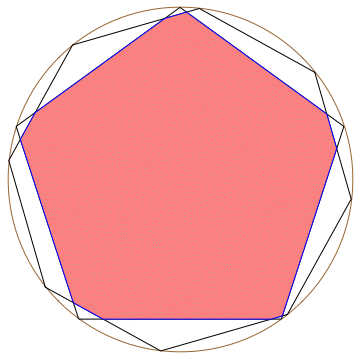
It is an elementary puzzle to
Q. Determine $s(k,n)$ precisely for all $n \ge k$.
I was interested in a higher-dimensional version of this question, but already, although elementary, it seems to need some care to nail down $s(k,n)$ explicitly.
