7
$\begingroup$

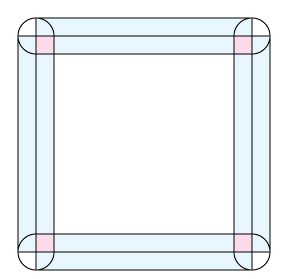
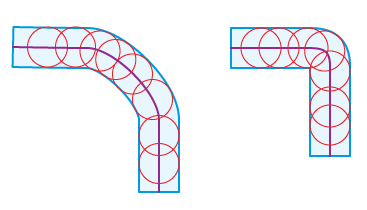
Let $C$ be a closed curve in the plane and let $N_\epsilon(C)$ be an $\epsilon$-neighborhood of $C$, like this: a closed curve with a neighborhood

(ignore the fact that the "curve" is polygonal in this picture, I drew it in MATLAB)

My question is: given fixed $\epsilon$, if we search among all closed curves $C$ of fixed length $\ell$, what is the optimal curve that makes the area of $N_\epsilon(C)$ as large as possible? It seems very intuitive to me that the curve should just be a circle, which gives an area of $2\epsilon\ell$ provided that $\epsilon\leq \ell/(2\pi)$.

Improve this question
$\endgroup$
0

2 Answers 2

Reset to default
6
$\begingroup$

Just to emphasize Thomas Richard's remark about smoothness, unless I've miscalculated, a $\frac{1}{4} L$-square leads to area $$2 \epsilon L - \epsilon^2 (4-\pi) < 2 \epsilon L \;.$$

      Epsilon

Added. This is to illustrate my "hypothesis" in the comments: Any smooth curve (not necessarily convex) such that its radius of curvature exceeds $\epsilon$ (at every point) leads to area $2 \epsilon L$. In the right figure, too-sharp curvature leads to gaps between the $\epsilon$-disk and the tube boundaries.

          EpsilonCurvature

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ But couldn't I approximate this with a smooth curve by rounding the corners by a tiny, tiny amount, and still retain the size of the neighborhood (in other words, does this really have anything to do with smoothness?) $\endgroup$
    – Tom Solberg
    Commented Oct 12, 2015 at 20:19
  • 2
    $\begingroup$ @TomSolberg: Good point. Any sharp turn w.r.t. $\epsilon$ will cause "overlap" of the neighborhood and so lose area. I believe the radius of curvature should exceed $\epsilon$ to achieve $2 \epsilon L$. So that's a hypothesis: Any smooth curve (not necessarily convex) such that its radius of curvature exceeds $\epsilon$ (at every point) leads to area $2 \epsilon L$. $\endgroup$
    – Joseph O'Rourke
    Commented Oct 12, 2015 at 20:24
  • 1
    $\begingroup$ Basically, you need $\varepsilon$ to be smaller than the injectivity radius of the normal exponential map. $\endgroup$
    – Thomas Richard
    Commented Oct 13, 2015 at 5:07
4
$\begingroup$

The area is certainly the same for all smooth convex curves and small $\epsilon$ - your polygonal curve is a good way to see why that might be true. For large $\epsilon,$ it is not clear what the question means...

Improve this answer
$\endgroup$
3
  • 3
    $\begingroup$ I think what's needed is smoothness rather than convexity to get that the area of an $\varepsilon$ neighborhood is $L\times\varepsilon$, where $L$ is the length of the curve, this is the first case of the tube formula. $\endgroup$
    – Thomas Richard
    Commented Oct 12, 2015 at 19:48
  • $\begingroup$ Yes, you are right, will fix. $\endgroup$
    – Igor Rivin
    Commented Oct 12, 2015 at 20:11
  • $\begingroup$ It doesn't need to be convex, but it does need to be a simple closed curve. $\endgroup$
    – Robert Israel
    Commented Oct 12, 2015 at 20:19

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .