28
$\begingroup$

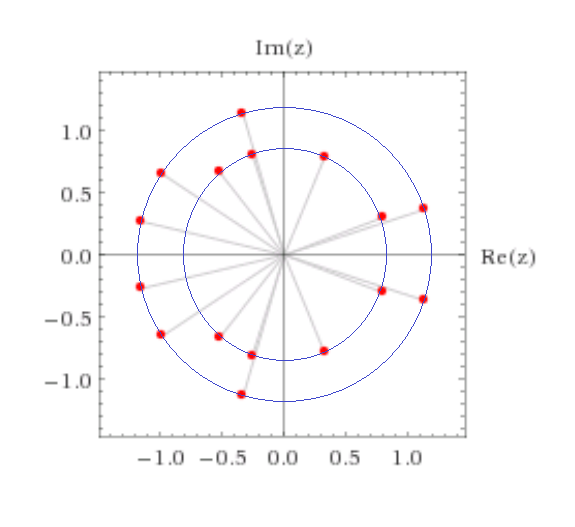
This is somewhat similar to this recent question, but extending in a different direction.

Let $f(x)$ be an irreducible polynomial of degree $n$ with integer coefficients. Call such $f$ a bicycle polynomial if all its roots are located on two circles around $O$, i.e. all roots have one of two moduli. (Of course we'll exclude polynomials of cyclotomic type like $\Phi_n(mx)$ for $m\in\mathbb Z$, which have all their roots fit on one circle.)

For $n=2k$ or $n=3k$, examples of bicycle polynomials are $f(x)=g(x^k-1)$, where $g$ is irreducible of degree $2$ or $3$. Taking here a $g$ of degree $4$ with two pairs of complex roots yields still other bicycle polynomials for $n=4k$.

Now: except replacing the "$-1$" by any other nonzero integer, those types of constructions already seem to be about all of it...

  • Do bicycle polynomials of degree $n$ exist if $n$ has only prime factors $>3$?

Assuming the existence of such a polynomial, it appears (?) to boil down to the existence of a degree $m$ polynomial ($m>3$ odd) with $m-1$ roots on one circle. This circle must presumably have an irrational radius because of what is known about Salem polynomials, but I'm stuck here.

Also related: How to best distribute points on two concentric circles?

Another question:

  • Does anything change if we allow complex integers as coefficients?
Improve this question
$\endgroup$
2
  • 3
    $\begingroup$ There are many more examples than the cyclotomic ones that have all roots lying on one circle. In particular, for $\alpha \neq 0$ any element in the union $\mathbb{Q}^{\mathrm{ab}}$ of all cyclotomic fields, the minimum polynomial of $\xi := \bar{\alpha} / \alpha$ will have all its roots on the unit circle; and in general, such a $\xi$ is not a root of unity. Of course, such polynomials have no real roots and hence they all have even degree. But they give other constructions of bicycle polynomials: if $\beta$ is a root of a bicycle polynomial, then so is $\xi \beta$, for any $\xi$ as above. $\endgroup$
    – Vesselin Dimitrov
    Commented Oct 5, 2015 at 3:52
  • 2
    $\begingroup$ A wide class of polynomials with all roots on the unit circle is given by the Lee-Yang Circle Theorem. See arxiv.org/pdf/1201.3169v1.pdf. Incidentally, the theorem is named after the two physicists who won a Nobel Prize for the overthrow of parity. $\endgroup$
    – Richard Stanley
    Commented Oct 5, 2015 at 23:32

2 Answers 2

Reset to default
20
$\begingroup$

Dubickas and Smyth (On the Remak Height, the Mahler Measure and Conjugate Sets of Algebraic Numbers Lying on Two Circles, 2001) discuss what they call extended Salem numbers.

Moreover, they present results in the direction about which you ask, i.e., conditions under which certain conjugates all fall on two (not one) circles, thereby forcing their associated minimal polynomial to have its degree divisible by either 3 or 2 in parts (a) and (b), respectively.

enter image description here

In the link above, you can find a discussion of extended Salem Numbers and more.

Improve this answer
$\endgroup$
2
  • $\begingroup$ @Wolfgang I wondered how I could've gotten an up-vote at MO 114745 (your Salem Polynomials link -- to a question from Nov. 2012) and it led me to this post under Linked. So I thought I'd try to hunt down something of relevance. I'm glad you found it helpful! $\endgroup$
    – Benjamin Dickman
    Commented Oct 5, 2015 at 6:58
  • 2
    $\begingroup$ There you go. So nobody should ever say that "old" posts are useless.:-) $\endgroup$
    – Wolfgang
    Commented Oct 5, 2015 at 7:30
11
$\begingroup$

I couldn't resist to include a picture showing how asymmetric the distribution of roots can be for a bicycle polynomial. Here are the roots of $$z^{16}+2 z^{15}+z^{14}-2 z^{13}-4 z^{12}-2 z^{11}+3 z^{10}\\ +5 z^9+3 z^8+z^7-z^5-z^4-z^3+z^2+z+1,$$ which is an example of an extended Salem polynomial given by Dubickas and Smyth in their paper.enter image description here

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .