let $g_{n,\gamma}(\sigma)$ be the function defined as the following $$ g_{n,\gamma}(\sigma)= \left(\frac{(\sigma-1)^2 +\gamma^2}{\sigma^2 +\gamma^2} \right)^{n/2} T_n\left( \frac{\sigma(\sigma-1) +\gamma^2}{\sigma^2 +\gamma^2}\right). $$ Where $T_n$ is the Tchebychev polynomial of first kind and degree $n,$ $\sigma \in ]0,1[$ and $\gamma >0.$ Is this function concave or convex i.e have we $g_{n,\gamma}(\sigma) + g_{n,\gamma}(1-\sigma)\leq 2 g_{n,\gamma}(1/2)$? Many thanks, Khadija
$\begingroup$
$\endgroup$
1
-
1$\begingroup$ It is easy to see by plotting that $g_{n,\gamma}$ is neither concave nor convex for most values of $n$ and $\gamma$. The main point is that the graph of $T_n$ is too wiggly. Anyway, this question would be better at math.stackexchange.com $\endgroup$– Neil StricklandCommented Sep 17, 2015 at 12:18
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
3
Maple says $g_{5,1}(\sigma)$ is neither convex nor concave for $\sigma \in ]0,1]$:
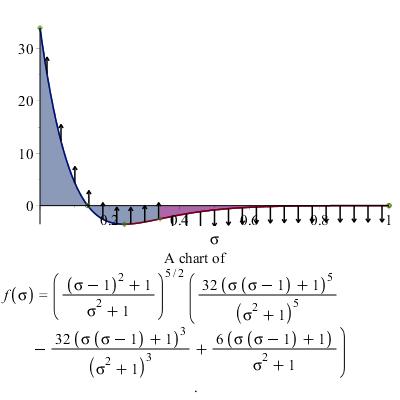
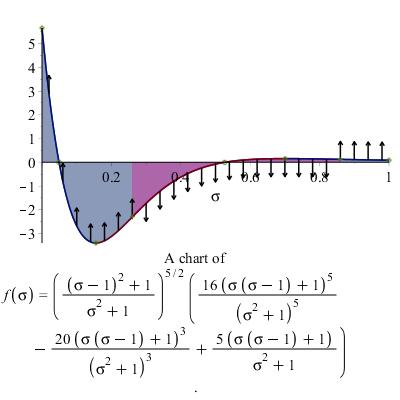
restart; with(orthopoly): Student[Calculus1]:-FunctionPlot((((sigma-1)^2+1)/(sigma^2+1))^(5/2)*U(5, ( sigma * (sigma-1)+1)/(sigma^2+1)), sigma = 0 .. 1);
See orthopoly and FunctionPlot for info.
Addition. The same with the ones of the first kind:
restart; with(orthopoly): Student[Calculus1]:-FunctionPlot((((sigma-1)^2+1)/(sigma^2+1))^(5/2)*T(5, (sigma *(sigma-1)+1)/(sigma^2+1)), sigma = 0 .. 1);
-
$\begingroup$ Thanks alot @user64494. Is it the same thing if $T_n$ is the Chebychev polynomial of first kind? $\endgroup$ Commented Sep 16, 2015 at 20:19
-
$\begingroup$ @ Khadija Mbarki : Yes, it is. See addition to my answer. $\endgroup$ Commented Sep 17, 2015 at 7:09
-
$\begingroup$ Could you check @user64494 if this identity holds true $$g_{n,\gamma}(\sigma) + g_{n,\gamma}(1-\sigma)= 2 g_{n,\gamma}(1/2)$$ holds true if and only if $\sigma=1/2$? $\endgroup$ Commented Oct 8, 2015 at 6:33