Minimal model (resolution) for a specific colored operad

Question

We know that for the operad $As:=\mathcal{F}(\mu)/(\mu\circ_1\mu-\mu\circ_2\mu)$, its minimal model is the free operad $\mathcal{F}(E)$ where $E=\mathbb{k}\langle\mu_2,\mu_3,\dots,\mu_n,\dots\rangle$ is the collection of $\mathbb{k}$-modules spanned by $\mu_n$ in arity $n$ and degree $|\mu_n|=n-2$.(Ref: http://arxiv.org/abs/math/0103052)

My question here is about colored operad:

Let $C$ be the set of rectangles of integral dimensions (width and length are natural numbers). Consider $C$ as the set of colors, we define $C$-operad $P$ in the category of modules over a fix base ring $\mathbb{k}$ as follow: $ P\pmatrix{c\\c_1,\dots,c_n}=\mathbb{k},\ \ \text{ if the rectangle } c \text{ is the disjoint union of rectangles } c_1,\dots,c_n $ ; and $P\pmatrix{c\\c_1,\dots,c_n}=0$ elsewhere.

Can we construct an explicit minimal model for this colored operad? Please help.

Answer 1

This is likely to be difficult. One feature of $As$ that makes it possible for an explicit small minimal model is a simple generators and relations picture; that is, there is one generator $\mu$ and one relation, which is quadratic in the generators.

Of course, there are infinitely many colors, but that would be no big deal if the generators and relations were "locally small" in some sense. That is, if your operad were generated by operations like this:

subject only to horizontal associativity relations for pictures like this:

then you would have some hope of having a description similar in nature to that for $As$.

On the other hand, if your operad were less rigid you might also be able to do something. That is, one could imagine a continuous set of colors and a larger space of operations, corresponding not only to disjoint unions of rectangles but all rectilinear isometric embeddings of the disjoint unions, then this colored operad would be closely related to the little squares operad (other versions are called the $E_2$ operad and the little disks operad. The algebraic versions of these operads are also fairly well understood, although explicitly writing down the differential for the minimal model is already significantly more complicated than for the associative case.

However, it seems like your situation is much, much worse. Because you're working only with disjoint unions and integral lengths, you have some discrete set; because your space of operations is only $\mathbb{k}$, there will be many, many relations. If there were only a few generators (as in my example above), then you still might have some hope. But there are going to be many, many, generators as well. Consider this picture:

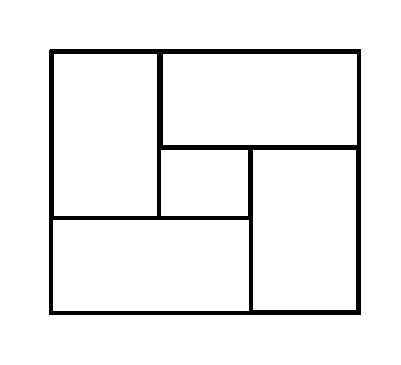
By varying lengths, you'll get many indecomposable 5 to one generators, all of which would need to be represented in the minimal model. Furthermore, by naively iterating this picture with a larger outside width, you get indecomposable $5^k$ to one generators, and that's without doing anything fancier at all; there are many other obvious things you could do to get more complicated indecomposables. So there are certainly countably many indecomposable generators taking the place of the single operation $\mu$ in your example; furthermore the generating relations among these are also countable, replacing the single associativity relation. Furthermore, these relations are extremely ugly, in the sense that they are of arbitrary degree in the generators, and even potentially mixed degree.

Because of all of this, I think it is very unlikely that it will be possible to write anything remotely like a minimal model for this operad down explicitly. I would love to be proven wrong, but I wouldn't get your hopes up.