6
$\begingroup$

Stalling`s end Theorem (a group has more than one end iff it splits over a finite subgroup) and the Seifert-van Kampen Theorem (the fundamental group of a 'decomposable' space is a free amalgamated free product w.r.t the 'pieces' of the space) are well-known theorems. However I often read that they are kind of analogues or that Stalling's Theorem is the 'combinatorial' version or something like that.

I was wondering if this could be made a bit more precise?

I know that there are some major differences. Indeed Stalling's Theorem deals with amalgamated free products and HNN-Extensions over finite subgroups, where the Seifert-van Kampen Theorem 'just' deals with amalgamated free products.

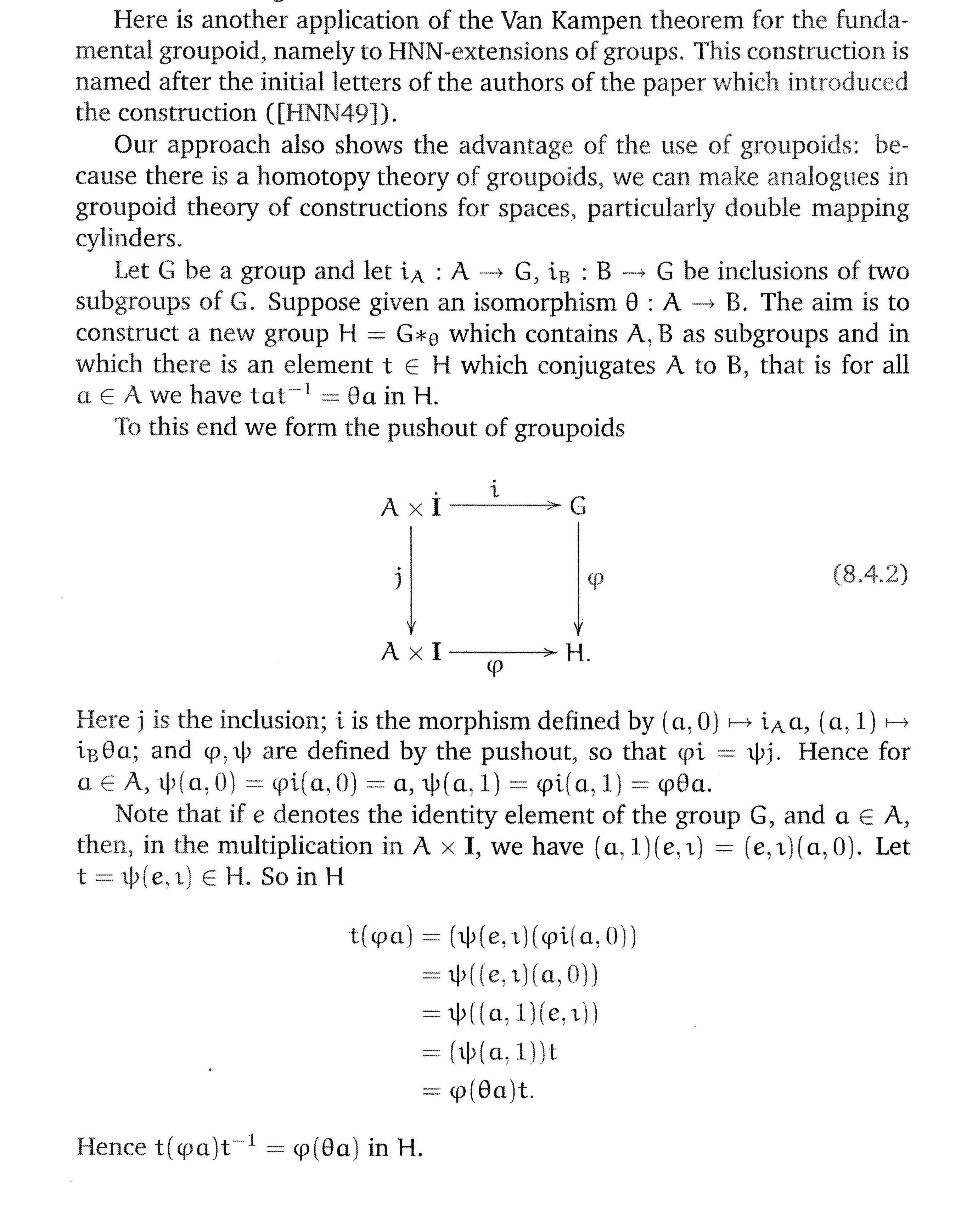
In this sense I was also wondering if there is some sort of 'Seifert-van Kampen Theorem' for HNN-Extensions?

Improve this question
$\endgroup$
6
  • $\begingroup$ I know Bass-Serre theory and Serre's book (and the proof of Dunwoody & Krön for Stalling's Theorem using Bass-Serre trees). However I don't think that this answers my question or I may haven't understood your comment correctly. $\endgroup$
    – M.U.
    Commented Aug 17, 2015 at 11:50
  • 1
    $\begingroup$ I never read that Stallings' and Seifert-van Kampen's theorems were analogous. Have you an example where you read this idea? $\endgroup$
    – Seirios
    Commented Aug 18, 2015 at 4:31
  • 1
    $\begingroup$ Krön, B., Teufl E., Ends, group theoretical and topological aspects or Krön, B., Cutting up graphs revisited - A short proof of Stalling's structure theorem $\endgroup$
    – M.U.
    Commented Aug 18, 2015 at 10:47
  • $\begingroup$ Could you be more specific? I don't currently have the first paper in front of me, but the second does not mention van Kampen's theorem at all. $\endgroup$
    – HJRW
    Commented Dec 18, 2017 at 22:31
  • 1
    $\begingroup$ It's probably not a coincidence that that sentence didn't make it into the published version. I think the real answer to your question is that these two theorems are not usually regarded as analogous. In fifteen years of working in geometric group, I had never heard them described as analogues. Perhaps an e-mail to Kroen would be a better way of finding out what he means. $\endgroup$
    – HJRW
    Commented Dec 20, 2017 at 15:26

1 Answer 1

Reset to default
2
$\begingroup$

Here is an extract from p.335 of Topology and Groupoids (T&G):

(source)

In this $\mathbf I$ is the groupoid with 2 objects $0,1$ and one arrow $\iota:0 \to 1$, and $\dot{\mathbf I}$ is the discrete groupoid on the set consisting of $0,1$. Thus $\mathbf I$ is a "interval object" in the category of groupoids and can be used to model in the category of groupoids topological objects such as double mapping cylinders, as stated in the text. To mirror the topology you need of course a Seifert-van Kampen Theorem for the fundamental groupoid on a set of base points. Compare for example this mathoverflow discussion.

I do not know about applications to the theory of ends! Perhaps old papers of Chris Houghton (see the bibliography of T&G) are relevant.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks. I actually heard a lot about your book but it seems that I really have to take a closer look at it :) $\endgroup$
    – M.U.
    Commented Aug 17, 2015 at 11:31

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .