Is the top interval of a finite distributive lattice, a boolean lattice?

Question

Let $(L,\wedge,\vee)$ be a finite distributive lattice, and let $1$ its greatest element.
An element $a \in L$ is called maximal if $a \le a' < 1$ implies $a = a'$.
Let $b$ be the meet of all the maximal elements, then $[b,1]$ is called the top interval of $L$.
A boolean lattice ($B_n$) is the subsets lattice of a set (of $n$ elements); for example $B_3$ is the following:

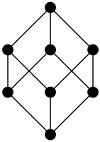
Question: Is the top interval of a finite distributive lattice, a boolean lattice?

Answer 1

Any finite distributive lattice whose smallest element is the meet of its maximal elements is a boolean algebra (= hypercube lattice), so the answer to your question is yes. The proof follows easily, for instance, from the characterization (up to isomorphism) of finite distributive lattices as order ideals of finite posets. See Enumerative Combinatorics, vol. 1, second ed., items a-i on pages 254-255.