1
$\begingroup$

I have asked this question on MathSE and no one could give me an answer. So I'll post my question here.

What I am trying to prove:

A convex polyhedron has positive Gauß-Curvature at every vertex.

What we know:

  • Gauß-Curvature at every vertex is given by $K(p) = 2\pi - \sum\limits_{\text{angle } \alpha_i \text{ around } p} \alpha_i$.
  • Gauß-Bonnet state $\sum\limits_{\text{every vertex }p} K(p) = 2\pi\chi(S)$, where $S$ the convex polyhedron. Therefore we can also write$\sum\limits_{\text{every vertex }p} K(p) = 4\pi$.

My attempt:

I plugged the Gauß-Curvature in the Gauß-Bonnet-Formula and obtained

$$ \sum\limits_{\text{every vertex }p} K(p) = \sum\limits_{\text{every vertex }p} \left(2\pi - \sum\limits_{\text{angle } \alpha_i \text{ around } p} \alpha_i\right) = 4\pi. $$ Assume now that there exists a $\tilde{p}$ such that $2\pi - \sum\limits_{\tilde{p}} \alpha_i < 0$. Then $2\pi < \sum\limits_{\tilde{p}} \alpha_i$...

From here i do not know how to get further on. Does anyone have an alternative of proving this statement or at least can somebody tell me how to complete my proof?

My attempt is wrong, since the Gauß-formula can also applied for non-convex polyhedra!

Can someone complete this proof?

Thanks in advance!

Improve this question
$\endgroup$
8
  • 3
    $\begingroup$ You do not need Gauss Bonnet to prove the evident fact that sum of angles around a vertex of a convex polytope is less than $2\pi$. $\endgroup$
    – Alexandre Eremenko
    Commented Jun 30, 2015 at 13:53
  • $\begingroup$ Do you have an idea how to prove the statement? $\endgroup$
    – aGer
    Commented Jun 30, 2015 at 16:23
  • 3
    $\begingroup$ Pick a plane which touches the vertex by not any other part of the polytope. Push it in slightly. Then you have a new polytope formed by the stuff on the side of the plane containing the point. It has the simple form of a planar polygon and one point lying above the polygon with lines connecting to each vertex of the polygon. Project the vertex to the plane, which is a point in the polygon. The plane vertex has angles that sum to $2\pi$. It is easy to see that each angle at the original vertex is strictly smaller than the projected one in the plane. $\endgroup$
    – Otis Chodosh
    Commented Jun 30, 2015 at 21:25
  • $\begingroup$ @OtisChodosh: Note that if the faces incident to the vertex form a star-shaped polygon on the plane (and the vertex has negative curvature), the original angles are not necessarily smaller than the projected angles. $\endgroup$
    – Joseph O'Rourke
    Commented Jul 1, 2015 at 13:57
  • 2
    $\begingroup$ @OtisChodosh: Example added at tail-end of post. $\endgroup$
    – Joseph O'Rourke
    Commented Jul 2, 2015 at 20:28

1 Answer 1

Reset to default
5
$\begingroup$

Let me try to sketch a proof of "the evident fact that sum of angles around a vertex of a convex polytope is less than $2\pi$," as phrased by Alexandre Eremenko. This proof below is more complex than Otis Chodosh's, but perhaps has the advantage of using convexity explicitly.

Let $v$ be a vertex of the convex polyhedron $P$. Intersect $P$ with a small sphere $S$ centered on $v$. The intersection forms a convex spherical polygon $Q$ (blue below) lying within one hemisphere of $S$. (The hemisphere rim (red below) corresponds to a plane supporting $P$ at $v$.) We can take the radius of $S$ to be $1$ by appropriately scaling $P$ prior to intersecting. Note that the angles of the faces of $P$ incident to $v$ become the arcs of $Q$.

Now I would like to claim that the perimeter of a convex spherical polygon $Q$ lying within a hemisphere of a unit sphere $S$ is at most $2\pi$. I will mimic a proof in a Noam Elkies posting for a planar analog of the claim. The proof is by induction.

Let $Q_0$ be the hemisphere rim; its length is $2\pi$. $Q_0$ contains $Q$: $Q_0 \supseteq Q$. We will form a succession of more tightly enclosing convex spherical polygons, $Q_0 \supseteq Q_1 \supseteq Q_2 \ldots \supseteq Q$, with decreasing perimeters (more accurately: non-increasing perimeters).

Let $k$ be the number of edges of $Q$ that do not lie along the boundary of $Q_i$. If $k=0$, then $Q_i=Q$ and we are finished. Let $e$ be some edge of $Q$ that does not lie on the boundary of $Q_i$. Extend $e$ to a great-circle arc that cuts $Q_i$ at points $a$ and $b$. Define $Q_{i+1}$ as following $Q_i$ but then the "shortcut" $ab$. Because $ab$ is a geodesic, it is a shortest path between $a$ and $b$, and therefore cannot be longer than the portion of $Q_i$ cut off. So the perimeter of $Q_{i+1}$ is at most the perimeter of $Q_i$. Continuing in this manner produces the nested sequence of enclosing spherical polygons, ending with $k=0$.

If no edge of $Q$ lies along the original $Q_0$, then the first cut will be a semicircle (green below), and the perimeter of $Q_1$ is $\pi+\pi$, i.e., still $2 \pi$.

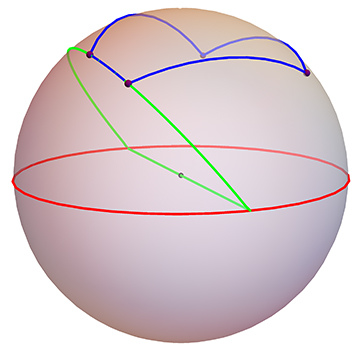
          ConvexSphericalPolygon
So the perimeter of $Q$ is at most $2\pi$, the perimeter of $Q_0$. Therefore the sum of the angles of the faces of $P$ incident to $v$ is at most $2\pi$. Therefore, $P$ has positive angle deficit at $v$, i.e., it has positive Gaussian curvature there.

Note convexity is used crucially, for a nonconvex spherical polygon could have an arbitrarily large perimeter, still fitting inside a hemisphere.

Added (2Jul2015). The example below is intended to show it is not correct to project the angles incident to $v$ to a plane and argue that the angles only enlarge under projection. Below, one angle is $68^\circ$ incident to $v$ in 3D, but that angle projects to $45^\circ$ in 2D.
          ProjectionCex
As you can see, some angles grow, some shrink, under projection.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged .