This answer is complementary to the other answers. I'll attempt to tackle the paradox
That "most of the mass is close to the equator, for any equator" seems almost contradictory (imagining orthogonal equatorial hyperplanes)...
head-on by leveraging some intuition about the 3-sphere.
Where the intuition struggles is in seeing how two $\epsilon$-width equatorial bands oriented in orthogonal directions can have any sort of substantial overlap, much less cover most of the sphere. And they must have substantial overlap: if an equatorial band covers 99% of the area of the sphere, and an orthogonal equatorial band also covers 99% of the sphere, then the overlap of the two bands has to cover at least 98% of the sphere.
The issue is that for the two spheres that are easy to visualize, the 1-sphere and the 2-sphere, the overlap of narrow equatorial bands in orthogonal directions is indeed insubstantial. For the 1-sphere it is, in fact, empty: one equatorial band consists of arcs on the west and east sides of the circe; the other consists of arcs on the south and north sides.
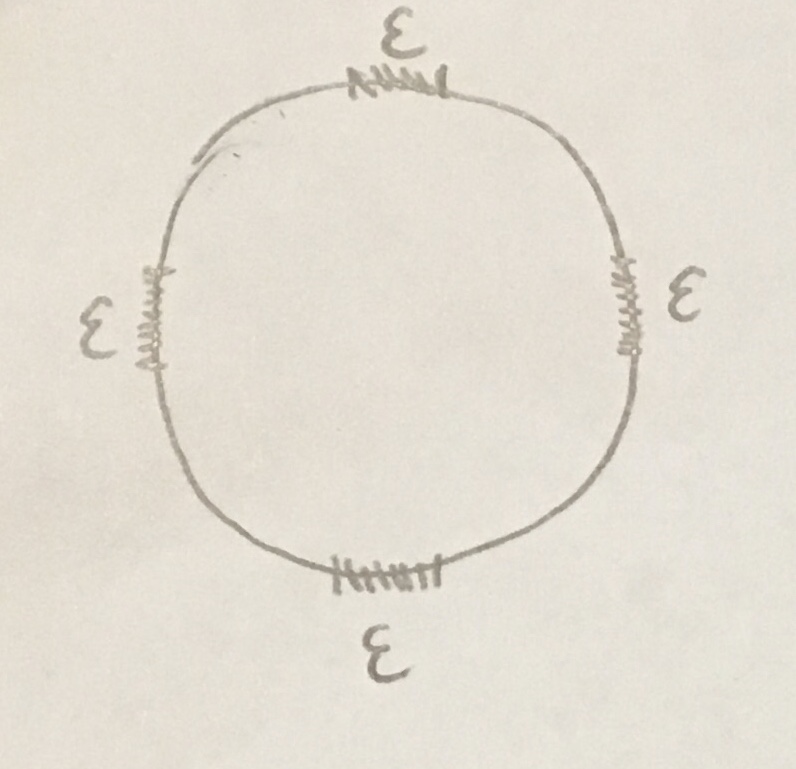
Of course if we increased $\epsilon$ so that each band covered 99% of the area (circumference in this case), then there would be an overlap, of four disconnected arcs, but as a schematic for the situation in higher dimensions this fails since the arcs disappear as $\epsilon$ shrinks.
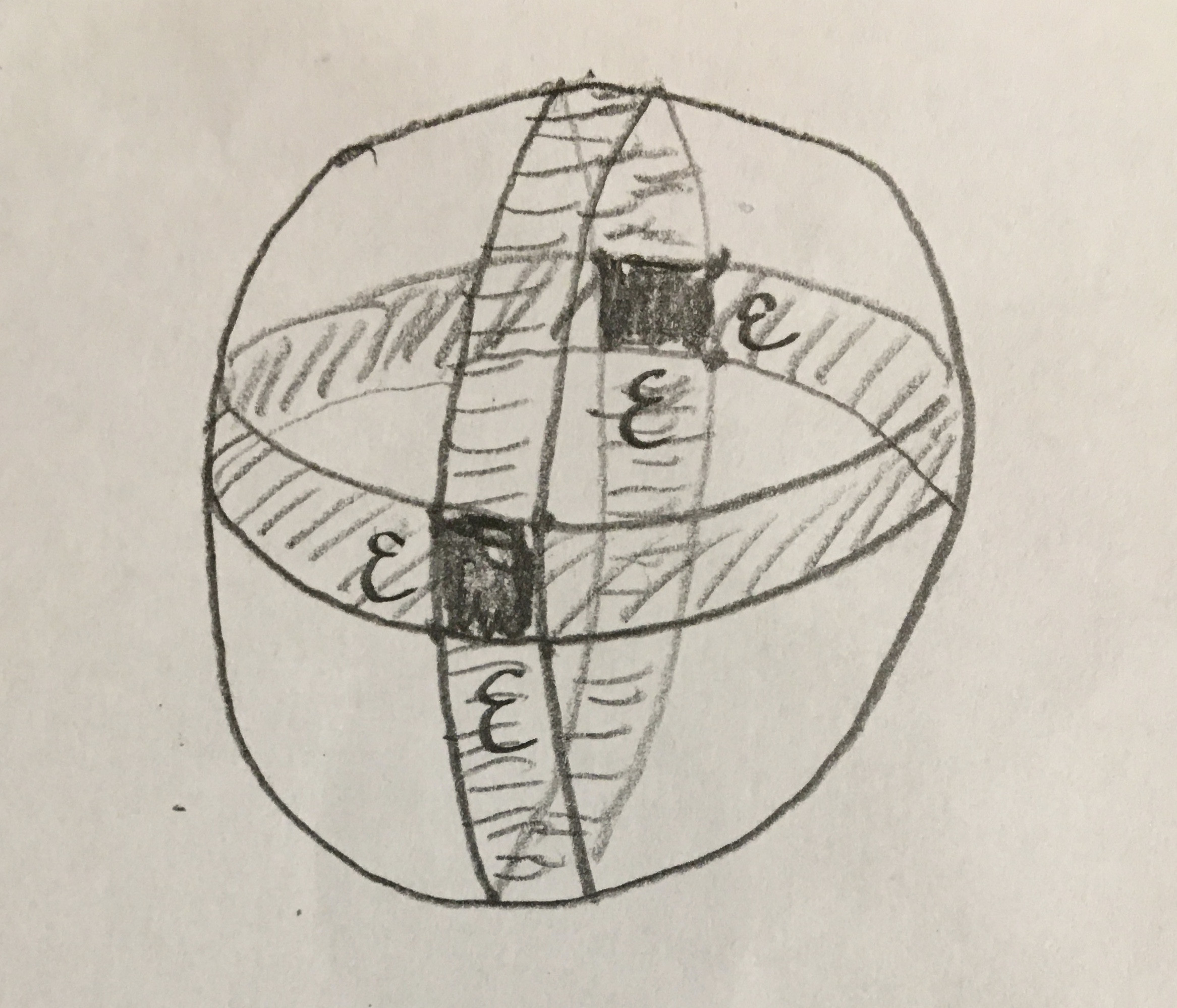
The situation is hardly better for the 2-sphere. The overlap is non-empty this time, but it consists of two antipodal $\epsilon\times\epsilon$ patches (black regions) where the orthogonal belts overlap, and it is hard to imagine, even schematically, how the area of the patches can remain large as $\epsilon$ shrinks.
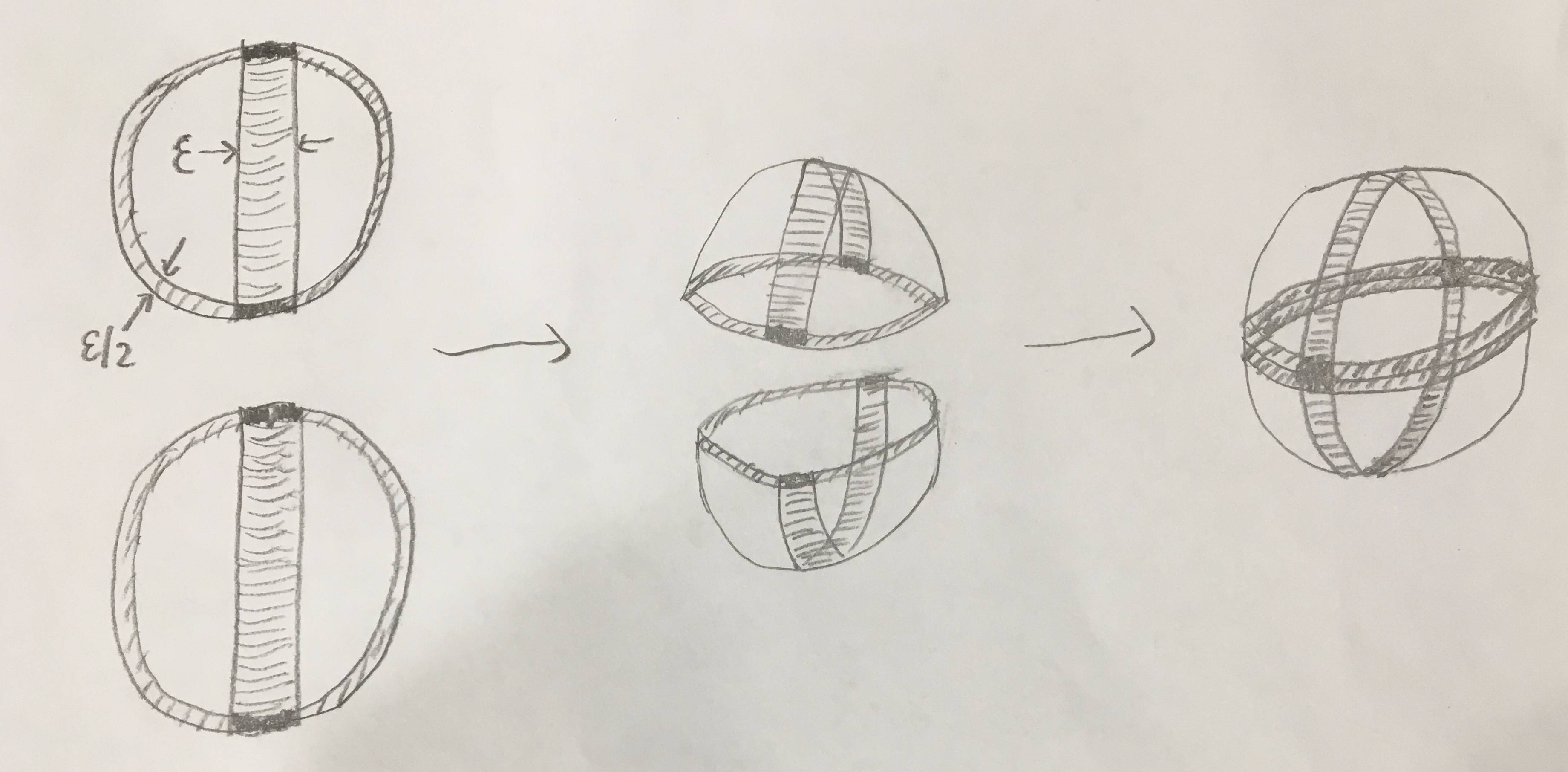
So how do things look for the 3-sphere? A picture of the 3-sphere is obtained by gluing together two 3-dimensional balls along their 2-dimensional surface. Let's redo the 1-sphere and 2-sphere by the analogous constructions. The 1-sphere is obtained by gluing together two 1-dimensional balls (line segments) along their 0-dimensional surfaces (endpoints). The west-east band originates with $\epsilon/2$-width segments at the surface (endpoints) of each line segment; the south-north band originates with an $\epsilon$-width segment spanning the equator (midpoint) of the line segments:

The 2-sphere is obtained by gluing together two 2-dimensional balls (disks) along their 1-dimensional surfaces (circles). One band originates with the $\epsilon/2$-width surface region (annulus) of each disk; the other originates with an $\epsilon$-width equatorial band of each disk:
The 3-sphere is obtained by gluing together two 3-dimensional balls along their spherical surface. One "equatorial band" will be the result of gluing the two spherical shells of thickness $\epsilon/2$ along their outer surfaces to form a spherical shell of thickness $\epsilon$. The other will be the result of gluing two thickened disks of thickness $\epsilon$ along their circumference to form a second spherical shell of thickness $\epsilon$. (One of the thickened disks is shown in the diagram with its center at $\infty$.) The two spherical shells intersect in a band of width $\epsilon$, thickness $\epsilon$, and circumference $2\pi R$, where $R$ is the radius of the balls. (The intersection is what looks like the rim of a bicycle wheel near the center of the last figure.)
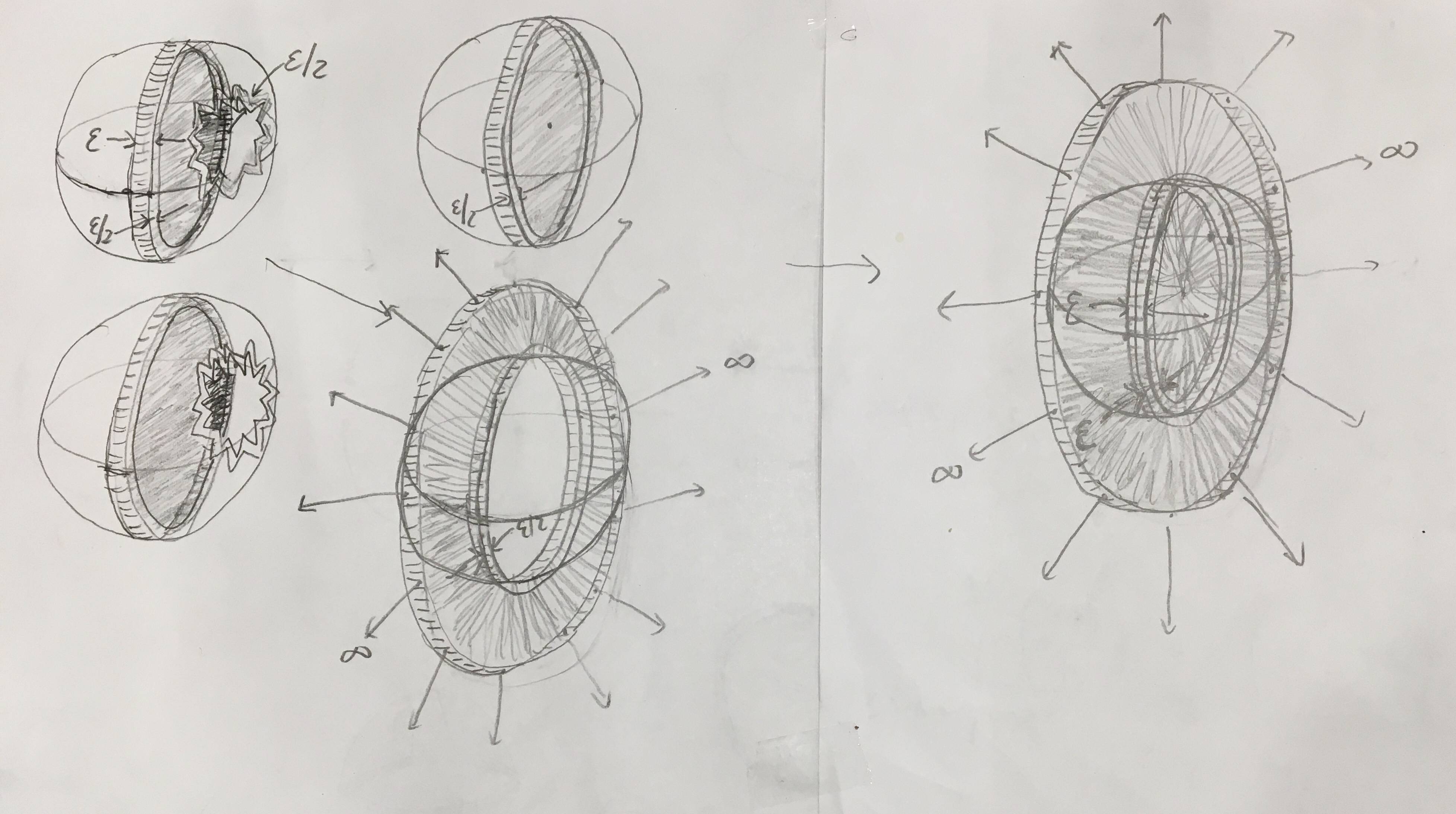
As a schematic for the $n$-sphere, with $n$ large, this picture seems to me successful. As $\epsilon$ shrinks, the overlap remains global in scope, containing a circumference. Furthermore, the picture starts to satisfy some consistency checks: It is easy to believe that most of the volume of an $n$-dimensional ball lies in its outer layer. It's somewhat less obvious but still plausible that most of the volume of an $n$-dimensional ball lies in a thickened disk containing the equator. (Andreas Blass's answer may help convince you of this). For both to be true, most of the volume must lie in a thickened surface band around the equator. That this is so is again plausible: if $n$ is large, then $n-1$ is also large, so most of the volume of the disk lies near its outer edge rather than near its center, that is, in the part where it overlaps the spherical shell; at the same time, it isn't far fetched that in the spherical shell (when $n$ is large) one doesn't lose much by keeping the equatorial region and discarding the caps containing the poles. (This is explained in Andreas Blass's answer.) But this equatorial band containing most of the mass is precisely the overlap of the orthogonal equatorial regions in our 3-sphere construction.





