24
$\begingroup$

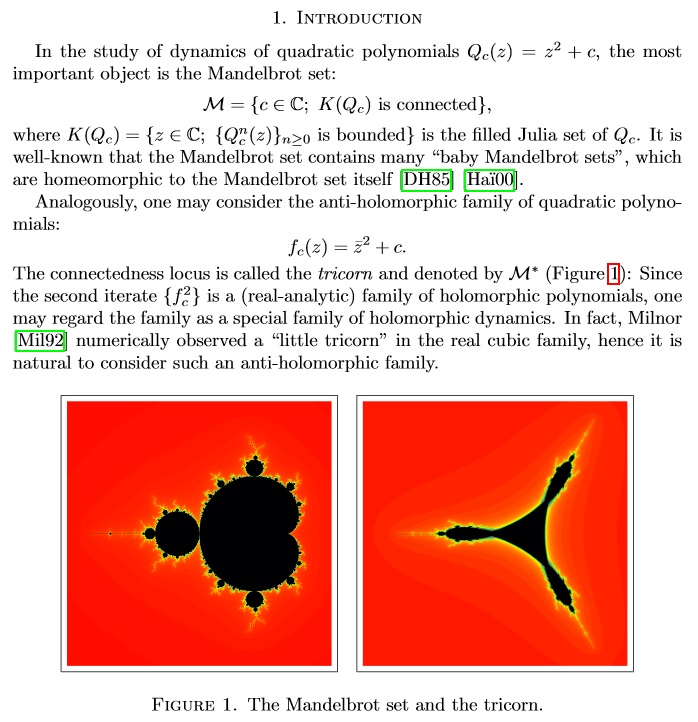
I clearly remember seeing a paper where the dynamic of the anti-conformal map $f(z)=\overline{z}^2+c$ was studied (the bar means complex conjugation). There was a picture of the analog of the Mandelbrot set, which looks very different from the usual one. Can someone help me to locate this paper ?

EDIT. Thanks to Benjamin Dickman. Another common name is Mandelbar, which escaped my memory. Once you know these two names, Tricorn and Mandelbar, the search becomes trivial.

EDIT 2. More information. Apparently this setting was introduced in the paper "On the structure of Mandelbar set by four authors, in Nonlinearity, 2 (1989), 541-553. I contacted one of the authors, and he told me the following story: someone wanted to draw a Mandelbrot set with a computer, but made a misprint in the program. The result looked interesting... Tricorn was discovered by Milnor (independently of this) in his experiments with a holomorphic (cubic) family.

EDIT 3. If someone is curious why I asked this, see the Comment in the end of this preprint: http://arxiv.org/abs/1507.01704

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ Higher-degree (unicritical) versions are sometimes called "multicorns". I believe Dierk Schleicher is among the people who has done some work on the topic (after Milnor). $\endgroup$
    – Lasse Rempe
    Commented Jun 8, 2015 at 8:11
  • 1
    $\begingroup$ Speaking of errors implementing the Mandelbrot fractal, you might like the Burning ship fractal, en.wikipedia.org/wiki/Burning_Ship_fractal $\endgroup$
    – Per Alexandersson
    Commented Sep 2, 2015 at 0:24
  • 1
    $\begingroup$ @Per Alexandersson: Thanks. They are funny. $\endgroup$
    – Alexandre Eremenko
    Commented Sep 2, 2015 at 1:55

1 Answer 1

Reset to default
22
$\begingroup$

Perhaps the key term is tricorn? See Inou's Self-similarity for the tricorn (arXiv pdf) and its references.

Sample excerpt:

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ @AlexandreEremenko You're welcome! I used antiholomorphic and Mandelbrot to find this particular paper; 'Mandelbar' is a pretty good word... $\endgroup$
    – Benjamin Dickman
    Commented Jun 6, 2015 at 7:00

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .