This question loosely builds on the second part of this one.
Take the Riemann $\xi$-function: $\xi(s) =\frac12 s\,(s-1) \,\pi^{-\frac{s}{2}}\, \Gamma\left(\frac{s}{2}\right)\, \zeta(s)$. Numerical evidence suggests that for all $a \in \mathbb{R}$, the zeros of:
$$g(s,a):=\xi(a+s)\pm \xi(a+1-s)$$
all reside on the critical line $\Re(s)=\frac12$.
The graph below illustrates the claim for $-2<a<2$ (and $\pm=+$). Each line shows $\Im(s)$ of a zero for $g(s,a)$ at $\Re(s)=\frac12$. When $a=0$ the zeros are equal to the non-trivial zeros $\rho$ of $\zeta(s)$.
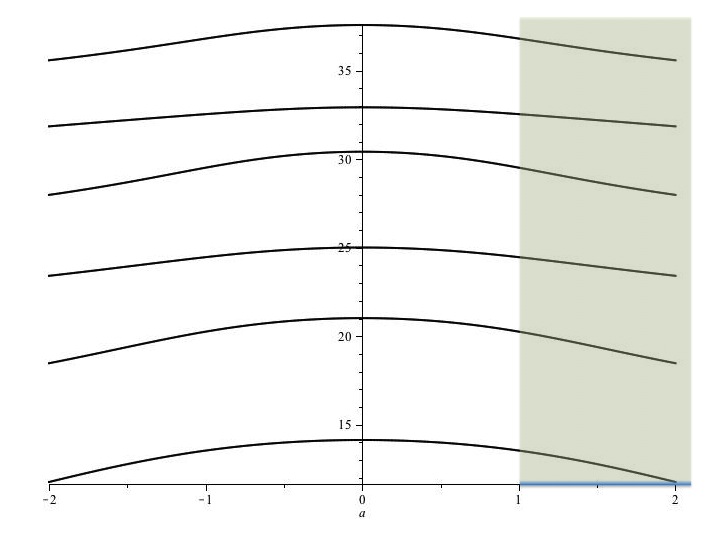
The highlighted part $(a\ge 1)$ shows the domain where $\displaystyle \sum_{n=1}^\infty\frac{1}{n^{a+s}}$ and $\displaystyle \sum_{n=1}^\infty\frac{1}{n^{a+1-s}}$ as well as their Euler products both do converge whilst using values of $s$ with $\Re(s)<1$.
Let's take $a=1$, $\pm=-$ and $0 < \Re(s) <1$, then for each $\xi(1+s) - \xi(2-s)=0$ the following equation should hold:
$$\frac{\zeta(s+1)}{\zeta(2-s)}=\frac{2^s\,\pi^{s-1}\,\cos\left(\frac{\pi\,s}{2}\right)\,\Gamma(3-s)}{s\,(s+1)}$$
or in terms of the primes from the Euler products:
$$\prod_{p=prime} \left( \dfrac{p^{s+1}- p^{2s-1}}{p^{s+1}-1} \right)= \frac{2^s\,\pi^{s-1}\,\cos\left(\frac{\pi\,s}{2}\right)\,\Gamma(3-s)}{s\,(s+1)}$$
Note that the real part of the exponent in the term $p^{2s-1}$ only becomes $0$ when $\Re(s)=\frac12$. It gets negative for $s < \frac12$. I realise this is quite a broad question, but is there anything more to say about the influence of this term on the location of the zeros in the highlighted domain?
Additional note:
The term $p^{2s-1}$ is independent of the choice of $a$; the LHS always is: $\displaystyle \prod_{p=prime} \left( \dfrac{p^{s+a}- p^{2s-1}}{p^{s+a}-1} \right)= yyy$.
