There's an unconventional way to see the 2-skeleton of the ideal triangulation as a union of two immersed ideal pairs of pants (which are always totally geodesic). The image shows one pair, which has a single line of self intersection.
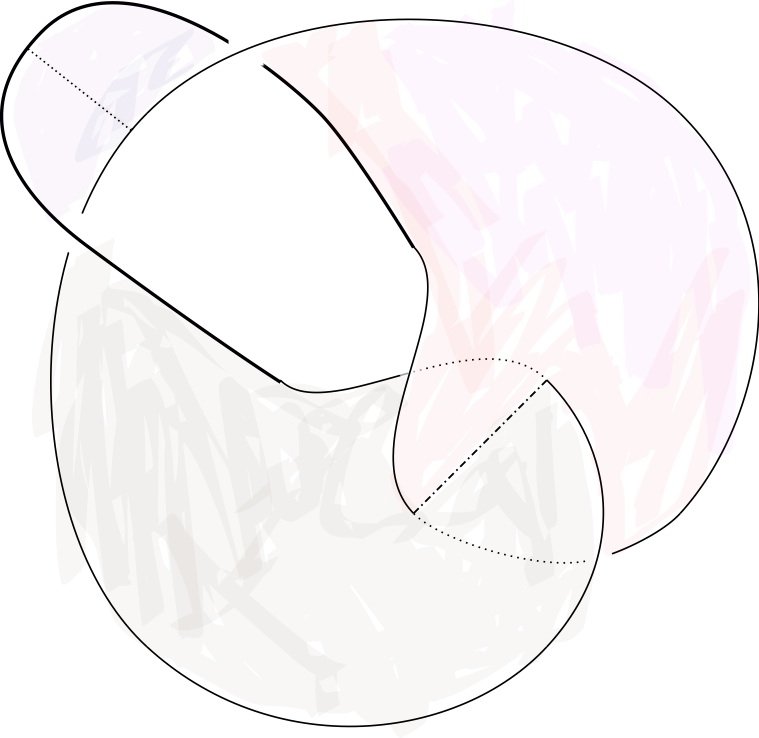
The figure 8 projection has two bigons, which may be arranged symmetrically (considering the projection onto $S^2$), and the other pair of immersed pants has a line of self-intersection along the dotted line in the other bigon. These dotted lines then have 3 branches of the surfaces coming through, and the pants each get divided into two ideal triangles. So the union forms the 2-skeleton of the ideal triangulation, with the two edges having degree 6. Then by the Poincaré polyhedron theorem one concludes that making the two tetrahedra regular ideal tetrahedra with dihedral angles of $\pi/6$ gives a complete hyperbolic structure.
Addendum: This is how George Francis describes the decomposition of the figure eight knot complement. Here are the figures from his Topological Picturebook.