The dominating number $\mathfrak{d}$ and convergent sequences

Question

All spaces considered below are compact Hausdorff.

If $K$ is a space, then $w(K)$ is its weight. For a Boolean algebra $\mathcal{A}$, $K_\mathcal{A}$ denotes its Stone space. I am interested in possible cardinalities of algebras such that their Stone spaces do not have non-trivial convergent sequences. Let me thus define the following cardinal number called (by me) the convergence number:

$\mathfrak{z}=\min\{|\mathcal{A}|:\ K_\mathcal{A}\text{ does not have non-trivial convergent sequences}\}$

($\mathfrak{z}$ from the Polish word "zbieżność" meaning "convergence")

Of course, $\mathfrak{z}$ is not greater than the continuum $\mathfrak{c}$ (consider $\mathcal{A}=\wp(\omega)$).

On the other hand, it is well-known that the splitting number $\mathfrak{s}$ is not greater than $\mathfrak{z}$ -- it follows from the following equivalent definition of $\mathfrak{s}$ due to Booth '74:

$\mathfrak{s}=\min\{w(K):\ K\text{ is not sequentially compact}\}.$

An example of a space $K$ from this definition is $2^\mathfrak{s}$ (which is the Stone space of an algebra).

Also, one can prove (see Geschke '06) that if a space $K$ has weight less than the covering number of category $\text{cov}(\mathcal{M})$, then $K$ must contain a non-trivial convergent sequence, thus $\text{cov}(\mathcal{M})\le\mathfrak{z}$.

It can be shown that the inequalities $\mathfrak{s}<\text{cov}(\mathcal{M})$ and $\text{cov}(\mathcal{M})<\mathfrak{s}$ are relatively consistent (see here). Under Martin's Axiom, all those numbers are equal (to the continuum $\mathfrak{c}$). A natural ZFC simultaneous upper bound of $\mathfrak{s}$ and $\text{cov}(\mathcal{M})$ is the dominating number $\mathfrak{d}$. My question is thus about relations between $\mathfrak{z}$ and $\mathfrak{d}$, especially I am interested in the following:

Question: Is it consistent that $\mathfrak{d}<\mathfrak{z}$ ($<\mathfrak{c}$)?

Recall that the cofinality of measure $\text{cof}(\mathcal{N})$ is not less than $\mathfrak{d}$. If $\kappa$ is a cardinal number such that $\text{cof}([\kappa]^\omega)=\kappa<\mathfrak{c}$, then assuming $\text{cof}(\mathcal{N})=\kappa$ I can construct an example of a Boolean algebra without non-trivial convergent sequences and of cardinality $\kappa$; hence, it is consistent that $\mathfrak{z}\le\text{cof}(\mathcal{N})<\mathfrak{c}$.

Answer 1

First of all, let me say that I love this question.

Alan Dow and I have been thinking about this question and its relatives quite a lot recently. We completed a paper on the topic last week (available on arXiv), and I'll summarize the results here.

As you mention in the comments, the number $\mathfrak{z}$ does not seem to admit a simple combinatorial description, and can be very difficult to work with. Our paper introduces a new cardinal characteristic of the continuum that is closely related to $\mathfrak{z}$ and is "almost" an upper bound for it (in a sense I'll explain below). But the new characteristic has a simple description and is much easier to work with. This allows us to analyze $\mathfrak{z}$ indirectly, by working with a more manageable proxy instead.

Definition: If $U$ and $A$ are infinite sets, we say that $U$ splits $A$ if both $A \cap U$ and $A \setminus U$ are infinite. The splitting number of the reals, denoted $\mathfrak{s}(\mathbb R)$, is the smallest possible cardinality of a collection $\mathcal U$ of open subsets of $\mathbb R$ such that every infinite $A \subseteq \mathbb R$ is split by some $U \in \mathcal U$.

The classical splitting number $\mathfrak{s}$ is the smallest possible cardinality of a collection $\mathcal S$ of subsets of $\mathbb N$ such that every infinite subset of $\mathbb N$ is split by some member of $\mathcal S$. The new number $\mathfrak{s}(\mathbb R)$ is just a topological variant of $\mathfrak{s}$, where instead of splitting subsets of $\mathbb N$ with subsets of $\mathbb N$, we're splitting subsets of $\mathbb R$ with open sets.

I should mention that the value of $\mathfrak{s}(\mathbb R)$ does not change if one replaces $\mathbb R$ with any other uncountable Polish space in the above definition.

Our main theorem relating $\mathfrak{s}(\mathbb R)$ to $\mathfrak{z}$ is the following:

Theorem: If $\mathfrak{s}(\mathbb R) < \aleph_\omega$, then $\mathfrak{z} \leq \mathfrak{s}(\mathbb R)$.

(Actually we have a slightly stronger theorem: if there is a cardinal $\kappa$ such that $\mathfrak{s}(\mathbb{R}) \leq \kappa = \mathrm{cof}(\kappa^{\aleph_0},\subseteq)$, then $\mathfrak{z} \leq \kappa$. It follows that $\mathfrak{z} \leq \mathfrak{s}(\mathbb R)$ whenever $\mathfrak{s}(\mathbb R) < \aleph_\omega$, as stated above, and more: if $\mathfrak{z} > \mathfrak{s}(\mathbb R)$, then either $\mathfrak{s}(\mathbb R)$ has countable cofinality, or there are inner models containing measurable cardinals.)

After proving this theorem relating $\mathfrak{z}$ and $\mathfrak{s}(\mathbb R)$ near the beginning of our paper, we go on to analyze $\mathfrak{s}(\mathbb R)$ in detail. We prove three lower bounds and one upper bound from $\mathsf{ZFC}$:

$\bullet$ $\mathfrak{s},\,\mathrm{cov}(\mathcal M),\,\mathfrak{b} \ \leq \ \mathfrak{s}(\mathbb R)$.

$\bullet$ $\max\{\mathfrak{b},\mathrm{non}(\mathcal N)\} \, \geq \, \mathfrak{s}(\mathbb R)$.

The second bullet point is particularly significant to your question, because it gives an upper bound for $\mathfrak{z}$ also (at least assuming $\mathfrak{s}(\mathbb R) < \aleph_\omega$). In addition to these inequalities, we prove two consistency results via forcing showing that it is possible to have either of

$\bullet$ $\mathfrak{s}(\mathbb R) \,<\, \mathrm{non}(\mathcal N)$

$\bullet$ $\mathfrak{s}(\mathbb R) \,>\, \mathrm{cof}(\mathcal M) = \mathfrak{d}$.

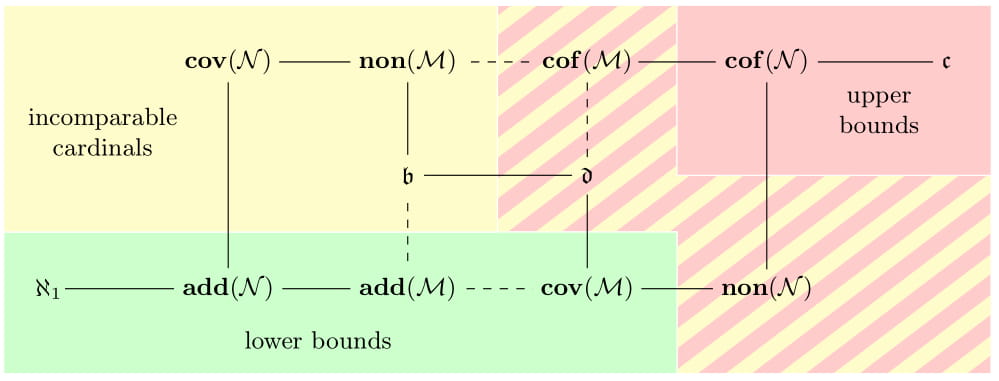
Taken together, these results completely determine the place of $\mathfrak{s}(\mathbb R)$ in Cichoń's diagram:

In this picture, the green cardinals are (consistently strict) lower bounds for $\mathfrak{s}(\mathbb R)$, the red cardinals are (consistently strict) upper bounds, and a carindal $\kappa$ is yellow we know both that both $\kappa < \mathfrak{s}(\mathbb R)$ and $\mathfrak{s}(\mathbb R) < \kappa$ are consistent.

Returning to your question, I am sad to admit that we still do not know whether $\mathfrak{d} < \mathfrak{z}$ is consistent. In our forcing model with $\mathfrak{d} < \mathfrak{s}(\mathbb R)$, we do not know the value of $\mathfrak{z}$, but this model is of course a good candidate for getting $\mathfrak{d} < \mathfrak{z}$. (It is possible though that $\mathfrak{z} < \mathfrak{s}(\mathbb R)$ in this model; we already know that the inequality $\mathfrak{z} < \mathfrak{s}(\mathbb R)$ is consistent because it holds in the Laver model, although a proof of this isn't available yet -- this result will go into a future paper.) Another good candidate we've thought of is a model obtained by adding $\aleph_1$ random reals to a model of $\mathsf{MA}+\neg \mathsf{CH}$, but once again we are not yet able to compute $\mathfrak{z}$ in such a model. I will note that $\mathfrak{d}$ is not a lower bound for $\mathfrak{z}$, because $$\aleph_1 = \mathfrak{z} = \mathfrak{s}(\mathbb R) = \max\{\mathfrak{b},\mathrm{non}(\mathcal N)\} < \mathfrak{d} = \aleph_2$$ in the Miller model.

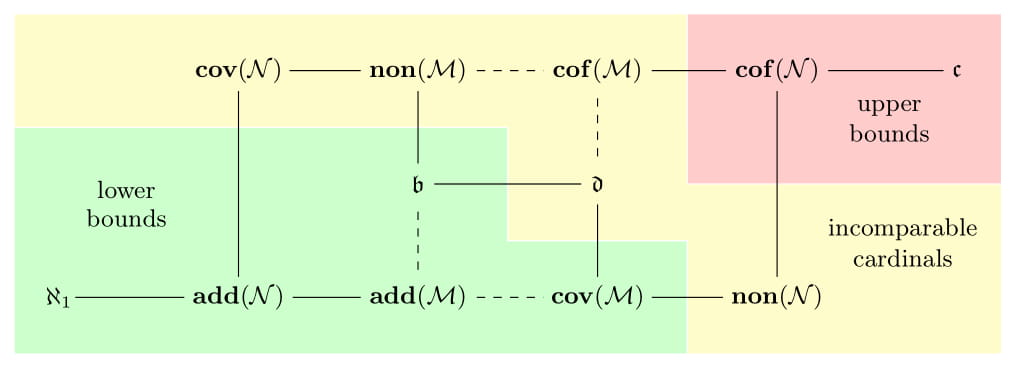
In closing I'll include one more image: it's a picture like the one above for $\mathfrak{s}(\mathbb R)$, but showing what we currently know about $\mathfrak{z}$ instead. The cardinals in the striped region are those that we know are consistently $>\! \mathfrak{z}$, but we don't know yet whether they're consistently $<\! \mathfrak{z}$ as well.