If you break a stick at two points chosen uniformly, the probability the three resulting sticks form a triangle is 1/4. Is there a nice proof of this?

Question

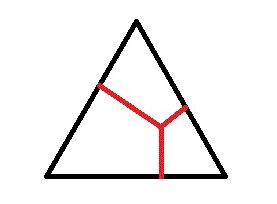
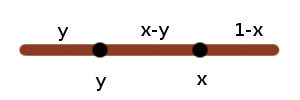
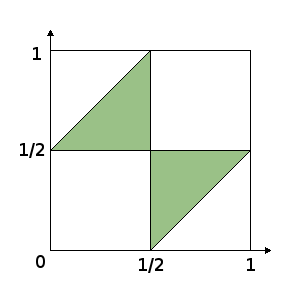
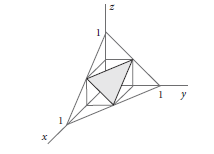
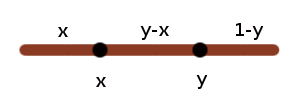There is a standard problem in elementary probability that goes as follows. Consider a stick of length 1. Pick two points uniformly at random on the stick, and break the stick at those points. What is the probability that the three segments obtained in this way form a triangle?

Of course this is the probability that no one of the short sticks is longer than 1/2. This probability turns out to be 1/4. See, for example, problem 5 in these homework solutions (Wayback Machine).

It feels like there should be a nice symmetry-based argument for this answer, but I can't figure it out. I remember seeing once a solution to this problem where the two endpoints of the interval were joined to form a circle, but I can't reconstruct it. Can anybody help?

Answer 1

Here's what seems like the sort of argument you're looking for (based off of a trick Wendel used to compute the probability the convex hull of a set of random points on a sphere contains the center of the sphere, which is really the same question in disguise):

Connect the endpoints of the stick into a circle. We now imagine we're cutting at three points instead of two. We can form a triangle if none of the resulting pieces is at least 1/2, i.e. if no semicircle contains all three of our cut points.

Now imagine our cut as being formed in two stages. In the first stage, we choose three pairs of antipodal points on the circle. In the second, we choose one point from each pair to cut at. The sets of three points lying in a semicircle (the nontriangles) correspond exactly to the sets of three consecutive points out of our six chosen points. This means that 6 out of the possible 8 selections in the second stage lead to a non-triangle, regardless of the pairs of points chosen in the first stage.

Answer 2

Consider an equilateral triangle with altitude 1. It is not hard to show that if you choose a point randomly in this triangle, the distances to the three sides gives the same distribution of lengths that you obtain by breaking a stick at two random points. Now, the locus of points for which no distance is longer than 1/2 is the smaller equilateral triangle formed by joining the midpoints of the edges, which has area 1/4 that of the original triangle.

Answer 3

A triangle is possible iff no part is $>{1\over2}$. With probability ${1\over2}$ both cuts are on the same side of the midpoint $M$, in which case no triangle is possible. If the cuts $x$ and $y$, $\ x < y$, are on different sides of $M$ then with probability ${1\over 2}$ the point $x$ is further left in its half than $y$ is in the right half. In this case there is no triangle possible either. It follows that only ${1\over 4}$ of all cuts admit the forming of a triangle.

Answer 4

Is the argument you remember along the lines of: picking three points on a circle, what is the probability they lie in the same semicircle?

The problem is discussed here:

http://godplaysdice.blogspot.com/2007/10/probabilities-on-circle.html

Answer 5

Yes, here's a nice and beautiful argument!

First you should draw a picture of axes a and b. You're asked to select uniformly a point in the square [0,1]x[0,1]. Now because of the symmetry (sic!) it's equivalent to choosing the points a and b uniformly in the triangle cut from the square by b > a.

So you're actually uniformly selecting a point inside triangle defined by lines a>=0, b<=1, 'b>=a'.

Now let's find the conditions to be able to make a triangle of short sticks. We should have a + (1-b) > b-a, b-a + (1-b) > a and b > 1 - b which indeed, as you say, boils down to

b > 1/2,  a < 1/2,  b-a < 1/2  

It remains to note that those lines create inside the big triangle a small triangle which is similar to big but with all lengths 1/2 of the big, so this small triangle has area of exactly 1/4 of original!

Answer 6

One reference for a solution to this problem is Carlos d'Andrea and Emiliano Gomez, "The broken spaghetti noodle", American Mathematical Monthly 113 (2006), p. 555, JSTOR, author's website (Wayback Machine). More generally the probability that an interval broken at n-1 points chosen uniformly at random is broken into pieces which can be rearranged to form an $n$-gon is $1 - n/2^{n-1}$.

Answer 7

It seems natural to rephrase the question in terms of barycentric coordinates in a triangle. These coordinates are numbers $x$, $y$, $z$ in the interval $[0,1]$ satisfying the equation $x+y+z=1$. We are looking for triples $(x,y,z)$ of such numbers satisfying the three triangle inequalities $x \le y+z$, $y\le x+z$, and $z\le x+y$. Replacing the relations "$\le$" by "$=$", we get line segments joining the midpoints of the edges of the triangle. These line segments cut the triangle into four congruent subtriangles. The central one of these four subtriangles is the region where all three triangle inequalities hold, and this region has area equal to one quarter of the area of the big triangle.

This is essentially the same argument as in the answers by Peter Shor and Ilya Nikokoshev, particularly in the reformulation of the latter answer in Ori Gurel-Gurevich's comment

Answer 8

More analytical option;

Without loss of generality assume that i) the stick is the $[0,1]$ interval, ii) and the first breaking point $x$ is chosen uniformly randomly in $[0,0.5]$. Now for each $x$ the next point $y$ should be in $[0.5,x+0.5]$ to guarantee the triangle. The probability of such choice is $x$. Then one can apply Bayes with $f(x)=2$ and $f(y|x)=x$: $$ \Pr\{\text{Triangle Making} \}=\int_0^{0.5} {2}{x}dx=\frac{1}{4} $$

Answer 9

Here's how I explained it in my blog post a few years ago, as a step in solving a related problem:

If we break the stick at two random points, $x$ and $y$, the three resulting pieces will have lengths $x$, $(y - x)$, and $(1 - y)$ if $x$ is to the left of $y$

and $y$, $(x - y)$, and $(1 - x)$ if $x$ is to the right of $y$

The three pieces form a triangle if none of the pieces is greater than half the length of the stick. In other words, if

$(y > 1/2) AND (x < 1/2) AND (y - x) < 1/2$

when point $x$ is to the left of point $y$ (first image above), and

$(x > 1/2) AND (y < 1/2) AND (x - y) < 1/2$

when $x$ is to the right of $y$ (second image above). If we plot all six of these inequalities we get the area that represents the proportion of triangles formed from our broken stick.

The shaded regions representing the conditions that form a triangle add up to 1/4 of the total area, or a 0.25 probability of forming a triangle.

Answer 10

The problem is indeed choosing uniformly randomly two points $x,y$ on the interval $[0,1]$ such that the length of each sub-interval is less than $\frac{1}{2}$. This is equivalent to probability that two points chosen uniformly randomly on the interval $[0,1]$ fall into the interval $[0,\frac{1}{2}]$ which is $\frac{1}{2}\times\frac{1}{2}=\frac{1}{4}$.

To see this, it is clear that one point, say $x$, should be in $[0,\frac{1}{2}]$ and another one, say $y$, in $[\frac{1}{2},1]$. Now translate $y$ backward by $\frac{1}{2}$ to the point $y'$. Now $y'<x$ for the desired event, i.e., for the case of having the length of each sub-interval less than $\frac{1}{2}$. This means each desired event is equivalent to having two points in $[0,\frac{1}{2}]$ and translate the first one by $\frac12$.

Answer 11

Let AB be the stick. WLOG we may assume AB=1(Since the probability won't depend on the length of AB). Let the points at which the stick is broken be P and Q.

$AP=x$, $PQ=y$ and $QB=z$.

Since $0\leq AP,PQ,QB \leq 1$ we need to consider all the points inside the $1\times 1\times 1 $ cube. Furthermore the points lie on the x+y+z=1 plane.

x+y+z=1 plane(click on the link to see the image of the plane)

On applying the triangle inequalities (i.e $x+y>z,y+z>x\text{ and }x+z>y$) we find that the net area of points satisfying the condition of forming a triangle is the shaded potion.

Shaded Area(Click on the link to see the shaded area)

Since the points $J,K,I$ are the midpoints of the sides of the of the triangle ACE.The probability = $\dfrac{\text{Area of }\Delta JKI}{\text{Area of }\Delta ACE}=\dfrac{1}{4}$

Answer 12

A proof without words that Pr(x < 1/2, y < 1/2, z < 1/2 given that x + y + z = 1) = 1/4 is as follows:

Answer 13

SOLUTION USING ONLY ORIGINAL LINE: Call the end points A and B and the midpoint M. Let N be the midpoint of MB. Call P the first random point and Q the second. In full generality, we can consider P as lying between M and B. On AM, let H be the point for which HP is half the length of the original stick. For the 3 sticks to make a triangle, it is necessary and sufficient that the total length of any 2 sticks be greater than the third. Therefore no piece can exceed half the length of the original stick. That tells us immediately that Q cannot lie on AH or PB, immediately eliminating half the possible points for Q. Likewise all points on MP are also eliminated. Only points on HM qualify as possible Q points. If we let AB=1, the probability that the 3 sticks can form a triangle is then the average length of HM for all Ps. Note that if P=N, the midpoint of MB, then HM=MP, so the qualifying HM points comprise 1/4 the length of the stick and p=1/4 that a triangle can be formed after randomly choosing a Q for this P. Similarly, for every P to the right of N there is a matching P an equal distance to the left of N, so that the average probability (HM length) for these two Ps is 1/4. That yields an overall average probability for all Ps of 1/4.