3
$\begingroup$

I saw in a paper (https://www.princeton.edu/~wbialek/rome/refs/bialek+ruyter_05.pdf Eq.37) the following identity:

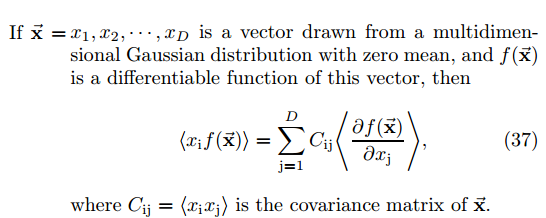
enter image description here

where the <.> operator refers to a population average.

No source or justification was given for this identity & they seemed to assume the reader should be familiar with it.

However, I have no idea where they got this or how to proove it....

Any help please?

Thanks!

Improve this question
$\endgroup$
1
  • 3
    $\begingroup$ Smells like an integration by parts argument, applied to what one gets from multiplying out the LHS. $\endgroup$
    – Yemon Choi
    Commented Mar 24, 2015 at 17:21

2 Answers 2

Reset to default
4
$\begingroup$

indeed, integration by parts does the trick:

$$\int d\mathbf{x}\; \mathbf{x} \,f(\mathbf{x})\exp\left(-\tfrac{1}{2}\mathbf{x}\cdot \mathbf{C}^{-1}\cdot\mathbf{x}\right)=-\int d\mathbf{x}\; \,f(\mathbf{x})\,\mathbf{C}\cdot\frac{\partial}{\partial \mathbf{x}}\exp\left(-\tfrac{1}{2}\mathbf{x}\cdot \mathbf{C}^{-1}\cdot\mathbf{x}\right)$$ $$=\int d\mathbf{x}\; \,\exp\left(-\tfrac{1}{2}\mathbf{x}\cdot \mathbf{C}^{-1}\cdot\mathbf{x}\right)\mathbf{C}\cdot\frac{\partial}{\partial \mathbf{x}}\, f(\mathbf{x})$$ $$\Rightarrow\left\langle\mathbf{x}\,f(\mathbf{x})\right\rangle=\mathbf{C}\cdot\left\langle \frac{\partial}{\partial \mathbf{x}}f(\mathbf{x})\right\rangle$$ where I have used that $\mathbf{C}$ is a symmetric matrix

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thank you Carlo. One question: why does the 'uv' part of integration by parts vanish? (here $u*v=\mathbf{C}f(\mathbf{x})\exp\left(-\tfrac{1}{2}\mathbf{x}\cdot \mathbf{C}^{-1}\cdot\mathbf{x}\right)$) $\endgroup$
    – DankMasterDan
    Commented Mar 24, 2015 at 23:41
  • 1
    $\begingroup$ you have to evaluate this boundary term at infinity, where it vanishes exponentially fast (assuming f(x) does not blow up at infinity, but then the average is not defined) $\endgroup$
    – Carlo Beenakker
    Commented Mar 25, 2015 at 7:14
2
$\begingroup$

This is the famous Stein's identity and indeed integration by parts is how you prove it!

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .