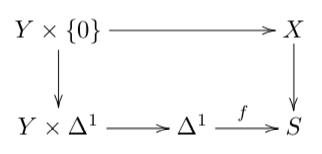
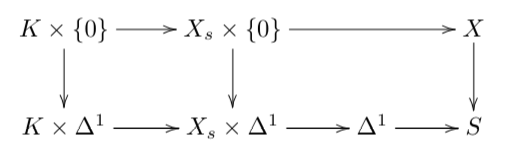I have encountered a problem in understanding Lurie's proof of the following fact:
"Given a left fibration between simplicial sets $q:X \to S$, there exists a functor $$ho(S) \to Ho(sSet)$$ which is defined as follows: a point $s \in S$ is sent to the fiber $X_s$ of $q$ over $s$, and an arrow $f:s \to s'$ in $S'$ is sent to the homotopy class of a lift in
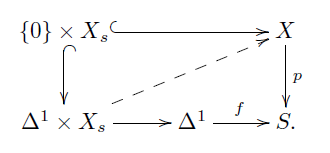
restricted to $\{1\} \times X_s$, which will be denoted by $f_!:X_s \to X_{s'}$".
The author wants to characterize the map $$[f_!]\circ (\cdot): Ho(sSet)(\cdot, X_s) \to Ho(sSet)(\cdot, X_{s'})$$ so as to obtain the desired result (except for the independence from the homotopy class of $f$, which I guess is left to the reader).
The problem is I don't see why it holds that, given a $K\in sSet$ and arrows $\eta:K \to X_s, \ \eta':K \to X_{s'}$, $$\eta'\simeq f_! \circ \eta \iff \exists p:K \times \Delta[1] \to X$$ such that $p_{|K \times \{0\}}\simeq \eta, \ p_{|K \times \{1\}}\simeq \eta'$ and $$q \circ p= K \times \Delta[1] \to \Delta[1] \to S$$ where the last arrow is $f$.
Thanks in advance for any help, which will of course be highly appreciated.