Analytic Solution to SDEs

Question

Are there any example of SDEs with constant diffusion terms, other than the Ornstein Uhlenbeck process, which have exact solutions? I'm thinking of something of the form: \begin{equation} dX_t = f(X_t)dt + a dW_t \end{equation} where $a$ is a constant, and $f$ is some function.

Answer 1

Yes. Unexpected weak solutions to the SDE $$ d Y = - \Phi'(Y) dt + \sqrt{2} dW \quad Y(0) \in \mathbb{R} $$ are available. To see this, transform the associated Fokker-Planck equation into a Schrödinger equation following e.g. Chapter 5 of H. Risken, The Fokker-Planck Equation, Springer-Verlag, 1989. Loosely speaking, if the resulting Schrödinger equation is solvable, then so is the Fokker-Planck equation for the SDE.

For example, in this way one can obtain a formula for the transition probabilities of a Brownian particle in the infinite well potential shown in the figure.

.

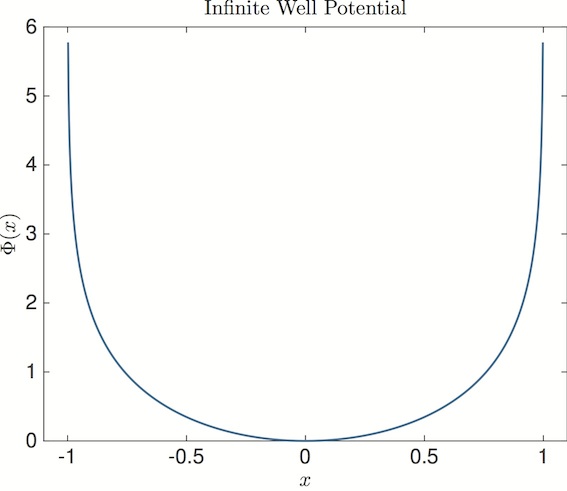For an animation of the transition probability density of the solution click here. The movie starts at a point mass initial condition at $x=0.125$, and evolves the transition density of the process over a time interval that is long enough for the process to relax to its stationary density. For a MATLAB function file which implements this solution click here.

Finally, here is an explicit formula for the transition density of this weak SDE solution: let $p_t(x,y)$ denote the transition probability density of $Y(t)$ given that $Y(0) = x$, i.e., $$ \int_A p_t(x,y) dy = \mathbb{P} ( Y(t) \in A \mid Y(0) = x ) $$ The formula the code uses is taken directly from Risken, and is given by: $$ p_t(x,y) = e^{\Phi(x)/2 - \Phi(y)/2} \sum_{n=0}^{\infty} \psi_n(x) \psi_n(y) e^{-\lambda_n t} $$ where I respectively introduced a potential: $$ \Phi(x) = - 2 \log( \cos(\pi x/2) ) $$ eigenfunctions $$ \psi_n(x) = \begin{cases} \cos((n+1/2) \pi x) & \text{if $n$ is even} \\ \cos(n \pi x) & \text{if $n$ is odd} \end{cases} $$ and corresponding eigenvalues $$ \lambda_n = \begin{cases} \pi^2 (n^2 + n) & \text{if $n$ is even} \\ \pi^2 (n^2-1/4) & \text{if $n$ is odd} \end{cases} $$ I verified this solution numerically using the numerical PDE-based, SDE solver described in Bou-Rabee and Vanden-Eijnden 2015.

Answer 2

Check the seminar book: Numerical Solution of Stochastic Differential Equations P.Kloeden.