I know that a Boolean lattice must be distributive.
But what with these lattices? Are these distributive?
$\hskip0.7in$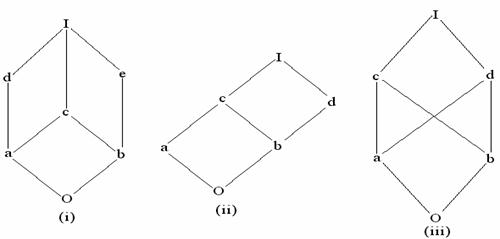
How to recognize which lattices are distributive or not only by looking on their diagrams? Is it even possible?
I know that a Boolean lattice must be distributive.
But what with these lattices? Are these distributive?
$\hskip0.7in$
How to recognize which lattices are distributive or not only by looking on their diagrams? Is it even possible?
There is of course the classic criterion of excluded lattices. A lattice is distributive if does not contain either $M_3$ or $N_5$ (see here for definitions). An easier criterion to check for large lattices is Birkhoff's two chain theorem: if a lattice is generated by two chains, then it is distributive. (The converse is not true.) You can find this in Birkhoff's book Lattice Theory.