Let $\gamma=\gamma(L)$ be a simple (non-self-intersecting) closed curve of length $L$ on the unit-radius sphere $S$. So if $L=2\pi$, $\gamma$ could be a great circle.
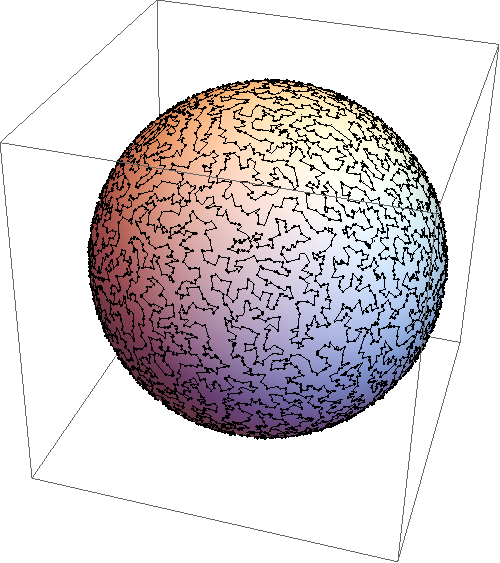
I am seeking the most equitably distributed $\gamma(L)$, distributed in the sense that the length of $\gamma$ within any disk is minimized. This is something like placing repelling electrons on a sphere, but here the curve self-repels. So there should be no "clots" of $\gamma$ anywhere on $S$. I am especially interested in large $L$. A possible $\gamma$ is shown below, surely not optimal for its length:
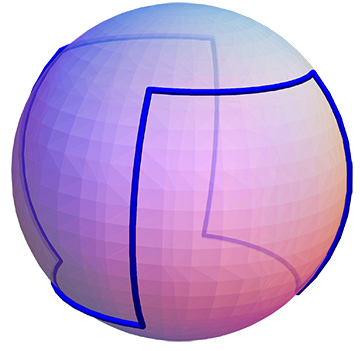
Here is an attempt to capture more formally "equitably distributed." I find this an awkward definition, and perhaps there is a more natural definition.
Around a point $c \in S$, measure the $r$-density of $\gamma$ as the total length within an $r$-disk: $$d_\gamma(c,r) = | \gamma \cap D(c,r)|$$ where $D(c,r)$ is the disk of geodesic radius $r$ centered on $c$. Then define $d_\gamma(r)$ as the maximum of $d_\gamma(c,r)$ over all $c \in S$.
Finally, we can say that, for two curves $\gamma_1$ and $\gamma_2$ of the same length $L$, that $\gamma_1 \le \gamma_2$ if $d_{\gamma_1}(r) \le d_{\gamma_2}(r)$ for all $r \in (0,\pi)$, i.e., $\gamma_1$ is less concentrated than $\gamma_2$ for all $r$ up to a hemisphere.
This definition provides a partial order on curves of a given length $L$. One version of my question is:
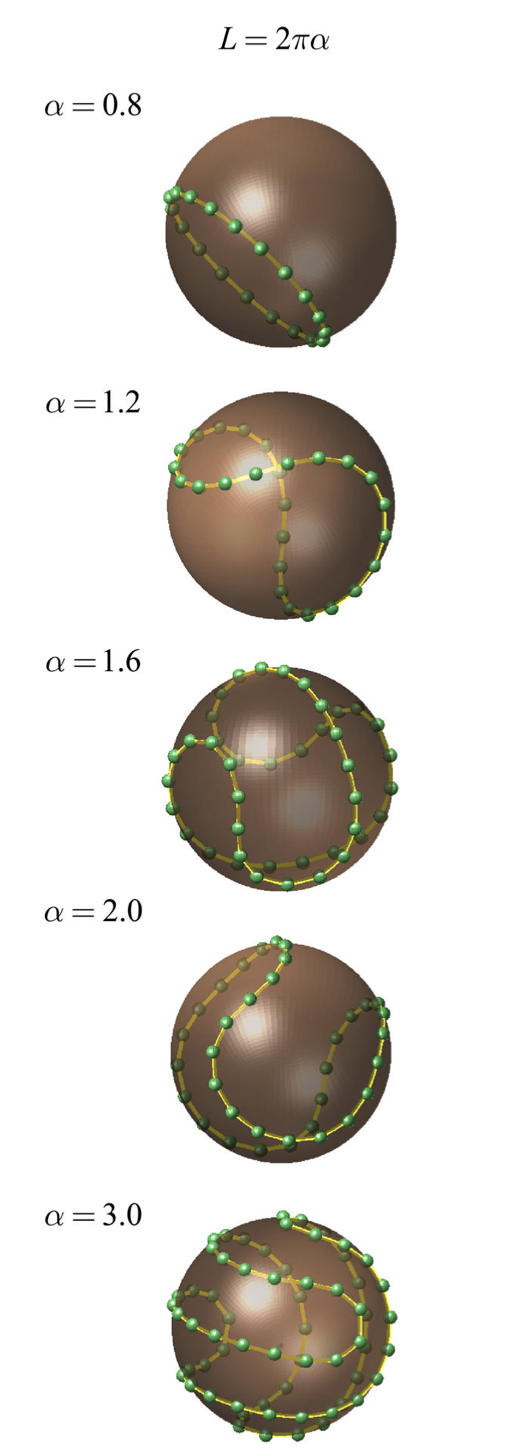
Q. What do the minimal elements of this poset look like, especially as $L$ gets large?
These minimal curves are in some sense nowhere densely clotted.
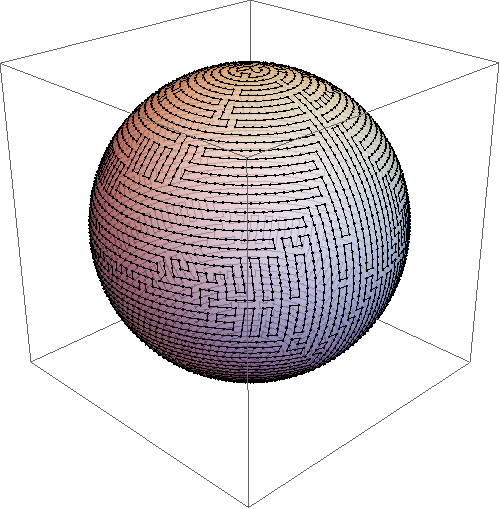
Update. Acknowledging Gerhard Paseman's remark, I thought I would include this attractive image of a space-filling curve on a sphere:

(Image from this website).
But notice it is certainly not equidistributed in any sense, crowding near the northpole.