54
$\begingroup$

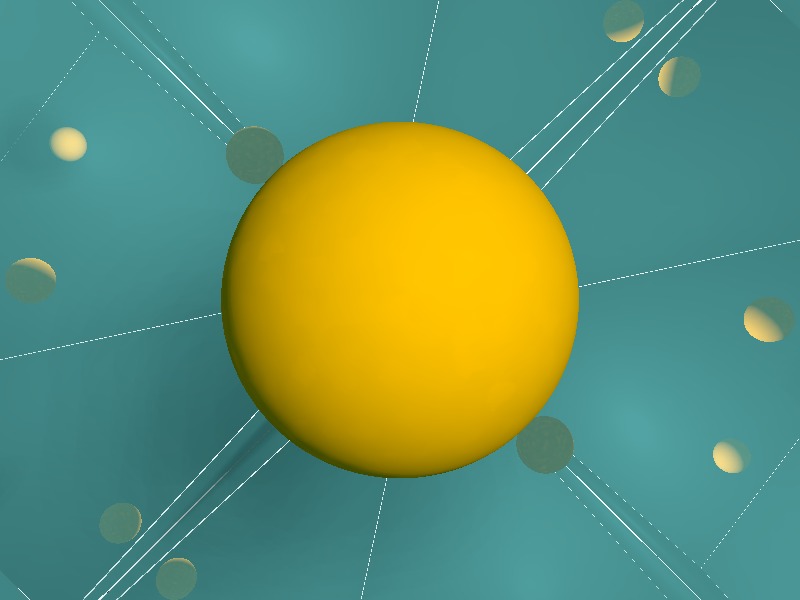
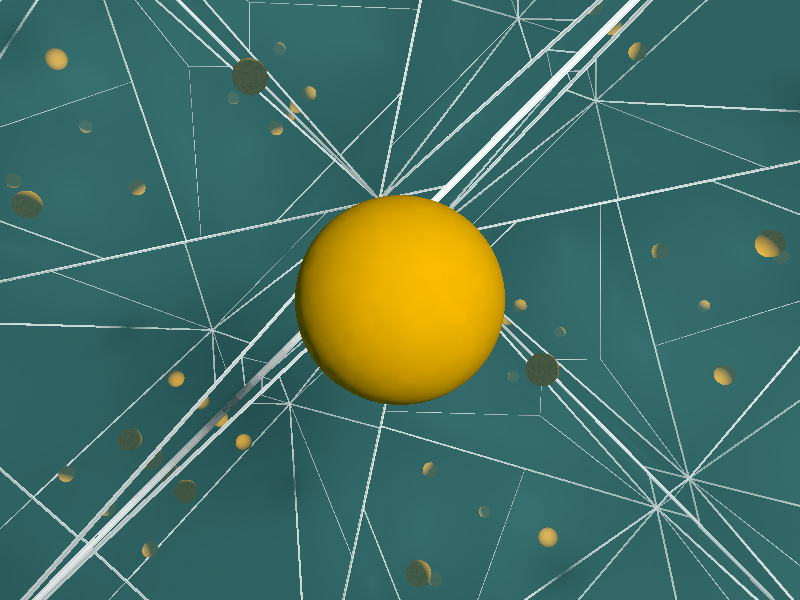
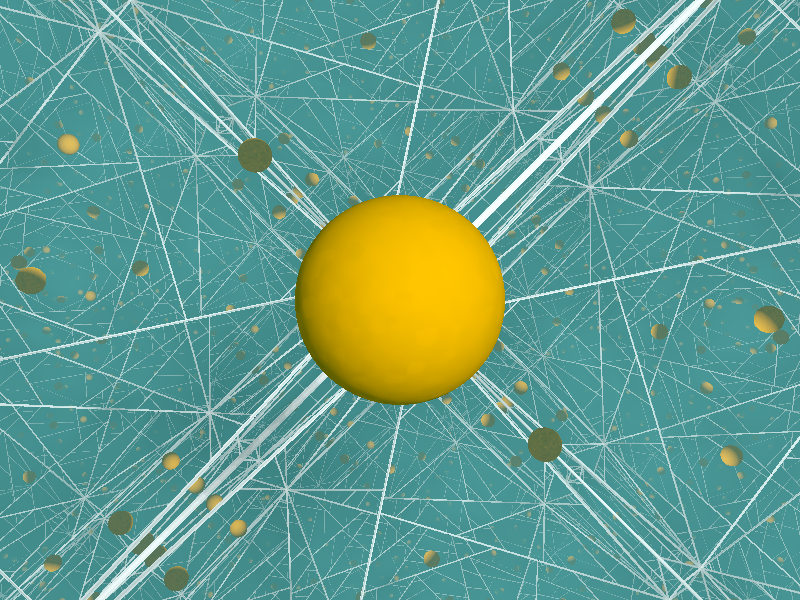
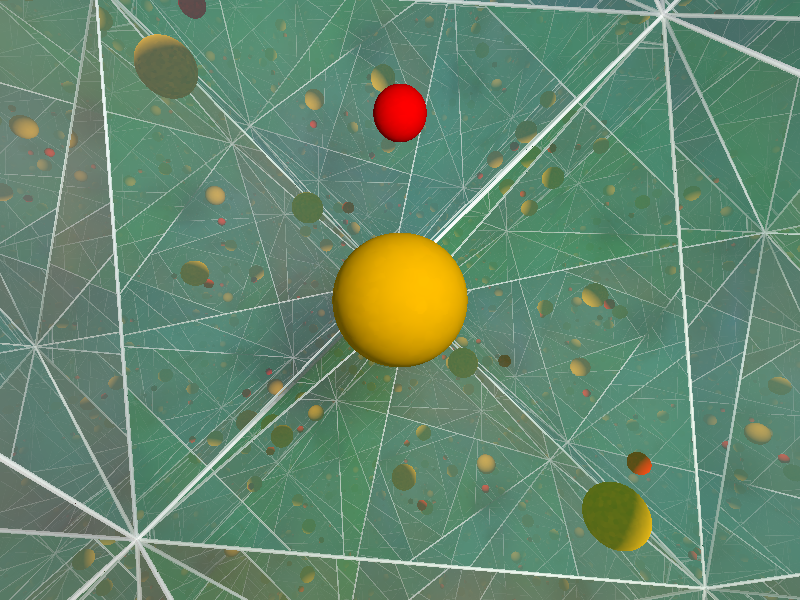
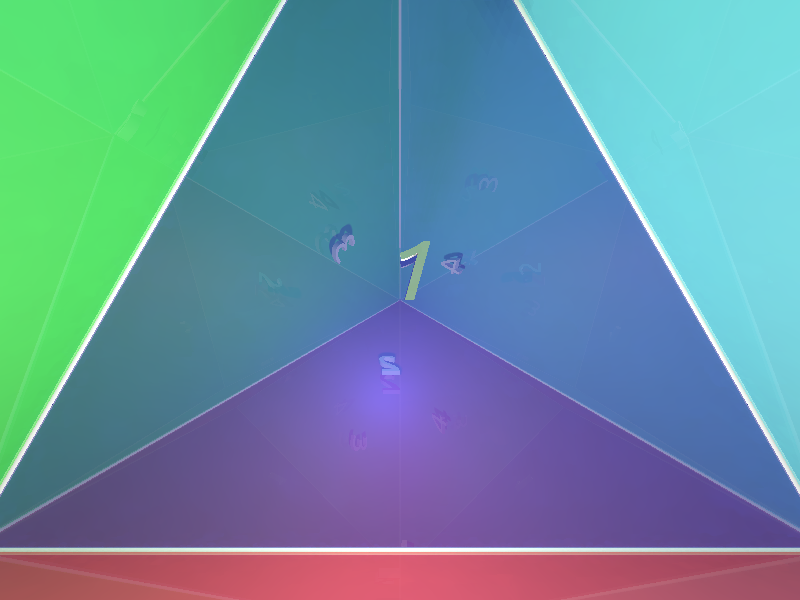
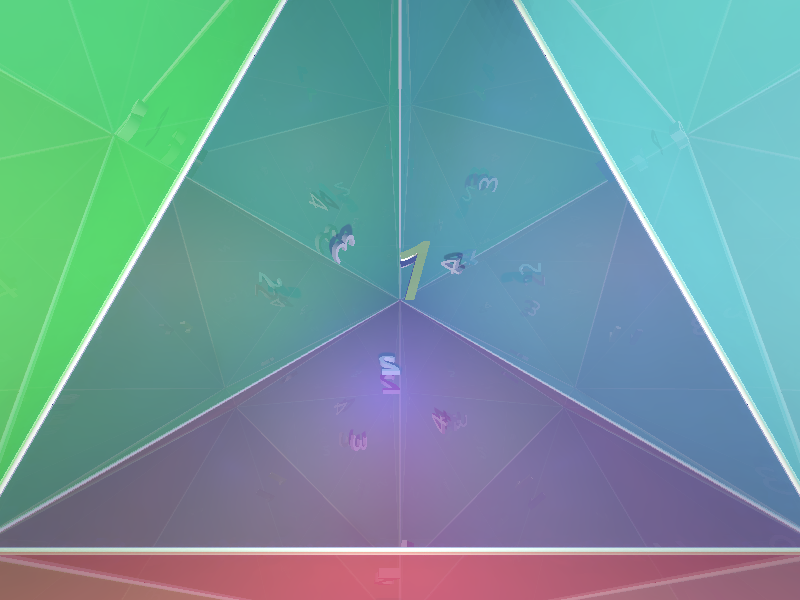
Suppose you were standing inside a regular tetrahedron $T$ whose internal face surfaces were perfect mirrors. Let's assume $T$'s height is $3{\times}$ yours, so that your eye is roughly at the centroid, and that you look perpendicular to a face:

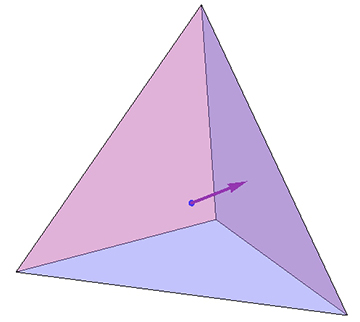
          TetraViewPoint
My question is:

Q. What do you see? Either qualitatively; or if anyone can find an image, that would be revealing.

Asking the same question for the view inside a mirrored cube is easier to visualize: the opposing parallel square-face mirrors would produce a "house of mirrors" effect (in three perpendicular directions).

                   
                    (Image from this link.)

Addendum 1 (21Nov2014). To respond to Yoav Kallus' (good) question, here is a quote from Handbook of Dynamical Systems, Volume 1, Part 1. (ed. B. Hasselblatt, A. Katok), 2010, p.194:

TetraBilliards
Addendum 2 (24Nov2014). Now that we have Ryan Budney's amazing POV-ray images, I would appreciate someone making an attempt to describe his $32$-reflection image qualitatively. I find it so complex that this may be an instance where a thousand words might be superior to one picture.

Improve this question
$\endgroup$
6
  • 5
    $\begingroup$ Since this will probably be related to billiards, do you know how billiards in T behave? $\endgroup$
    – Yoav Kallus
    Commented Nov 21, 2014 at 1:00
  • $\begingroup$ Joseph, could you tell what software you use for graphics? $\endgroup$
    – Michael
    Commented Nov 21, 2014 at 6:42
  • 5
    $\begingroup$ for related beautiful Povray drawing, see here. $\endgroup$
    – few_reps
    Commented Nov 21, 2014 at 9:03
  • 4
    $\begingroup$ It helps to build intuition to ask what it looks like one dimension lower in a triangle with angles that are not $\pi/n$. You see only part of a universal cover of a sphere with $3$ punctures, and while angles of $\pi/n$ (or sometimes $2\pi/n$) makes some of the pieces on either side of a puncture fit together, these pieces don't fit together in general. Imagine a telephone pole in the middle of a photo where the scene on the right behind the pole doesn't fit the scene on the left. Analogues happen with the reflecting tetrahedron because the dihedral angles are irrational multiples of $\pi$. $\endgroup$
    – Douglas Zare
    Commented Nov 25, 2014 at 3:03
  • 1
    $\begingroup$ Higher dimensional analogues to $\pi/n$ polygons are given by Coxeter groups: See mathoverflow.net/questions/148758/a-special-tessellation $\endgroup$
    – user25199
    Commented Nov 26, 2014 at 8:06

3 Answers 3

Reset to default
65
$\begingroup$

Here are a couple pictures. If you'd like to do more, I created this with a simple povray script. Feel free to e-mail me and I'll send it to you.

With four reflections.

enter image description here

With 8 levels of reflections.

enter image description here

One with 24 reflections.

enter image description here

And one with 32 reflections, and each mirror having a slightly different tint, a red sphere, and a slightly wider viewing angle.

enter image description here

Here is the view from inside a mirrored tetrahedron, near one of the vertices, looking towards the opposite face. Near the centre of each face is a number indicating which vertex the triangle is opposite. The sequence shows the triangles becoming more reflective and less opaque. The field of view is 120 degrees so there is a little bit of lens distortion at the fringes of the image.

enter image description here enter image description here enter image description here enter image description here enter image description here enter image description here enter image description here

And here is the link to the scripts for the tetrahedra with the numbering and without the spheres.

Improve this answer
$\endgroup$
17
  • 5
    $\begingroup$ I suppose I might as well make an mpeg movie out of this. I'll put it up on my webpage in a day or two, whenever it finishes rendering. :) $\endgroup$
    – Ryan Budney
    Commented Nov 21, 2014 at 2:08
  • 2
    $\begingroup$ Please post the pov-ray script! Also, is there some way to model a simple stick-figure person, in order to help answer the question of what it would look like if a person were inside the tetrahedron? Then we could see variation in which way is up in the reflected images; and ideally back vs. front. $\endgroup$
    – LarsH
    Commented Nov 21, 2014 at 16:58
  • 2
    $\begingroup$ Will post it tonight, once I'm back at home. I think I'll add some near-transparent numbers to the faces so that we can keep track of where the reflections are coming from. $\endgroup$
    – Ryan Budney
    Commented Nov 21, 2014 at 19:15
  • 3
    $\begingroup$ Here is the current version of the script. I'll update it as this discussion evolves: dropbox.com/s/7vtl554pjdt6mpa/orourke.pov?dl=0 $\endgroup$
    – Ryan Budney
    Commented Nov 22, 2014 at 3:06
  • 3
    $\begingroup$ I think that's just "fish eye lens effect". Notice it primarily happens out towards the edges in the last image, which has the widest field of view (90 degree viewing angle). The previous images had a 60 degree viewing angle, which results in less distortion. $\endgroup$
    – Ryan Budney
    Commented Nov 24, 2014 at 14:42
8
$\begingroup$

There is a software by Jeff Weeks, Curved Spaces which allows for flying through various such spaces. It includes a mirrored tetrahedron but with $\pi/2$ dihedral angles. One can construct own spaces though, by providing a list of 4$\times$4 matrices generating the (linearized version of the) transformation group.

Snapshot:

enter image description here

(obviously not that inspiring, you have to see it in motion to really appreciate)

Addendum

After several unsuccessful attempts to realize the Euclidean tetrahedron in the program I contacted Jeff Weeks; it turned out that unfortunately Curved Spaces cannot handle noncompact groups.

Jeff also mentioned the spherical case - regular spherical tetrahedron with dihedral angles $2\pi/5$, whose face reflections fill up the 600-cell. It occurred to me that this is a rare situation when the Euclidean case (dihedral angle $\arccos 1/3$) is the most pathological between the nearest "non-pathological" cases - spherical case mentioned above and the hyperbolic case (dihedral angle $\pi/3$). A wonderful picture of the latter may be found in the Wikipedia chapter "Order-6 tetrahedral honeycomb"

enter image description here

An additional question then - is the Euclidean case in any sense a quotient of this?

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ No, symmetry groups do not match. As you noted yourself, the 600-cell thing features 5 tetrahedrons sharing an edge (2π/5 = 72° dihedral angle) and the hyperbolic case features 6 tetrahedrons sharing an edge (π/3 = 60° dihedral angle). Whereas dihedral angle in Euclidean case is irrational. This means that: • in spherical case, five reflections about faces adjacent to the same edge compose to an isometry (orientation-reversing) of the tetrahedron; • in hyperbolic case, six reflections about faces adjacent to the same edge compose to identity transform; • nothing like this in Euclidean case. $\endgroup$
    – Incnis Mrsi
    Commented Apr 5, 2015 at 13:02
  • $\begingroup$ @IncnisMrsi You are right - I formulated it vaguely since I don't know what precisely I mean, but here is a simplified version: look at quotients of the unit circle in $\mathbb C$. Quotient by (the subgroup generated by) $e^{\frac{2\pi i}5}$ is a circle, and by $e^{\frac{2\pi i}6}$ is another one, but one might also form quotients by all intermediate elements lying in between, including irrational ones, to obtain a family of quotients (most of them pathological) connecting these two cases. I was thinking maybe one could play similar game with quotients of the hyperbolic space... $\endgroup$
    – მამუკა ჯიბლაძე
    Commented Apr 5, 2015 at 19:06
4
$\begingroup$

I don't know why this question got bumped to the front page, but since it did, I might as well answer that I made a movie of this a long time ago, showing the view inside a (Euclidean) regular tetrahedron with reflective faces (tinted red, green, blue and white, and intrinsically luminous so we can see something!), as well as what it's like to move along a straight line "through" the mirrors (equivalently, a billiard ball path, but with the view flipped at each reflection so that we seem to go through the mirror).

In comparison, this video, made by my friend Vincent Nesme¹, shows a rendering of the 600-cell as a regular tiling of the constantly curved 3-sphere by 600 (spherical!) regular tetrahedra, and moving in a straight line (great circle) in this geometry.

  1. I had previously made such a video by using gnomonic projection to map the lines into Euclidean space and feeding the result into POVray, but while this correctly projects the lines, it does not correctly project their thickness. Vincent was much more patient than I was and wrote his own raytracer for this.
Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .