One would be able to construct a Cayley table that has all $e_i$ elements of the basis of algebra $A$ where $0<i<\dim A$ such that $e_0=1$, $e_1=i$, $e_2=j$ and so on. I'm looking for an algebraic expression of the table for an algebra of dimension $N$, which enables me to find the product $e_ie_j$ without looking at the table. Does such an expression exist?
P.S. The Cayley tables for quaternions, octonions and sedonions can be found in Wikipedia.
I am not aware of a single formula, only recursive formulas involving the "shuffle basis" for Cayley-Dickson spaces. The shuffle basis is not the one commonly used by most researchers. For the shuffle basis, $e_0=1$ and $e_{2n}=(e_n,0)$ and $e_{2n+1}=(0,e_n)$. Furthermore, the product $e_pe_q=\pm e_{pq}$ where $pq$ is defined as the 'exclusive or' of the binary representations of $p$ and $q$. There are actually eight[edit: four] different Cayley-Dickson doubling products which satisfy the quaternion properties but the one most commonly used is $(a,b)(c,d)=(ac-db^*,a^*d+cb)$. For each of the eight[edit: four] Cayley-Dickson doubling products there is a distinct and well-defined 'sign function' or 'twist' $\omega(p,q)$ such that $e_pe_q=\omega(p,q)e_{pq}$. It is always true that $\omega(0,0)=\omega(p,0)=\omega(0,q)=1$ and that $\omega(p,p)=-1$ for $p>0.$ This much is true for all eight[edit: four] Cayley-Dickson doubling products. For the product mentioned above, for all positive $n$, $\omega(1,2n)=1$ and $\omega(2n,1)=-1$. Furthermore, for $0\ne p\ne q\ne 0$ $\omega(p,q)=-\omega(q,p)$ is true for all eight[edit: four] doubling products. For the given doubling product it is also true that the following are all equal for $0\ne p\ne q\ne 0$: $\omega(p,q),\omega(2p,2q),\omega(2q,2p+1),\omega(2q+1,2p),\omega(2q+1,2p+1).$ From these properties, the 'sign function' or 'twist' $\omega(p,q)$ for all higher dimensional Cayley-Dickson algebras can be recovered inductively when using the shuffle basis.
Update: "The eight Cayley-Dickson doubling products", Adv. Appl. Clifford Algebras 26 (2016) pp 529–551, doi:10.1007/s00006-015-0638-6, arXiv:1707.07318 I now find that 4 of those 8 should also be discarded: each allows zero divisors at the eight dimensional stage. The 4 remaining products are denoted in the paper as $P_0$, $P_3$ (the standard doubling product), $P_4$, $P_7$. Those four produce algebras isomorphic to the standard Cayley-Dickson algebras.
I can now provide you with an easier answer. As in the first answer we use the convention that $e_pe_q=\pm e_{p\oplus q}$ where for non-negative integers $p$ and $q$, $p\oplus q$ is the bitwise exclusive 'or' of the binary representations of $p$ and $q$. Of the eight equivalent Cayley-Dickson doubling products there is one which, to my knowledge, has not been used previously by researchers.
[edit: unfortunately, this particular doubling product cannot be classified as a Cayley-Dickson doubling product, since it produces zero divisors at the third doubling (the octonions) rather than at the fourth (the sedenions).]
$$ (a,b)(c,d)=(ac-b^*d,da^*+bc) $$
As with the other seven doubling products we have $e_0e_p=e_pe_0=e_p$ for all $p$, $e_p^2=-1$ for $p>0$ and $e_pe_q=-e_qe_p$ for $0\ne p\ne q\ne 0$. But in addition we have the wonderful formulas
$$ \text{If } 2^N\le p<q<2^{N+1} \text{ then } e_pe_q=e_{p\oplus q} $$ and $$ \text{If } 2^N\le p<2^{N+1}\le q \text{ then } e_pe_q=(-1)^{⌊q/2^N⌋}e_{p\oplus q} $$
where $⌊\bullet ⌋$ is the floor function.
For example, compute $e_{21}e_{5}$. First, $e_{21}e_{5}=-e_{5}e_{21}$. And since $4\le5<8<21$ we have $e_{21}e_{5}=-e_{5}e_{21}=-(-1)^{⌊21/4⌋}e_{5\oplus 21}=-(-1)^5e_{16}=e_{16}$.
Here is a a picture of the twist (or sign values for the basis vector multiplication table for the 1024-ions with a black pixel denoting a $+1$ and a white pixel denoting a $-1$. The table is indistinguishable to the naked eye for higher dimensional n-ions.
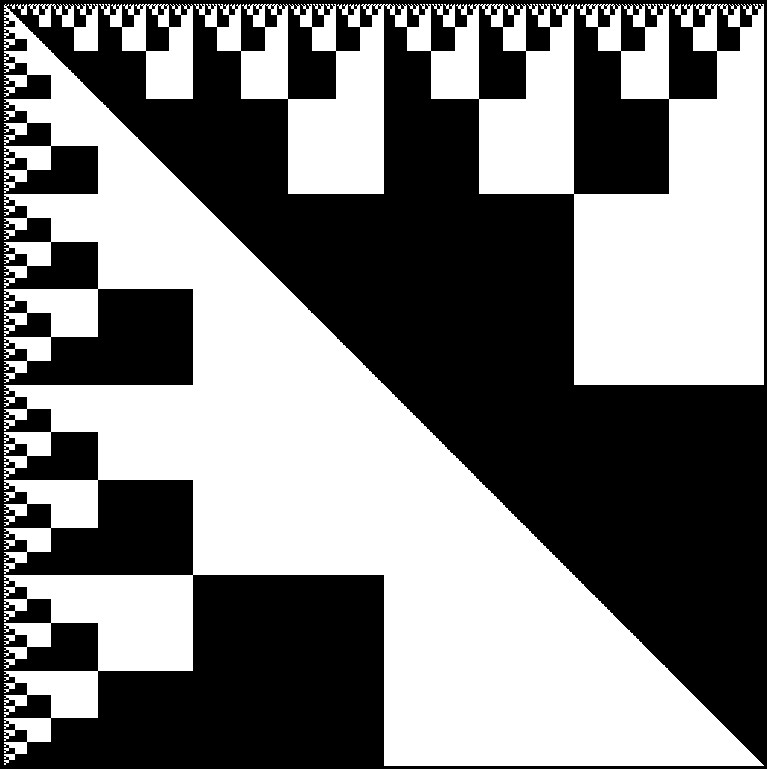
For more information see my preprint http://arxiv.org/abs/1602.02317 which is currently being refereed for publication.
